Cake Cutting

It is always possible to "fairly" divide a cake among 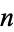 people using only vertical cuts. Furthermore, it is possible to cut and divide a cake such that each person believes that everyone has received  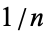of the cake according to his own measure (Steinhaus 1999, pp. 65-71). Finally, if there is some piece on which two people disagree, then there is a way of partitioning and dividing a cake such that each participant believes that he has obtained more than  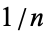of the cake according to his own measure.

There are also similar methods of dividing collections of individually indivisible objects among two or more people when cash payments are used to even up the final division (Steinhaus 1999, pp. 67-68).

Ignoring the height of the cake, the cake-cutting problem is really a question of fairly dividing a circle into 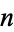 equal area pieces using cuts in its plane. One method of proving fair cake cutting to always be possible relies on the Frobenius-König theorem.

REFERENCES:

Beck, A. "Constructing a Fair Share." Amer. Math. Monthly 94, 157-162, 1987.

Brams, S. J.; Jones, M. A.; and Klamler, C. "Better Ways to Cut a Cake." Not. Amer. Math. Soc. 53, 1314-1321, 2006.

Brams, S. J. and Taylor, A. D. "An Envy-Free Cake Division Protocol." Amer. Math. Monthly 102, 9-19, 1995.

Brams, S. J. and Taylor, A. D. Fair Division: From Cake-Cutting to Dispute Resolution. New York: Cambridge University Press, 1996.

Dubbins, L. "Group Decision Devices." Amer. Math. Monthly 84, 350-356, 1997.

Dubbins, L. and Spanier, E. "How to Cut a Cake Fairly." Amer. Math. Monthly 68, 1-17, 1961.

Gale, D. "Dividing a Cake." Math. Intel. 15, 50, 1993.

Hill, T. "Determining a Fair Border." Amer. Math. Monthly 90, 438-442, 1983.

Hill, T. P. "Mathematical Devices for Getting a Fair Share." Amer. Sci. 88, 325-331, Jul.-Aug. 2000.

Jones, M. L. "A Note on a Cake Cutting Algorithm of Banach and Knaster." Amer. Math. Monthly 104, 353-355, 1997.

Knaster, B. "Sur le problème du partage pragmatique de H. Steinhaus." Ann. de la Soc. Polonaise de Math. 19, 228-230, 1946.

Rebman, K. "How to Get (At Least) a Fair Share of the Cake." In Mathematical Plums (Ed. R. Honsberger). Washington, DC: Math. Assoc. Amer., pp. 22-37, 1979.

Robertson, J. and Webb, W. Cake Cutting Algorithms: Be Fair If You Can. Wellesley, MA: A K Peters, 1998.

Steinhaus, H. "Remarques sur le partage pragmatique." Ann. de la Soc. Polonaise de Math. 19, 230-231, 1946.

Steinhaus, H. "The Problem of Fair Division." Econometrica 16, 101-104, 1948.

Steinhaus, H. "Sur la division pragmatique." Ekonometrika (Supp.) 17, 315-319, 1949.

Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, pp. 64-67, 1999.

Stromquist, W. "How to Cut a Cake Fairly." Amer. Math. Monthly 87, 640-644, 1980.