
 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 28-4-2020
Date: 5-2-2016
Date: 20-9-2020
|
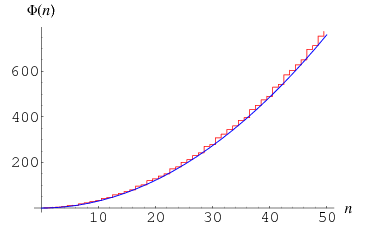
The summatory function 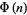 of the totient function
of the totient function 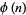 is defined by
is defined by
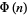 |
 |
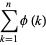 |
(1) |
 |
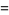 |
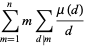 |
(2) |
 |
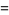 |
 |
(3) |
 |
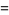 |
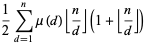 |
(4) |
(Hardy and Wright 1979, p. 268), plotted as the red curve above. The first values of 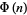 are 1, 2, 4, 6, 10, 12, 18, 22, 28, ... (OEIS A002088).
are 1, 2, 4, 6, 10, 12, 18, 22, 28, ... (OEIS A002088).
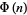 has the asymptotic series
has the asymptotic series
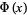 |
 |
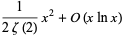 |
(5) |
 |
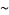 |
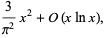 |
(6) |
where  is the Riemann zeta function (Perrot 1881; Nagell 1951, p. 131; Hardy and Wright 1979, p. 268; blue curve above). An improved asymptotic estimate due to Walfisz (1963) is given by
is the Riemann zeta function (Perrot 1881; Nagell 1951, p. 131; Hardy and Wright 1979, p. 268; blue curve above). An improved asymptotic estimate due to Walfisz (1963) is given by
![Phi(x)∼(3x^2)/(pi^2)+O[x(lnx)^(2/3)(lnlnx)^(4/3)].](https://mathworld.wolfram.com/images/equations/TotientSummatoryFunction/NumberedEquation1.gif) |
(7) |
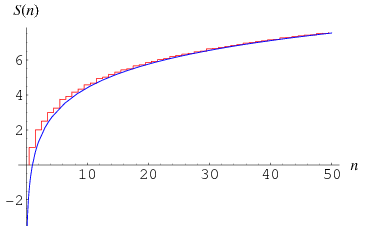
Consider the summatory function of  ,
,
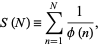 |
(8) |
plotted as the red curve above. For 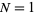 , 2, ..., the first few terms are 1, 2, 5/2, 3, 13/4, 15/4, 47/12, 25/6, ... (OEIS A028415 and A048049). The sum diverges as
, 2, ..., the first few terms are 1, 2, 5/2, 3, 13/4, 15/4, 47/12, 25/6, ... (OEIS A028415 and A048049). The sum diverges as 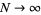 , but Landau (1900) showed that the asymptotic behavior is given by
, but Landau (1900) showed that the asymptotic behavior is given by
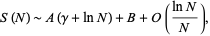 |
(9) |
where 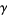 is the Euler-Mascheroni constant,
is the Euler-Mascheroni constant,
 |
 |
![sum_(k=1)^(infty)([mu(k)]^2)/(kphi(k))](https://mathworld.wolfram.com/images/equations/TotientSummatoryFunction/Inline30.gif) |
(10) |
 |
 |
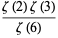 |
(11) |
 |
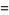 |
 |
(12) |
 |
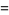 |
 |
(13) |
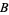 |
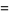 |
![sum_(k=1)^(infty)([mu(k)]^2lnk)/(kphi(k))](https://mathworld.wolfram.com/images/equations/TotientSummatoryFunction/Inline42.gif) |
(14) |
 |
 |
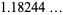 |
(15) |
(OEIS A082695), 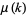 is the Möbius function,
is the Möbius function, 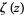 is the Riemann zeta function, and
is the Riemann zeta function, and 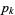 is the
is the 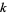 th prime (Landau 1900; Halberstam and Richert 1974, pp. 110-111; DeKoninck and Ivić 1980, pp. 1-3; Finch 2003, p. 116; Havil 2003, p. 115; Dickson 2005).
th prime (Landau 1900; Halberstam and Richert 1974, pp. 110-111; DeKoninck and Ivić 1980, pp. 1-3; Finch 2003, p. 116; Havil 2003, p. 115; Dickson 2005).
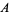 and
and  can also be written as
can also be written as
 |
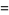 |
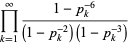 |
(16) |
 |
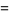 |
![product_(k=1)^(infty)[1+1/(p_k(p_k-1))]](https://mathworld.wolfram.com/images/equations/TotientSummatoryFunction/Inline57.gif) |
(17) |
and
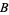 |
 |
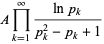 |
(18) |
 |
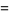 |
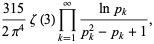 |
(19) |
respectively, making these constants similar in form to Artin's constant (Finch 2003, pp. 116-117).
The sum
 |
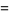 |
 |
(20) |
 |
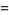 |
![zeta(2)product_(p)[1+1/(p^2(p-1))]](https://mathworld.wolfram.com/images/equations/TotientSummatoryFunction/Inline69.gif) |
(21) |
 |
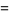 |
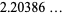 |
(22) |
(OEIS A118262) is sometimes known as the totient constant (Niklasch), where
![product_(p)[1+1/(p^2(p-1))]=1.33978...](https://mathworld.wolfram.com/images/equations/TotientSummatoryFunction/NumberedEquation4.gif) |
(23) |
(OEIS A065483) and the products are taken over the primes 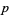 .
.
REFERENCES:
DeKoninck, J.-M. and Ivić, A. Topics in Arithmetical Functions: Asymptotic Formulae for Sums of Reciprocals of Arithmetical Functions and Related Fields. Amsterdam, Netherlands: North-Holland, 1980.
Dickson, L. E. History of the Theory of Numbers, Vol. 1: Divisibility and Primality. New York: Dover, pp. 113-158, 2005.
Finch, S. R. "Euler Totient Constants." §2.7 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 115-119, 2003.
Halberstam, H. and Richert, H.-E. Sieve Methods. New York: Academic Press, 1974.
Hardy, G. H. and Wright, E. M. "The Average Order of 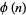 ." §18.5 in An Introduction to the Theory of Numbers, 5th ed. Oxford, England: Clarendon Press, pp. 268-269, 1979.
." §18.5 in An Introduction to the Theory of Numbers, 5th ed. Oxford, England: Clarendon Press, pp. 268-269, 1979.
Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, 2003.
Landau, E. "Über die zahlentheoretische Function 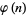 und ihre Beziehung zum Goldbachschen Satz." Nachr. Königlichen Ges. Wiss. Göttingen, Math.-Phys. Klasse, 177-186, 1900. Werke, Vol. 1 (Ed. L. Mirsky, I. J. Schoenberg, W. Schwarz, and H. Wefelscheid). Thales Verlag, pp. 106-115, 1983. Mitrinović, D. S. and Sándor, J. §I.27 in Handbook of Number Theory. Dordrecht, Netherlands: Kluwer, 1995.
und ihre Beziehung zum Goldbachschen Satz." Nachr. Königlichen Ges. Wiss. Göttingen, Math.-Phys. Klasse, 177-186, 1900. Werke, Vol. 1 (Ed. L. Mirsky, I. J. Schoenberg, W. Schwarz, and H. Wefelscheid). Thales Verlag, pp. 106-115, 1983. Mitrinović, D. S. and Sándor, J. §I.27 in Handbook of Number Theory. Dordrecht, Netherlands: Kluwer, 1995.
Nagell, T. "Relatively Prime Numbers. Euler's 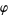 -Function." §8 in Introduction to Number Theory. New York: Wiley, pp. 23-26, 1951.
-Function." §8 in Introduction to Number Theory. New York: Wiley, pp. 23-26, 1951.
Niklasch, G. "Some Number-Theoretical Constants." https://www.gn-50uma.de/alula/essays/Moree/Moree.en.shtml.
Perrot, J. 1811. Quoted in Dickson, L. E. History of the Theory of Numbers, Vol. 1: Divisibility and Primality. New York: Dover, p. 126, 2005.
Sloane, N. J. A. Sequences A028415, A048049, A065483, A082695, A085609, A098468, and A118262 in "The On-Line Encyclopedia of Integer Sequences."
Stephens, P. J. "Prime Divisor of Second-Order Linear Recurrences, I." J. Number Th. 8, 313-332, 1976.
Walfisz, A. Ch. 5 in Weyl'sche Exponentialsummen in der neueren Zahlentheorie. Berlin: Deutscher Verlag der Wissenschaften, 1963.



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
قسم شؤون المعارف ينظم دورة عن آليات عمل الفهارس الفنية للموسوعات والكتب لملاكاته
|
|
|