


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 8-8-2019
Date: 26-8-2019
Date: 20-9-2019
|
The class of all regular sequences of particularly well-behaved functions equivalent to a given regular sequence. A distribution is sometimes also called a "generalized function" or "ideal function." As its name implies, a generalized function is a generalization of the concept of a function. For example, in physics, a baseball being hit by a bat encounters a force from the bat, as a function of time. Since the transfer of momentum from the bat is modeled as taking place at an instant, the force is not actually a function. Instead, it is a multiple of the delta function. The set of distributions contains functions (locally integrable) and Radon measures. Note that the term "distribution" is closely related to statistical distributions.
Generalized functions are defined as continuous linear functionals over a space of infinitely differentiable functions such that all continuous functions have derivatives which are themselves generalized functions. The most commonly encountered generalized function is the delta function. Vladimirov (1971) contains a nice treatment of distributions from a physicist's point of view, while the multivolume work by Gel'fand and Shilov (1964abcde) is a classic and rigorous treatment of the field. A result of Schwarz shows that distributions can't be consistently defined over the complex numbers 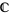 .
.
While it is possible to add distributions, it is not possible to multiply distributions when they have coinciding singular support. Despite this, it is possible to take the derivative of a distribution, to get another distribution. Consequently, they may satisfy a linear partial differential equation, in which case the distribution is called a weak solution. For example, given any locally integrable function 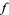 it makes sense to ask for solutions
it makes sense to ask for solutions 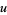 of Poisson's equation
of Poisson's equation
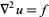 |
(1) |
by only requiring the equation to hold in the sense of distributions, that is, both sides are the same distribution. The definitions of the derivatives of a distribution  are given by
are given by
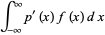 |
 |
 |
(2) |
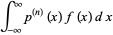 |
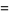 |
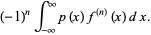 |
(3) |
Distributions also differ from functions because they are covariant, that is, they push forward. Given a smooth function 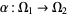 , a distribution
, a distribution 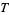 on
on 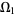 pushes forward to a distribution on
pushes forward to a distribution on 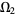 . In contrast, a real function
. In contrast, a real function 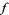 on
on  pulls back to a function on
pulls back to a function on 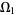 , namely
, namely 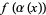 .
.
Distributions are, by definition, the dual to the smooth functions of compact support, with a particular topology. For example, the delta function  is the linear functional
is the linear functional 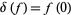 . The distribution corresponding to a function
. The distribution corresponding to a function  is
is
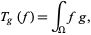 |
(4) |
and the distribution corresponding to a measure  is
is
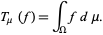 |
(5) |
The pushforward map of a distribution 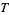 along
along 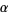 is defined by
is defined by
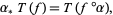 |
(6) |
and the derivative of 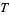 is defined by
is defined by  where
where 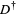 is the formal adjoint of
is the formal adjoint of 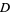 . For example, the first derivative of the delta function is given by
. For example, the first derivative of the delta function is given by
![d/(dx)[delta(f)]=-(df)/(dx)|_(x=0).](http://mathworld.wolfram.com/images/equations/GeneralizedFunction/NumberedEquation5.gif) |
(7) |
As is the case for any function space, the topology determines which linear functionals are continuous, that is, are in the dual vector space. The topology is defined by the family of seminorms,
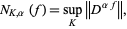 |
(8) |
where sup denotes the supremum. It agrees with the C-infty topology on compact subsets. In this topology, a sequence converges,  , iff there is a compact set
, iff there is a compact set 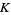 such that all
such that all 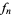 are supported in
are supported in 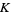 and every derivative
and every derivative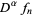 converges uniformly to
converges uniformly to 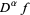 in
in 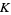 . Therefore, the constant function 1 is a distribution, because if
. Therefore, the constant function 1 is a distribution, because if 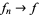 then
then
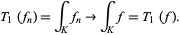 |
(9) |
REFERENCES:
Brychkov, Yu. A. and Prudnikov, A. P. Integral Transforms of Generalized Functions. New York: Gordon and Breach, 1989.
Gel'fand, I. M. and Shilov, G. E. Generalized Functions, Vol. 1: Properties and Operations. New York: Academic Press, 1964a.
Gel'fand, I. M. and Shilov, G. E. Generalized Functions, Vol. 2: Spaces of Fundamental and Generalized Functions. New York: Academic Press, 1964b.
Gel'fand, I. M. and Shilov, G. E. Generalized Functions, Vol. 3: Theory of Differential Equations. New York: Academic Press, 1964c.
Gel'fand, I. M. and Shilov, G. E. Generalized Functions, Vol. 4: Applications of Harmonic Analysis. New York: Academic Press, 1964d.
Gel'fand, I. M. and Shilov, G. E. Generalized Functions, Vol. 5: Integral Geometry and Representation Theory. New York: Academic Press, 1964e.
Kanwal, R. P. Generalized Functions: Theory and Technique, 2nd ed. Boston, MA: Birkhäuser, 1998.
Vladimirov, V. S. Equations of Mathematical Physics. New York: Dekker, 1971.



|
|
|
|
"عادة ليلية" قد تكون المفتاح للوقاية من الخرف
|
|
|
|
|
|
|
ممتص الصدمات: طريقة عمله وأهميته وأبرز علامات تلفه
|
|
|
|
|
|
|
المجمع العلمي للقرآن الكريم يقيم جلسة حوارية لطلبة جامعة الكوفة
|
|
|