


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 14-8-2019
Date: 21-5-2019
Date: 16-5-2018
|

The problem of finding the curve down which a bead placed anywhere will fall to the bottom in the same amount of time. The solution is a cycloid, a fact first discovered and published by Huygens in Horologium oscillatorium (1673). This property was also alluded to in the following passage from Moby Dick: "[The try-pot] is also a place for profound mathematical meditation. It was in the left-hand try-pot of the Pequod, with the soapstone diligently circling round me, that I was first indirectly struck by the remarkable fact, that in geometry all bodies gliding along a cycloid, my soapstone, for example, will descend from any point in precisely the same time" (Melville 1851).
Huygens also constructed the first pendulum clock with a device to ensure that the pendulum was isochronous by forcing the pendulum to swing in an arc of a cycloid. This is accomplished by placing two evolutes of inverted cycloid arcs on each side of the pendulum's point of suspension against which the pendulum is constrained to move (Wells 1991, p. 47; Gray 1997, p. 123). Unfortunately, friction along the arcs causes a greater error than that corrected by the cycloidal path (Gardner 1984).
The parametric equations of the cycloid are
 |
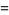 |
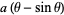 |
(1) |
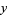 |
 |
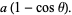 |
(2) |
To see that the cycloid satisfies the tautochrone property, consider the derivatives
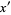 |
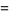 |
 |
(3) |
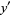 |
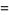 |
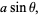 |
(4) |
and
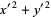 |
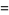 |
![a^2[(1-2costheta+cos^2theta)+sin^2theta]](http://mathworld.wolfram.com/images/equations/TautochroneProblem/Inline15.gif) |
(5) |
 |
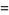 |
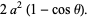 |
(6) |
Now
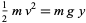 |
(7) |
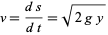 |
(8) |
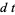 |
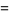 |
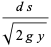 |
(9) |
 |
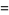 |
 |
(10) |
 |
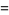 |
 |
(11) |
 |
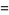 |
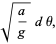 |
(12) |
so the time required to travel from the top of the cycloid to the bottom is
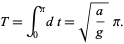 |
(13) |
However, from an intermediate point  ,
,
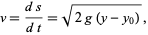 |
(14) |
so
 |
 |
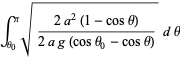 |
(15) |
 |
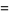 |
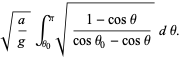 |
(16) |
To integrate, rearrange this equation using the half-angle formulas
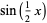 |
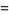 |
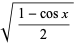 |
(17) |
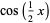 |
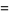 |
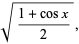 |
(18) |
with the latter rewritten in the form
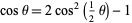 |
(19) |
to obtain
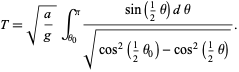 |
(20) |
Now transform variables to
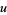 |
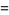 |
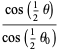 |
(21) |
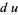 |
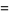 |
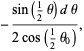 |
(22) |
so
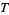 |
 |
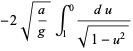 |
(23) |
 |
 |
![2sqrt(a/g)[sin^(-1)u]_0^1](http://mathworld.wolfram.com/images/equations/TautochroneProblem/Inline55.gif) |
(24) |
 |
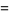 |
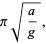 |
(25) |
and the amount of time is the same from any point.
REFERENCES:
Gardner, M. The Sixth Book of Mathematical Games from Scientific American. Chicago, IL: University of Chicago Press, pp. 129-130, 1984.
Gray, A. Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed. Boca Raton, FL: CRC Press, 1997.
Lagrange, J. L. "Sue les courbes tautochrones." Mém. de l'Acad. Roy. des Sci. et Belles-Lettres de Berlin 21, 1765. Reprinted in Oeuvres de Lagrange, tome 2, section deuxième: Mémoires extraits des recueils de l'Academie royale des sciences et Belles-Lettres de Berlin. Paris: Gauthier-Villars, pp. 317-332, 1868.
Melville, H. "The Tryworks." Ch. 96 in Moby Dick. New York: Bantam, 1981. Originally published in 1851.
Muterspaugh, J.; Driver, T.; and Dick, J. E. "The Cycloid and Tautochronism." http://php.indiana.edu/~jedick/project/intro.html
Muterspaugh, J.; Driver, T.; and Dick, J. E. "P221 Tautochrone Problem." http://php.indiana.edu/~jedick/project/project.html
Phillips, J. P. "Brachistochrone, Tautochrone, Cycloid--Apple of Discord." Math. Teacher 60, 506-508, 1967.
Wagon, S. Mathematica in Action. New York: W. H. Freeman, pp. 54-60 and 384-385, 1991.
Wells, D. The Penguin Dictionary of Curious and Interesting Geometry. London: Penguin, pp. 46-47, 1991.



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
قسم شؤون المعارف ينظم دورة عن آليات عمل الفهارس الفنية للموسوعات والكتب لملاكاته
|
|
|