The decimal number system is familiar to every reader and will be used to illustrate the meaning of the binary system. When we write the number 3814, each of the digits 3, 8, 1, and 4 conveys a meaning dependent on its position within the number: 3 refers to thousands, 8 to hundreds, 1 to tens, and 4 to units. Another way of conveying the same information is to write the number as a sum of multiples of powers of 10. Here we have

                                        3814 = 3(10³) + 8(10²) + 1(10¹) + 4(10⁰).

Having written 3814 in this way, we can easily observe the significance of 10 in our decimal system. The number 10 is referred to as the radix of the decimal system.

Any positive integer greater than 1 could serve as a radix as well as the integer 10. For instance, the number 194 written in radix 5 would read 1234, meaning that

                                        194 = 1(5³) +2 (5²) + 3(5¹) + 4(5⁰).

The same number in radix 7 would read 365, meaning 3(7²) + 6(7¹) +5(7⁰). If a radix larger than 10 were used, it would be necessary to create some new symbols, since in any system, an integer smaller than the radix should be represented by a single digit.

To make the ideas just introduced more precise, we will define a digit to be any single symbol used to represent a nonnegative integer. A number is defined to be a symbol consisting of a sequence of k + 1 digits, N = a_k …..a₂a₁a₀, where each ai is a digit. A number is related to the radix R of the number system by the equation

                                           N = a_kR^k+... + a₂R ² + a₁R + a⁰.

To convert any number N from the decimal system to the system with radix R, the following method will serve.

1. Determine the highest power, k, of R which does not exceed N.

2. Divide N by R^k. The quotient, a_k, is the first digit of N and the remainder, r₁, is used in step 3.

3. (a) If r₁ is larger than R^k-1, divide r1 by Rk-1 to obtain a quotient a^k-1, the second digit of N, and a remainder r₂ to be used in step 4.

(b) If r₁ is smaller than R^k-1, the second digit of N is 0, and r1 is used in step 4.

4. Repeat step 3 with the result of (a) or (b) and continue until all powers of R less than k have been used.

EXAMPLE 1. Convert 97 to the number system with radix 3.

Solution. 34 equils 81 and is the highest power of 3 less than 97. 97 - 81 is 1 with remainder 16. The first digit of 97 in radix 3 is therefore 1. Now since 3³ = 27 is larger than 16, the second digit is 0. Next, 16 is divided by 3² = 9 to give a quotient 1 and remainder 7. Thus the third digit is 1. Next, 7 is divided by 3 to give the quotient 2, the fourth digit, and remainder 1. Division by 3⁰ = 1 gives a quotient 1, the last digit of our number. Thus we find that the decimal number 97 should be written as 10,121 in the number system with radix 3.

To change from a given radix to the decimal system, it is necessary only to expand according to the definition in terms of powers of the radix and evaluate the resulting sum as a number in decimal notation.

EXAMPLE 2. Find the decimal equivalent of 2312 in the system of radix 4.

Solution. 2312 in radix 4 means

                                   2(4³) + 3(4²) +1(4) + 2 = 128 +48 + 4 = 2,

which is equal to 182 in decimal notation.

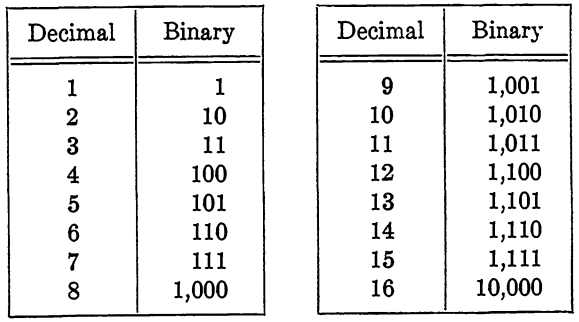
The radix 2 is of special interest since a number written in radix 2 contains only two digits, 0 and 1. It is easy to see how such a number can be represented by a sequence of switches, lights, relays, or other devices, each of which has two states corresponding to 0 and 1. The system using radix 2 is called the binary number system. The general discussion above will serve to define radix 2 but, for convenience, Table 6-1 is included to show the first sixteen numbers in both the decimal system and the binary system.

For conversion of an arbitrary number from the binary system to the decimal, or from the decimal to the binary, the methods above may be used.

                  TABLE 1-1

                         BINARY AND DECIMAL REPRESENTATIONS OF NUMBERS

مواضيع ذات صلة

Zero Polynomial

Kronecker,s Polynomial Theorem

THE ALGEBRA OF SETS-The number of elements in a set

THE ALGEBRA OF SETS-Solution of equations

THE ALGEBRA OF SETS-Conditional equations

THE ALGEBRA OF SETS-Properties of set inclusion

THE ALGEBRA OF SETS- Expanding, factoring, and simplifying

THE ALGEBRA OF SETS-Fundamental laws

THE ALGEBRA OF SETS-Venn diagrams

THE ALGEBRA OF SETS-The combination of sets

THE ALGEBRA OF SETS-Element and set

THE ALGEBRA OF SETS-Introduction