Definition Let G be a group, and let X be a set. The group G is said to act on the set X (on the left) if each element g of G determines a corresponding function θ_g: X → X from the set X to itself, where

(i) θ_gh = θ_g ◦ θ_h for all g, h ∈ G;

(ii) the function θ_e determined by the identity element e of G is the identity function of X.

Let G be a group acting on a set X. Given any element x of X, the orbit  [x]_G of x (under the group action) is defined to be the subset {θ_g(x) : g ∈ G} of X, and the stabilizer of x is defined to the the subgroup {g ∈ G : θ_g(x) =x} of the group G. Thus the orbit of an element x of X is the set consisting of all points of X to which x gets mapped under the action of elements of the group G. The stabilizer of x is the subgroup of G consisting of all elements of this group that fix the point x. The group G is said to act freely on X if θ_g(x) ≠x for all x ∈ X and g ∈ G satisfying g ≠e. Thus the group G acts freely on X if and only if the stabilizer of every element of X is the trivial subgroup of G.

Let e be the identity element of G. Then x = θ_e(x) for all x ∈ X, and therefore x ∈ [x]_G for all x ∈ X, where [x]_G = {θ_g(x) : g ∈ G}.

Let x and y be elements of G for which [x]_G ∩ [y]_G is non-empty, and let z ∈ [x]_G ∩ [y]_G. Then there exist elements h and k of G such that z = θ_h(x) = θ_k(y). Then θ_g(z) = θ_gh(x) = θ_gk(y), θ_g(x) = θ_gh−1 (z) and θ_g(y) = θ_gk−1 (z) for all g ∈ G, and therefore [x]_G = [z]_G = [y]_G. It follows from this that the group action partitions the set X into orbits, so that each element of X determines an orbit which is the unique orbit for the action of G on X to which it belongs. We denote by X/G the set of orbits for the action of G on X.

Now suppose that the group G acts on a topological space X. Then there is a surjective function q: X → X/G, where q(x) = [x]G for all x ∈ X.

This surjective function induces a quotient topology on the set of orbits: a subset U of X/G is open in this quotient topology if and only if q⁻¹ (U) is an open set in X. We define the orbit space X/G for the action of G on X to be the topological space whose underlying set is the set of orbits for the action of G on X, the topology on X/G being the quotient topology induced by the function q: X → X/G. This function q: X → X/G is then an identification map: we shall refer to it as the quotient map from X to X/G.

We shall be concerned here with situations in which a group action on a topological space gives rise to a covering map. The relevant group actions are those where the group acts freely and properly discontinuously on the topological space

Definition Let G be a group with identity element e, and let X be a topological space. The group G is said to act freely and properly discontinuously on X if each element g of G determines a corresponding continuous map θ_g: X → X, where the following conditions are satisfied:

(i) θ_gh = θ_g ◦ θ_h for all g, h ∈ G;

(ii) the continuous map θ_e determined by the identity element e of G is the identity map of X;

(iii) given any point x of X, there exists an open set U in X such that x ∈ U and θ_g(U) ∩ U = ∅ for all g ∈ G satisfying g ≠e.

Let G be a group which acts freely and properly discontinuously on a topological space X. Given any element g of G, the corresponding continuous function θ_g: X → X determined by X is a homeomorphism. Indeed it follows from conditions (i) and (ii) in the above definition that θ_g−1 ◦ θ_g and θ_g ◦ θ_g−1 are both equal to the identity map of X, and therefore θ_g: X → X is a homeomorphism with inverse θ_g−1 : X → X.

Remark The terminology `freely and properly discontinuously' is traditional, but is hardly ideal. The adverb ‘freely’ refers to the requirement that θ<sub>g</sub>(x) ≠x for all x ∈ X and for all g ∈ G satisfying g ≠ e. The adverb  ‘discontinuously’ refers to the fact that, given any point x of G, the elements of the orbit {θ<sub>g</sub>(x) : g ∈ G} of x are separated; it does not signify that the functions defining the action are in any way discontinuous or badly-behaved. The adverb ‘properly’ refers to the fact that, given any compact subset K of X, the number of elements of g for which K θ<sub>g</sub>(K) ≠θ is finite. Moreover the definitions of properly discontinuous actions in textbooks and in sources of reference are not always in agreement: some say that an action of a group G on a topological space X (where each group element determines a corresponding homeomorphism of the topological space) is properly discontinuous if, given any x ∈ X, there exists an open set U in X such that the number of elements g of the group for which g(U)∩U ≠ ∅ is finite; others say that the action is properly discontinuous if it satisfies the conditions given in the definition above for a group acting freely and properly discontinuously on the set. William Fulton, in his textbook Algebraic topology: a first course  (Springer, 1995), introduced the term `evenly' in place of `freely and properly discontinuously', but this change in terminology does not appear to have been generally adopted.

Proposition 1.8 Let G be a group acting freely and properly discontinuously on a topological space X. Then the quotient map q: X → X/G from X to the corresponding orbit space X/G is a covering map.

Proof The quotient map q: X → X/G is surjective. Let V be an open set in X. Then q⁻¹(q(V )) is the union S_g∈G_θg(V ) of the open sets θ_g(V ) as g ranges over the group G, since q⁻¹ (q(V )) is the subset of X consisting of all elements of X that belong to the orbit of some element of V . But any union of open sets in a topological space is an open set. We conclude therefore that if V is an open set in X then q(V ) is an open set in X/G.

Let x be a point of X. Then there exists an open set U in X such that x ∈ U and θ_g(U) ∩ U = ∅ for all g ∈ G satisfying g ≠ e. Now q⁻¹ (q(U)) =S_g∈Gθ_g(U). We claim that the sets θ_g(U) are disjoint. Let g and h be elements of G. Suppose that θ_g(U)∩θ_h(U) ≠ ∅. Then θ_h⁻¹ (θ_g(U)∩θ_h(U)) ≠ ∅. But θ_h⁻¹ : X → X is a bijection, and therefore θ_h⁻¹ (θ_g(U) ∩ θ_h(U)) = θh⁻¹ (θ_g(U)) ∩ θ_h⁻¹ (θ_h(U)) = θ_h⁻¹_g(U) ∩ U,  and therefore θ_h⁻¹_g(U) ∩ U ≠∅. It follows that h⁻¹_g = e, where e denotes the identity element of G, and therefore g = h. Thus if g and h are elements of g, and if g ≠ h, then θ_g(U) ∩ θ_h(U) = ∅. We conclude therefore that the preimage q⁻¹(q(U)) of q(U) is the disjoint union of the sets θ_g(U) as g ranges over the group G. Moreover each these sets θ_g(U) is an open set in X.

Now U ∩ [u]G = {u} for all u ∈ U, since [u]G = {θ_g(u) : g ∈ G} and U ∩ θ_g(U) = ∅ when g ≠e. Thus if u and v are elements of U, and if q(u) =q(v) then [u]G = [v]G and therefore u = v. It follows that the restriction q|U: U → X/G of the quotient map q to U is injective, and therefore q maps U bijectively onto q(U). But q maps open sets onto open sets, and any continuous bijection that maps open sets onto open sets is a homeomorphism.

We conclude therefore that the restriction of q: X → X/G to the open set U maps U homeomorphically onto q(U). Moreover, given any element g of G,

the quotient map q satisfies q = q ◦ θ_g⁻¹ , and the homeomorphism θ_g⁻¹ maps θ_g(U) homeomorphically onto U. It follows that the quotient map q maps θ_g(U) homeomorphically onto q(U) for all g ∈ U. We conclude therefore that q(U) is an evenly covered open set in X/G whose preimage q⁻¹ (q(U)) is the disjoint union of the open sets θ_g(U) as g ranges over the group G. It follows that the quotient map q: X → X/G is a covering map, as required.

Theorem 1.9 Let G be a group acting freely and properly discontinuously on a path-connected topological space X, let q: X → X/G be the quotient map from X to the orbit space X/G, and let x₀ be a point of X. Then there exists a surjective homomorphism λ: π₁(X/G, q(x₀)) → G with the property that γ˜(1) = θ_λ([γ])(x₀) for any loop γ in X/G based at q(x₀), where γ˜ denotes the unique path in X for which γ˜(0) = x0 and q ◦ γ˜ = γ. The kernel of this homomorphism is the subgroup q_#(π₁(X, x₀)) of π₁(X/G, q(x₀)).

Proof Let γ: [0, 1] → X/G be a loop in the orbit space with γ(0) = γ(1) =q(x₀). It follows from the Path Lifting Theorem for covering maps (Path Lifting Theorem) that there exists a unique path γ˜: [0, 1] → X for which γ˜ (0) = x₀ and q ◦ γ˜ = γ. Now γ˜ (0) and γ˜ (1) must belong to the same orbit, since q(γ˜ (0)) = γ(0) = γ(1) = q(γ˜(1)). Therefore there exists some element g of G such that γ˜(1) = θ_g(x₀). This element g is uniquely determined, since the group G acts freely on X. Moreover the value of g is determined by the based homotopy class [γ] of  in π₁ (X,q(x₀)). Indeed it follows from Proposition 1.1 that if σ is a loop in X/G based at q(x0), if σ˜ is the lift of σ starting at x₀ (so that q ◦σ˜ = σ and σ˜ (0) = x₀), and if [γ] = [σ] in π₁(X/G, q(x₀)) (so that γ ≃ σ rel {0, 1}), then γ˜ (1) = σ˜(1). We conclude therefore that there exists a well-defined function

                             λ: π₁(X/G, q(x₀)) → G,

which is characterized by the property that γ˜ (1) = θ_λ([γ])(x₀) for any loop γ in X/G based at q(x0), where γ˜ denotes the unique path in X for which γ˜(0) = x0 and q ◦ γ˜ = γ.

Now let α: [0, 1] → X/G and β: [0, 1] → X/G be loops in X/G based at x₀, and let α˜: [0, 1] → X and β˜: [0, 1] → X be the lifts of α and β respectively starting at x0, so that                            q ◦ α˜ = α, q ◦ β˜ = β and α˜(0) = β˜(0) = x₀. Thenα˜(1) = θ_λ([α])(x₀) and β˜(1) = θ_λ([β])(x₀). Then the path θ_λ([α]) ◦ β˜ is also a lift of the loop β, and is the unique lift of β starting at α˜ (1). Let α.β be the concatenation of the loops α and β, where

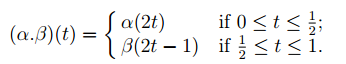
Then the unique lift of α.β to X starting at x₀ is the path σ: [0, 1] → X, where

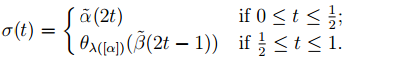
It follows that

θ_{λ([α][β])(x0)} = θ_λ([α.β])(x₀) = σ(1) = θ_λ([α])(β˜(1))

                = θ_{λ([α])(θλ([β])}(x₀)) = θ_{λ([α])λ([β])}(x₀)

and therefore λ([α][β]) = λ([α])λ([β]). Therefore the function

      λ: π₁(X/G, q(x₀)) → G

is a homomorphism.

Let g ∈ G. Then there exists a path α in X from x₀ to θ_g(x₀), since the space X is path-connected. Then q ◦ α is a loop in X/G based at q(x₀), and g = λ([q ◦ α]). This shows that the homomorphism λ is surjective.

Let γ: [0, 1] → X/G be a loop in X/G based at q(x₀). Suppose that [γ] ∈ ker λ. Then γ˜(1) = θ_e(x₀) = x₀, and therefore γ˜ is a loop in X based at x₀. Moreover [γ] = q_#[γ˜], and therefore [γ] ∈ q_#(π₁(X, x₀)). On the other hand, if [γ] ∈ q_#(π₁(X, x₀)) then γ = q ◦ γ˜ for some loop γ˜ in X based at x₀ (see Corollary 1.3). But then x₀ = γ˜ (1) = θ_λ([γ])(x₀), and therefore λ([γ]) = e, where e is the identity element of G. Thus ker λ = q_#(π₁(X, x₀)),  as required.

Corollary 1.10 Let G be a group acting freely and properly discontinuously on a path-connected topological space X, let q: X → X/G be the quotient map from X to the orbit space X/G, and let x0 be a point of X. Then q_#(π₁(X, x₀)) is a normal subgroup of the fundamental group π₁(X/G, q(x₀))  of the orbit space, and

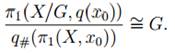
Proof The subgroup q_#(π₁(X, x₀)) is the kernel of the homomorphism

                     λ: π₁(X/G, q(x₀)) → G

described in the statement of Theorem 1.9. It is therefore a normal subgroup of π₁(X/G, q(x₀)), since the kernel of any homomorphism is a normal subgroup. The homomorphism λ is surjective, and the image of any group homomorphism is isomorphism of the quotient of its domain by its kernel.

The result follows.

Corollary 1.11 Let G be a group acting freely and properly discontinuously on a simply-connected topological space X, let q: X → X/G be the quotient map from X to the orbit space X/G, and let x₀ be a point of X. Then

               π₁(X/G, q(x₀)) ≅ G.

Proof This is a special case of Corollary 1.10.

Example The group Z of integers under addition acts freely and properly discontinuously on the real line R. Indeed each integer n determines a corresponding homeomorphism θ_n: R → R, where θ_n(x) = x + n for all x ∈ R.

Moreover θ_m ◦ θ_n = θ_m+n for all m, n ∈ Z, and θ₀ is the identity map of R. If U = (−1/2, 1/2) then θ_n(U) ∩ U = ∅ for all non-zero integers n. The real line R is simply-connected. It follows from Corollary 1.11 that π₁(R/Z, b) ≅ Z for any point b of R/Z.

Now the orbit space R/Z is homeomorphic to a circle. Indeed let q: R →R/Z be the quotient map. Then the surjective function p: R → S¹ which sends t ∈ R to (cos 2πt,sin 2πt) induces a continuous map h: R/Z → S¹ defined on the orbit space which satisfies h ◦ q = p, since the quotient map q is an identification map. Moreover real numbers t₁ and t₂ satisfy p(t₁) = p(t₂)  if and only if q(t₁) = q(t₂). It follows that the induced map h: R/Z → S¹ is a bijection. This map also maps open sets to open sets, for if W is any open set in the orbit space R/Z then q⁻¹(W) is an open set in R, and therefore p(q⁻¹ (W)) is an open set in S¹, since the covering map p: R → S¹ maps open sets to open sets. But p(q⁻¹ (W)) = h(W) for all open sets W in R/Z. Thus the continuous bijection h: R/Z → S¹ maps open sets to open sets, and is therefore a homeomorphism. Thus Corollary 1.11 generalizes the result of Theorem (π₁(S¹, b) ≅ Z for any b ∈ S¹).

Example The group Zⁿ of ordered n-tuples of integers under addition acts freely and properly discontinuously on Rⁿ, where

   θ_{(m1,m2,...,mn)}(x₁, x₂, . . . , x_n) = (x₁ + m₁, x₂ + m₂, . . . , x_n + m_n)

for all (m₁, m₂, . . . , m_n) ∈ Z_n and (x₁, x₂, . . . , x_n) ∈ Rⁿ . The orbit space Rⁿ/Zⁿ is an n-dimensional torus, homeomorphic to the product of n circles. It follows from Corollary 1.11 that the fundamental group of this n-dimensional torus is isomorphic to the group Zⁿ.

Example Let C₂ be the cyclic group of order 2. Then C₂ = {e, a} where e is the identity element, a ≠e, a² = e. Then the group C₂ acts freely and properly discontinuously on the n-dimensional sphere Sⁿ for each nonnegative integer n. We represent Sⁿ as the unit sphere centred on the origin in Rⁿ⁺¹. The homeomorphism θ_e determined by the identity element e of C₂ is the identity map of Sⁿ; the homeomorphism θ_a determined by the element a of C₂ is the antipodal map that sends each point x of Sⁿ to −x.

The orbit space Sⁿ/C₂ is homeomorphic to real projective n-dimensional space RPⁿ. The n-dimensional sphere is simply-connected if n > 1. It follows from Corollary 1.11 that the fundamental group of RPⁿ is isomorphic to the cyclic group C₂ when n > 1.

Note that S⁰ is a pair of points, and RP⁰ is a single point. Also S¹is a circle (which is not simply-connected) and RP¹ is homeomorphic to a circle.  Moreover, for any b ∈ S¹ , the homomorphism q_#: π₁(S¹, b) → π₁(RP¹, q(b))  corresponds to the homomorphism from Z to Z that sends each integer n to 2n. This is consistent with the conclusions of Corollary 1.10 in this example.

Example Given a pair (m, n) of integers, let θ_m,n:R²→R² be the homeomorphism of the plane R² defined such that θ_m,n(x, y) = (x+m,(−1)^my +n)

for all (x, y) ∈ R². Let (m₁, n₁) and (m₂, n₂) be ordered pairs of integers.

Then θ_m1,n1 ◦θ_m2,n2 = θ_{m1+m2,n1+(−1)m1n2} . Let Γ be the group whose elements are represented as ordered pairs of integers, where the group operation # on Γ is defined such that

        (m₁, n₁)#(m₂, n₂) = (m₁ + m₂, n₁ + (−1)m₁ n₂)

for all (m₁, n₁),(m₂, n₂) ∈ Γ. The group Γ is non-Abelian, and its identity element is (0, 0). This group acts on the plane R²: given (m, n) ∈ Γ thecorresponding symmetry θ_m,n is a translation if m is even, and is a glide reflection if m is odd. Given a pair (m, n) of integers, the corresponding homeomorphism θm,n maps an open disk about the point (x, y) onto an open

disk of the same radius about the point θ(m,n)(x, y). It follows that if D is the open disk of radius ½ about the point (x, y), and if D ∩ θ_m,n(D) is nonempty, then (m, n) = (0, 0). Thus the group Γ maps freely and properly discontinuously on the plane R².

The orbit space R²/Γ is homeomorphic to a Klein bottle. To see this,  note each orbit intersects the closed unit square S, where S = [0, 1] × [0, 1].

If 0 < x < 1 and 0 < y < 1 then the orbit of (x, y) intersects the square S in one point, namely the point (x, y). If 0 < x < 1, then the orbit of (x, 0)  intersects the square in two points (x, 0) and (x, 1). If 0 < y < 1 then the orbit of (0, y) intersects the square S in the two points (0, y) and (1, 1 − y).

(Note that (1, 1−y) = θ_1,1(0, y).) And the orbit of any corner of the square S intersects the square in the four corners of the square. The restriction q|S of the quotient map q: R

2 → R²/Γ to the square S is a continuous surjection defined on the square: one can readily verify that it is an identification map.

It follows that the orbit space R²/Γ is homeomorphic to the identification space obtained from the closed square S by identifying together the points  (x, 0) and (x, 1) where the real number x satisfies 0 < x < 1, identifying together the points (0, y) and (1, 1 − y) where the real number y satisfies 0 < y < 1, and identifying together the four corners of the square: this identification space is the Klein bottle.