


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 26-12-2021
Date: 14-2-2017
Date: 30-12-2021
|
Let 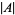 denote the cardinal number of set
denote the cardinal number of set  , then it follows immediately that
, then it follows immediately that
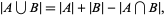 |
(1) |
where  denotes union, and
denotes union, and 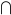 denotes intersection. The more general statement
denotes intersection. The more general statement
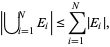 |
(2) |
also holds, and is known as Boole's inequality or one of the Bonferroni inequalities.
This formula can be generalized in the following beautiful manner. Let 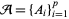 be a p-system of
be a p-system of 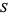 consisting of sets
consisting of sets 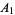 , ...,
, ..., 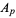 , then
, then
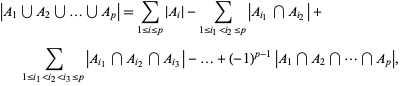 |
(3) |
where the sums are taken over k-subsets of  . This formula holds for infinite sets
. This formula holds for infinite sets  as well as finite sets (Comtet 1974, p. 177).
as well as finite sets (Comtet 1974, p. 177).
The principle of inclusion-exclusion was used by Nicholas Bernoulli to solve the recontres problem of finding the number of derangements (Bhatnagar 1995, p. 8).
For example, for the three subsets 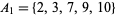 ,
,  , and
, and 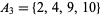 of
of 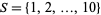 , the following table summarizes the terms appearing the sum.
, the following table summarizes the terms appearing the sum.
| # | term | set | length |
| 1 | 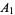 |
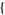 2, 3, 7, 9, 10 2, 3, 7, 9, 10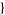 |
5 |
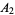 |
 1, 2, 3, 9 1, 2, 3, 9 |
4 | |
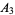 |
 2, 4, 9, 10 2, 4, 9, 10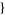 |
4 | |
| 2 | 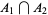 |
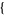 2, 3, 9 2, 3, 9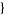 |
3 |
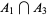 |
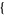 2, 9, 10 2, 9, 10 |
3 | |
 |
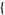 2, 9 2, 9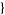 |
2 | |
| 3 |  |
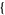 2, 9 2, 9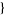 |
2 |
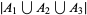 is therefore equal to
is therefore equal to 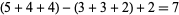 , corresponding to the seven elements
, corresponding to the seven elements 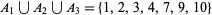 .
.
REFERENCES:
Andrews, G. E. Number Theory. Philadelphia, PA: Saunders, pp. 139-140, 1971.
Andrews, G. E. q-Series: Their Development and Application in Analysis, Number Theory, Combinatorics, Physics, and Computer Algebra. Providence, RI: Amer. Math. Soc., p. 60, 1986.
Bhatnagar, G. Inverse Relations, Generalized Bibasic Series, and Their U(n) Extensions. Ph.D. thesis. Ohio State University, 1995.
Comtet, L. Advanced Combinatorics: The Art of Finite and Infinite Expansions, rev. enl. ed. Dordrecht, Netherlands: Reidel, pp. 176-177, 1974.
da Silva. "Proprietades geraes." J. de l'Ecole Polytechnique, cah. 30.
de Quesada, C. A. "Daniel Augusto da Silva e la theoria delle congruenze binomie." Ann. Sci. Acad. Polytech. Porto, Coīmbra 4, 166-192, 1909.
Dohmen, K. Improved Bonferroni Inequalities with Applications: Inequalities and Identities of Inclusion-Exclusion Type. Berlin: Springer-Verlag, 2003.
Havil, J. Gamma: Exploring Euler's Constant. Princeton, NJ: Princeton University Press, p. 66, 2003.
Knuth, D. E. The Art of Computer Programming, Vol. 1: Fundamental Algorithms, 3rd ed. Reading, MA: Addison-Wesley, pp. 178-179, 1997.
Sylvester, J. "Note sur la théorème de Legendre." Comptes Rendus Acad. Sci. Paris 96, 463-465, 1883.



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
قسم شؤون المعارف ينظم دورة عن آليات عمل الفهارس الفنية للموسوعات والكتب لملاكاته
|
|
|