


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 6-12-2020
Date: 21-3-2020
Date: 26-4-2020
|
In order to find integers  and
and 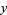 such that
such that
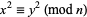 |
(1) |
(a modified form of Fermat's factorization method), in which case there is a 50% chance that 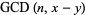 is a factor of
is a factor of 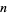 , choose a random integer
, choose a random integer 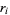 , compute
, compute
 |
(2) |
and try to factor 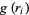 . If
. If 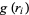 is not easily factorable (up to some small trial divisor
is not easily factorable (up to some small trial divisor 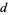 ), try another
), try another 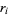 . In practice, the trial
. In practice, the trial 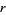 s are usually taken to be
s are usually taken to be 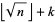 , with
, with 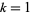 , 2, ..., which allows the quadratic sieve factorization method to be used. Continue finding and factoring
, 2, ..., which allows the quadratic sieve factorization method to be used. Continue finding and factoring 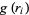 s until
s until 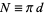 are found, where
are found, where 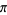 is the prime counting function. Now for each
is the prime counting function. Now for each 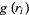 , write
, write
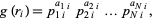 |
(3) |
and form the exponent vector
![v(r_i)=[a_(1i); a_(2i); |; a_(Ni)].](https://mathworld.wolfram.com/images/equations/DixonsFactorizationMethod/NumberedEquation4.gif) |
(4) |
Now, if 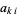 are even for any
are even for any 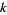 , then
, then 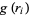 is a square number and we have found a solution to (◇). If not, look for a linear combination
is a square number and we have found a solution to (◇). If not, look for a linear combination  such that the elements are all even, i.e.,
such that the elements are all even, i.e.,
![c_1[a_(11); a_(21); |; a_(N1)]+c_2[a_(12); a_(22); |; a_(N2)]+...+c_N[a_(1N); a_(2N); |; a_(NN)]=[0; 0; |; 0] (mod 2)](https://mathworld.wolfram.com/images/equations/DixonsFactorizationMethod/NumberedEquation5.gif) |
(5) |
![[a_(11) a_(12) ... a_(1N); a_(21) a_(22) ... a_(2N); | | ... |; a_(N1) a_(N2) ... a_(NN)][c_1; c_2; |; c_N]=[0; 0; |; 0] (mod 2).](https://mathworld.wolfram.com/images/equations/DixonsFactorizationMethod/NumberedEquation6.gif) |
(6) |
Since this must be solved only mod 2, the problem can be simplified by replacing the 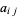 s with
s with
 |
(7) |
Gaussian elimination can then be used to solve
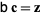 |
(8) |
for  , where
, where  is a vector equal to
is a vector equal to 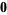 (mod 2). Once
(mod 2). Once 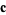 is known, then we have
is known, then we have
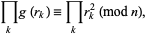 |
(9) |
where the products are taken over all 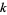 for which
for which 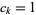 . Both sides are perfect squares, so we have a 50% chance that this yields a nontrivial factor of
. Both sides are perfect squares, so we have a 50% chance that this yields a nontrivial factor of 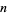 . If it does not, then we proceed to a different
. If it does not, then we proceed to a different 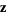 and repeat the procedure. There is no guarantee that this method will yield a factor, but in practice it produces factors faster than any method using trial divisors. It is especially amenable to parallel processing, since each processor can work on a different value of
and repeat the procedure. There is no guarantee that this method will yield a factor, but in practice it produces factors faster than any method using trial divisors. It is especially amenable to parallel processing, since each processor can work on a different value of 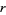
REFERENCES:
Bressoud, D. M. Factorization and Primality Testing. New York: Springer-Verlag, pp. 102-104, 1989.
Dixon, J. D. "Asymptotically Fast Factorization of Integers." Math. Comput. 36, 255-260, 1981.
Lenstra, A. K. and Lenstra, H. W. Jr. "Algorithms in Number Theory." In Handbook of Theoretical Computer Science, Volume A: Algorithms and Complexity (Ed. J. van Leeuwen). New York: Elsevier, pp. 673-715, 1990.
Pomerance, C. "A Tale of Two Sieves." Not. Amer. Math. Soc. 43, 1473-1485, 1996.



|
|
|
|
تفوقت في الاختبار على الجميع.. فاكهة "خارقة" في عالم التغذية
|
|
|
|
|
|
|
أمين عام أوبك: النفط الخام والغاز الطبيعي "هبة من الله"
|
|
|
|
|
|
|
المجمع العلمي ينظّم ندوة حوارية حول مفهوم العولمة الرقمية في بابل
|
|
|