Expressions of the form

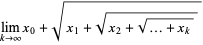(1)

are called nested radicals. Herschfeld (1935) proved that a nested radical of real nonnegative terms converges iff  is bounded. He also extended this result to arbitrary powers (which include continued square roots and continued fractions as well), a result is known as Herschfeld's convergence theorem.

Nested radicals appear in the computation of pi,

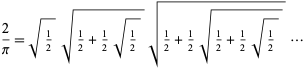(2)

(Vieta 1593; Wells 1986, p. 50; Beckmann 1989, p. 95), in trigonometrical values of cosine and sine for arguments of the form , e.g.,

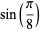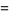			(3)
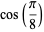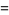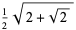			(4)
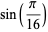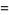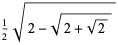			(5)
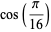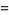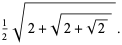			(6)

Nest radicals also appear in the computation of the golden ratio

(7)

and plastic constant

{1, +, RadicalBox[{1, +, RadicalBox[{1, +, ...}, 3]}, 3]}, 3]. " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation4.gif" style="height:44px; width:164px" />

(8)

Both of these are special cases of

{a, +, RadicalBox[{a, +, ...}, n]}, n], " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation5.gif" style="height:26px; width:120px" />

(9)

which can be exponentiated to give

{a, +, RadicalBox[{a, +, ...}, n]}, n], " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation6.gif" style="height:26px; width:148px" />

(10)

so solutions are

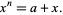(11)

In particular, for , this gives

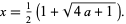(12)

The silver constant is related to the nested radical expression

{7, +, 7, RadicalBox[{7, +, ...}, 3]}, 3]. " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation9.gif" style="height:26px; width:106px" />

(13)

There are a number of general formula for nested radicals (Wong and McGuffin). For example,

{{(, {1, -, q}, )}, {x, ^, n}, +, q, {x, ^, {(, {n, -, 1}, )}}, RadicalBox[{{(, {1, -, q}, )}, {x, ^, n}, +, q, {x, ^, {(, {n, -, 1}, )}}, RadicalBox[..., n]}, n]}, n] " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation10.gif" style="height:44px; width:302px" />

(14)

which gives as special cases

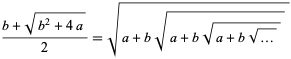(15)

(, , ),

{{x, ^, {(, {n, -, 1}, )}}, RadicalBox[{{x, ^, {(, {n, -, 1}, )}}, RadicalBox[{{x, ^, {(, {n, -, 1}, )}}, RadicalBox[..., n]}, n]}, n]}, n] " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation12.gif" style="height:59px; width:193px" />

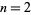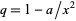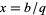(16)

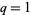(), and

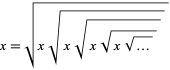(17)

(). Equation (14) also gives rise to

{{q, ^, {(, {{(, {{n, ^, {(, {k, +, 1}, )}}, -, n}, )}, /, {(, {n, -, 1}, )}}, )}}, {(, {1, -, q}, )}, {x, ^, {(, {n, ^, {(, {j, +, 1}, )}}, )}}, +, ...}, n] ...+RadicalBox[{{q, ^, {(, {{(, {{n, ^, {(, {k, +, 2}, )}}, -, n}, )}, /, {(, {n, -, 1}, )}}, )}}, {(, {1, -, q}, )}, {x, ^, {(, {n, ^, {(, {j, +, 2}, )}}, )}}, +, RadicalBox[..., n]}, n]^_, " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation14.gif" style="height:101px; width:299px" />

(18)

which gives the special case for 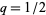, , 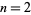, 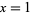and ,

(19)

Equation (◇) can be generalized to

{x, RadicalBox[{x, RadicalBox[{x, ...}, n]}, n]}, n] " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation16.gif" style="height:44px; width:153px" />

(20)

for integers 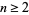, which follows from

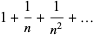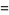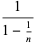			(21)
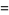			(22)
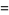			(23)
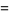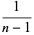			(24)
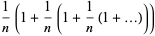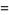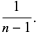			(25)

In particular, taking 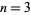 gives

{x, RadicalBox[{x, RadicalBox[{x, ...}, 3]}, 3]}, 3]. " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation17.gif" style="height:44px; width:142px" />

(26)

(J. R. Fielding, pers. comm., Oct. 8, 2002).

Ramanujan discovered

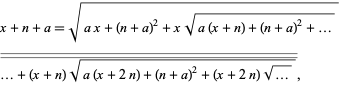(27)

which gives the special cases

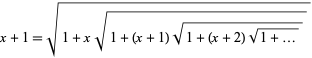(28)

for 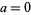,  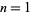(Ramanujan 1911; Ramanujan 2000, p. 323; Pickover 2002, p. 310), and

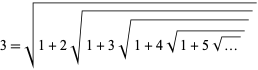(29)

for 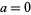, , and . The justification of this process in general (and in the particular example of 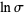, where 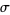is Somos's quadratic recurrence constant) is given by Vijayaraghavan (in Ramanujan 2000, p. 348).

An amusing nested radical follows rewriting the series for e

(30)

as

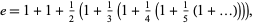(31)

so

{x, RadicalBox[{x, RadicalBox[{x, ...}, 5]}, 4]}, 3]) " src="http://mathworld.wolfram.com/images/equations/NestedRadical/NumberedEquation23.gif" style="height:59px; width:168px" />

(32)

(J. R. Fielding, pers. comm., May 15, 2002).

REFERENCES:

Beckmann, P. A History of Pi, 3rd ed. New York: Dorset Press, 1989.

Berndt, B. C. Ramanujan's Notebooks, Part IV. New York: Springer-Verlag, pp. 14-20, 1994.

Borwein, J. M. and de Barra, G. "Nested Radicals." Amer. Math. Monthly 98, 735-739, 1991.

Herschfeld, A. "On Infinite Radicals." Amer. Math. Monthly 42, 419-429, 1935.

Jeffrey, D. J. and Rich, A. D. In Computer Algebra Systems (Ed. M. J. Wester). Chichester, England: Wiley, 1999.

Landau, S. "A Note on 'Zippel Denesting.' " J. Symb. Comput. 13, 31-45, 1992a.

Landau, S. "Simplification of Nested Radicals." SIAM J. Comput. 21, 85-110, 1992b.

Landau, S. "How to Tangle with a Nested Radical." Math. Intell. 16, 49-55, 1994.

Landau, S. "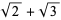: Four Different Views." Math. Intell. 20, 55-60, 1998.

Pólya, G. and Szegö, G. Problems and Theorems in Analysis, Vol. 1. New York: Springer-Verlag, 1997.

Ramanujan, S. Question No. 298. J. Indian Math. Soc. 1911.

Ramanujan, S. Collected Papers of Srinivasa Ramanujan (Ed. G. H. Hardy, P. V. S. Aiyar, and B. M. Wilson). Providence, RI: Amer. Math. Soc., p. 327, 2000.

Sizer, W. S. "Continued Roots." Math. Mag. 59, 23-27, 1986.

Vieta, F. Uriorum de rebus mathematicis responsorum. Liber VII. 1593. Reprinted in New York: Georg Olms, pp. 398-400 and 436-446, 1970.

Wells, D. The Penguin Dictionary of Curious and Interesting Numbers. Middlesex, England: Penguin Books, 1986.

Wong, B. and McGuffin, M. "The Museum of Infinite Nested Radicals." http://www.dgp.toronto.edu/~mjmcguff/math/nestedRadicals.html.