Consider two spherical conductors connected by a wire. Suppose that electric charge flows periodically back and forth between the spheres. Let q be the charge on one of the conductors. The system has zero net charge, so the charge on the other conductor is -q. Let

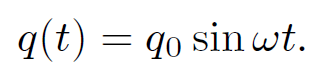 (1.1)

We expect the oscillating current flowing in the wire connecting the two spheres to generate electromagnetic radiation. Let us consider the simple case where the length of the wire is small compared to the wavelength of the emitted radiation. If this is the case then the current I flowing between the conductors has the same phase along the whole length of the wire. It follows that

 (1.2)

where I₀ = 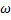q₀. This type of antenna is called a Hertzian dipole, after the German physicist Heinrich Hertz. The magnetic vector potential generated by a current distribution j(r) is given by the well-known formula

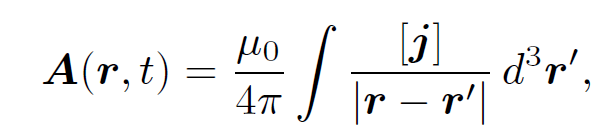 (1.3)

where

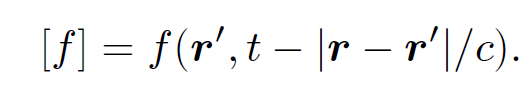 (1.4)

Suppose that the wire is aligned along the z-axis and extends from z = -l/2 to z = l/2. For a wire of negligible thickness we can replace j(rʹ, t-|r -rʹ|/c) d³rʹ by I(rʹ, t - |r - rʹ|/c) dzʹ 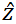. Thus, A(r, t) = Az(r, t)  and

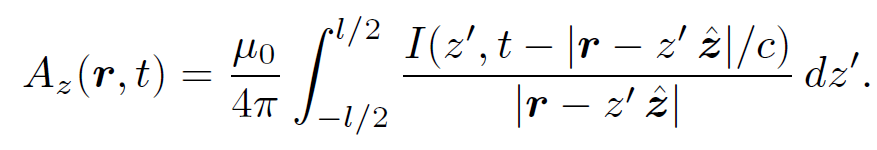 (1.5)

In the region r >> l

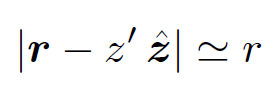 (1.6)

and

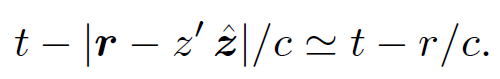 (1.7)

The maximum error in the latter approximation is Δt ~ l/c. This error (which is a time) must be much less than a period of oscillation of the emitted radiation, otherwise the phase of the radiation will be wrong. So

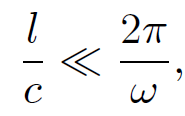 (1.8)

which implies that l << λ, where λ = 2π c/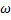 is the wavelength of the emitted radiation. However, we have already assumed that the length of the wire l is much less than the wavelength of the radiation, so the above inequality is automatically satisfied. Thus, in the ''far field" region, r >> λ, we can write

 (1.9)

This integral is easy to perform since the current is uniform along the length of the wire. So,

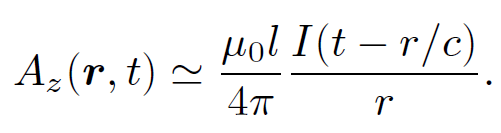 (1.10)

The scalar potential is most conveniently evaluated using the Lorentz gauge condition

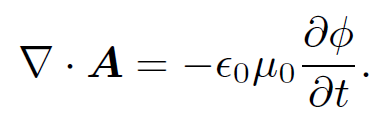 (1.11)

Now,

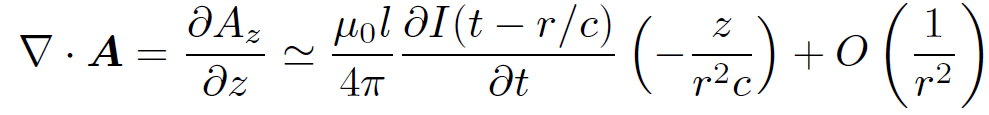 (1.12)

to leading order in r^-1. Thus,

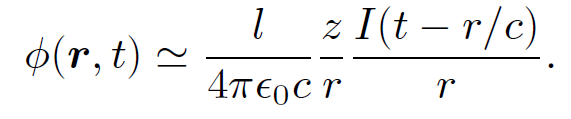 (1.13)

Given the vector and scalar potentials, Eqs. (1.10) and (1.13), respectively, we can evaluate the associated electric and magnetic fields using

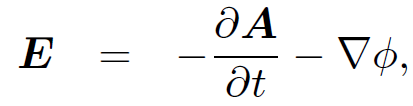
 (1.14)

Note that we are only interested in radiation fields, which fall off like r^-1 with increasing distance from the source. It is easily demonstrated that

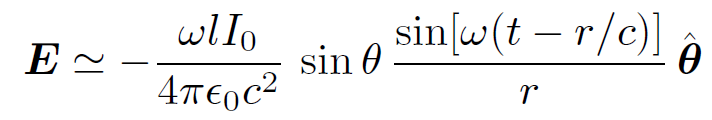 (1.15)

and

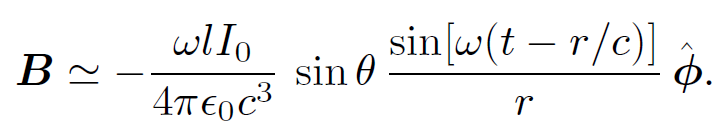(1.16)

Here, (r, θ, ϕ) are standard spherical polar coordinates aligned along the z-axis. The above expressions for the far field (i.e., r >> λ) electromagnetic fields generated by a localized oscillating. Note that the fields are symmetric in the azimuthal angle ϕ. There is no radiation along the axis of the oscillating dipole (i.e., θ = 0), and the maximum emission is in the plane perpendicular to this axis (i.e., θ = π/2). The average power crossing a spherical surface S (whose radius is much greater than λ) is

 (1.17)

where the average is over a single period of oscillation of the wave, and the Poynting flux is given by

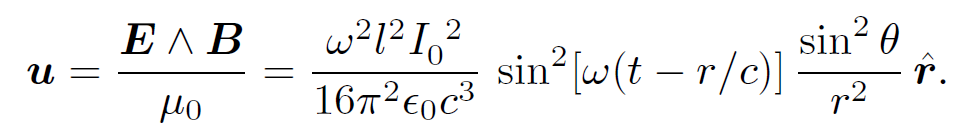 (1.18)

It follows that

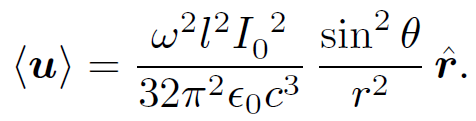 (1.19)

Note that the energy flux is radially outwards from the source. The total power flux across S is given by

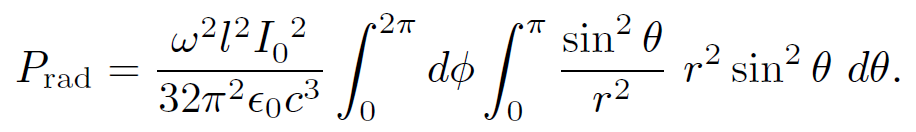 (1.20)

Thus,

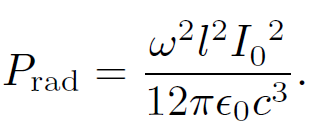 (1.21)

The total flux is independent of the radius of S, as is to be expected if energy is conserved. Recall that for a resistor of resistance R the average ohmic heating power is

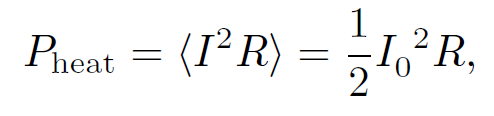 (1.22)

assuming that I = I₀ cos 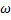t. It is convenient to define the ''radiation resistance" of a Hertzian dipole antenna:

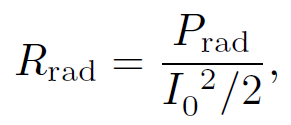 (1.23)

so that

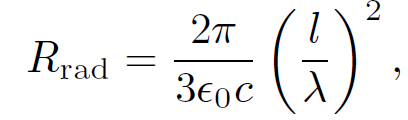 (1.24)

where λ = 2π c/ is the wavelength of the radiation. In fact,

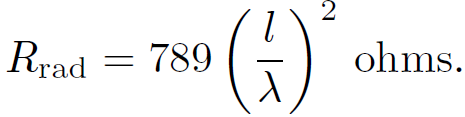 (1.25)

In the theory of electrical circuits, antennas are conventionally represented as resistors whose resistance is equal to the characteristic radiation resistance of the antenna plus its real resistance. The power loss I₀²R_rad/2 associated with the radiation resistance is due to the emission of electromagnetic radiation. The power loss I₀²R/2  associated with the real resistance is due to ohmic heating of the antenna. Note that the formula (1.25) is only valid for l << λ. This suggests that R_red << R for most Hertzian dipole antennas; i.e., the radiated power is swamped by the ohmic losses. Thus, antennas whose lengths are much less than that of the emitted radiation tend to be extremely inefficient. In fact, it is necessary to have l ~ λ in order to obtain an efficient antenna. The simplest practical antenna is the ''half-wave antenna," for which l = λ/2. This can be analyzed as a series of Hertzian dipole antennas stacked on top of one another, each slightly out of phase with its neighbours. The characteristic radiation resistance of a half-wave antenna is

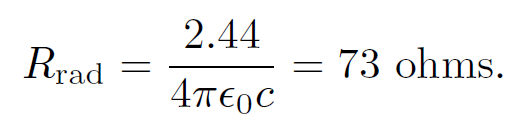 (1.26)

Antennas can be used to receive electromagnetic radiation. The incoming wave induces a voltage in the antenna which can be detected in an electrical circuit connected to the antenna. In fact, this process is equivalent to the emission of electromagnetic waves by the antenna viewed in reverse. It is easily demonstrated that antennas most readily detect electromagnetic radiation incident from those directions in which they preferentially emit radiation. Thus, a Hertzian dipole antenna is unable to detect radiation incident along its axis, and most efficiently detects radiation incident in the plane perpendicular to this axis. In the theory of electrical circuits, a receiving antenna is represented as an e.m.f in series with a resistor. The e.m.f., V₀ cos 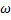t, represents the voltage induced in the antenna by the incoming wave. The resistor, R_rad, represents the power re-radiated by the antenna (here, the real resistance of the antenna is neglected). Let us represent the detector circuit as a single load resistor R_load connected in series with the antenna. The question is: how can we choose R_load so that the maximum power is extracted from the wave and transmitted to the load resistor? According to Ohm's law:

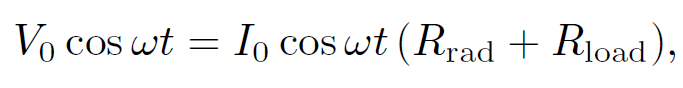 (1.27)

where I = I₀ cos !t is the current induced in the circuit. The power input to the circuit is

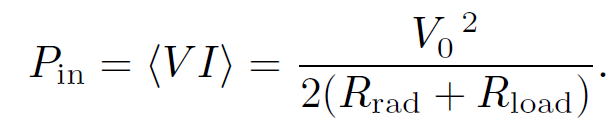 (1.28)

The power transferred to the load is

 (1.29)

The power re-radiated by the antenna is

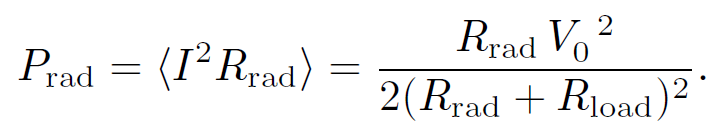 (1.30)

Note that P_in = P_load + P_rad. The maximum power transfer to the load occurs when

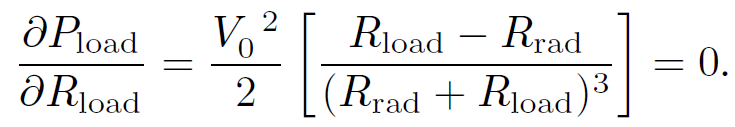 (1.31)

Thus, the maximum transfer rate corresponds to

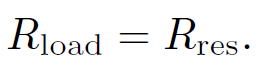 (1.32)

In other words, the resistance of the load circuit must match the radiation resistance of the antenna. For this optimum case,

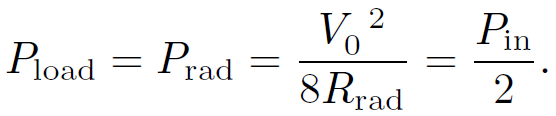 (1.33)

So, in the optimum case half of the power absorbed by the antenna is immediately re-radiated. Clearly, an antenna which is receiving electromagnetic radiation is also emitting it. This is how the BBC catch people who do not pay their television license fee in England. They have vans which can detect the radiation emitted by a TV aerial whilst it is in use (they can even tell which channel you are watching!). For a Hertzian dipole antenna interacting with an incoming wave whose electric field has an amplitude E₀ we expect

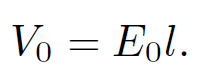 (1.34)

Here, we have used the fact that the wavelength of the radiation is much longer than the length of the antenna. We have also assumed that the antenna is properly aligned (i.e., the radiation is incident perpendicular to the axis of the antenna). The Poynting flux of the incoming wave is

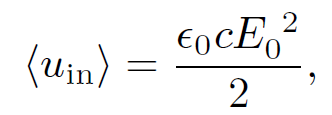 (1.35)

whereas the power transferred to a properly matched detector circuit is

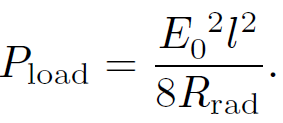 (1.36)

Consider an idealized antenna in which all incoming radiation incident on some area A_eff is absorbed and then magically transferred to the detector circuit with no re-radiation. Suppose that the power absorbed from the idealized antenna matches that absorbed from the real antenna. This implies that

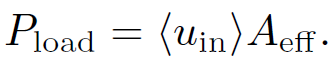 (1.37)

The quantity A_eff is called the ''effective area" of the antenna; it is the area of the idealized antenna which absorbs as much net power from the incoming wave as the actual antenna. Thus,

 (1.38)

giving

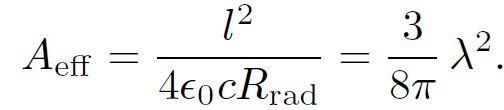 (1.39)

It is clear that the effective area of a Hertzian dipole antenna is of order the wavelength squared of the incoming radiation. For a properly aligned half-wave antenna

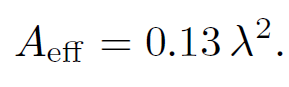 (1.40)

Thus, the antenna, which is essentially one dimensional with length λ/2, acts as if it is two dimensional, with width 0,26 λ, as far as its absorption of incoming electromagnetic radiation is concerned.