The main reason for sampling is to get an idea of the properties of the whole population. For example, if we want to know the mean of a population, our best guess is the mean of a representative sample, and similarly for the median. Naturally,  a bigger sample will lead to a more reliable estimate.

Measures of spread in a sample can be used to estimate the spread of a population, but be careful! When you calculate the standard deviation of a sample,  you use deviations from the mean of the sample, not the population. The sample mean is the best estimate of the population mean, but it is not perfect. The best estimate of the population mean would be made by using the squared deviations from the population mean, not the sample mean; but in most cases this is not available. When the population mean is not known, it can be shown that the best estimate of the population variance is found by summing the squared deviations from the sample mean, and then dividing by n − 1, where n is the sample size.

Usually we write m for the sample mean, and s for the estimate of the standard deviation found by dividing the sum of the squared deviations from m by n−1.

That is, if the readings in a sample are x₁,x₂,...,x_n, then the sample standard deviation is

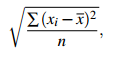
but the best estimate of the population standard deviation is

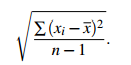
To get a 95% confidence interval for the mean of a normal (or approximately normal) population (such as human attributes like height and weight, or family incomes for large groups like cities), one takes a sample—let’s say its mean is x^-—and use

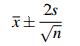
where, if the population standard deviation is known, σ say, we use s = σ; but if the population standard deviation is not known, we use

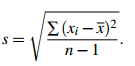
Sample Problem 1.1 You observe the output of a machine for 4 h. The machine produces the following number of screws per hour:

hour 1 : 20,050screws

hour 2 : 19,990screws

hour 3 : 20,020screws

hour 4 : 19,980screws.

Assuming these are typical hours of production, and assuming the hourly production follows approximately a normal distribution, find a 95% confidence interval for hourly screw production.

Solution. The mean is

(20,050+19,990+20,020+19,980)/4= 20,010.

The sum of squared deviations is

402+202+102+302= 3,000.

So the standard deviation estimate is

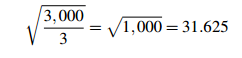
and the 95% range is

20,010±63.25.

In any kind of sampling, it is important to avoid bias, if we want to use the results to make predictions about the whole population.

Perhaps the most common type of bias in sampling is the convenience sample.

For example, an interviewer might wait outside a supermarket door and ask customers a question as they arrive. The customers at a supermarket might not be representative of the whole population. Another example is the telephone survey,  which is biased toward those who have their telephones handy.

Another example of bias is self-selection. We have all seen surveys in newspapers and on television where the public are invited to respond. This type of survey will over-represent those who feel strongly about the topic. Another form of selfselection is when opinions are sought from a sample but only those who choose to respond are counted. This is called a voluntary response sample.

Sometimes the survey itself is biased. The question itself might suggest an answer.

A common form of sampling is an observational study. These are often conducted to test drugs, production methods, and so on. For example, to test whether too much chocolate increases the chance of diabetes, 100 rats were fed one pound of chocolate a day for 6 months. Fifteen percent developed diabetes, while the percentage among rats is only 3%. This does not prove that too much chocolate causes diabetes; other factors might be the increased calories in the diet, lack of exercise, laboratory conditions, and so on. This interference from other factors is called confounding.

Sample Problem 1.2 A sociologist wants to know the opinions of employed adult women about government funding for day care. She obtains a list of the 520 members of a local business and professional women’s club and mails a questionnaire to 100 of these women selected at random. Only 48 questionnaires are returned. What are the population and the sample in this survey? Is this survey biased?

Solution. The population is all employed adult women in the area represented by the club, and the sample is the set of 100 women randomly chosen. The study is almost certainly biased; business and professional women are not representative of all employed women, and the fact that only those who chose to respond are included will very probably affect the result.

To avoid confounding, a comparative study, also called an experimental study,  is usually conducted. In this case other factors are controlled. The experimental subjects are divided into two groups, the experimental group and the control group.

Both groups receive exactly the same treatment except for the variable being tested.

For example, in the rat experiment, one could use 200 rats instead of 100. The original set of rats is the experimental group, while the new 100 are the control group. Other factors (laboratory conditions, calorie intake) are the same for the two groups; only the one variable—the amount of chocolate—varies between the two groups.

This technique works well when testing inanimate objects, or rats, but what if the experimental subjects are people? For example, suppose a new drug is being tested.

The very fact that you know you are receiving a drug, or that you know there is a new drug available and you are not receiving it, could influence your reaction.

For this reason, placebos are used. A placebo is a fake drug, looking exactly the same as the actual drug but not containing the active ingredient. For example, if the tablets used in an experiment contain 5 g of a new hormone substitute, the

placebo might instead contain 5 g of sugar. (This is a common example, and even if sugar and salt are not involved, placebo drugs are often called “sugar pills” or “salt pills.”) Subjects in medical experiments have a tendency to respond favorably to any treatment, whether the real one or the placebo; this is called the “placebo effect.”

In experiments involving placebos, it is standard practice that neither the participants nor those who test for effects are told which treatment was received.