


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 23-11-2018
Date: 23-11-2018
Date: 25-11-2018
|
 |
 |
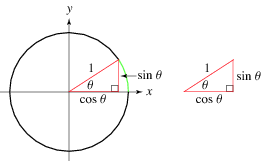
The cosine function 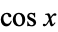 is one of the basic functions encountered in trigonometry (the others being the cosecant,cotangent, secant, sine, and tangent). Let
is one of the basic functions encountered in trigonometry (the others being the cosecant,cotangent, secant, sine, and tangent). Let 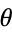 be an angle measured counterclockwise from the x-axis along the arc of the unit circle. Then
be an angle measured counterclockwise from the x-axis along the arc of the unit circle. Then  is the horizontal coordinate of the arc endpoint.
is the horizontal coordinate of the arc endpoint.
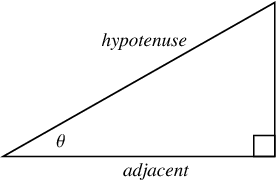
The common schoolbook definition of the cosine of an angle  in a right triangle (which is equivalent to the definition just given) is as the ratio of the lengths of the side of the triangle adjacent to the angle and the hypotenuse, i.e.,
in a right triangle (which is equivalent to the definition just given) is as the ratio of the lengths of the side of the triangle adjacent to the angle and the hypotenuse, i.e.,
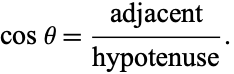 |
(1) |
A convenient mnemonic for remembering the definition of the sine, cosine, and tangent is SOHCAHTOA (sine equals opposite over hypotenuse, cosine equals adjacent over hypotenuse, tangent equals opposite over adjacent).
As a result of its definition, the cosine function is periodic with period  . By the Pythagorean theorem,
. By the Pythagorean theorem,  also obeys the identity
also obeys the identity
 |
(2) |
 |
The definition of the cosine function can be extended to complex arguments 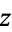 using the definition
using the definition
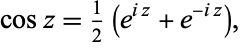 |
(3) |
where e is the base of the natural logarithm and i is the imaginary number. Cosine is an entire function and is implemented in the Wolfram Language as Cos[z].
A related function known as the hyperbolic cosine is similarly defined,
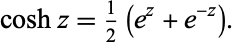 |
(4) |
The cosine function has a fixed point at 0.739085... (OEIS A003957), a value sometimes known as the Dottie number(Kaplan 2007).
The cosine function can be defined analytically using the infinite sum
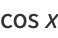 |
 |
 |
(5) |
 |
 |
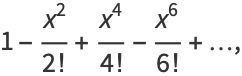 |
(6) |
or the infinite product
![cosx=product_(n=1)^infty[1-(4x^2)/(pi^2(2n-1)^2)].](http://mathworld.wolfram.com/images/equations/Cosine/NumberedEquation5.gif) |
(7) |

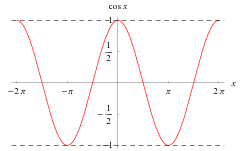
A close approximation to  for
for ![x in [0,1]](http://mathworld.wolfram.com/images/equations/Cosine/Inline15.gif) is
is
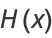 |
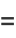 |
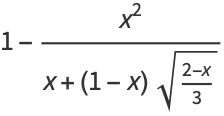 |
(8) |
 |
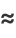 |
 |
(9) |
(Hardy 1959), where the difference between 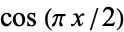 and Hardy's approximation is plotted above.
and Hardy's approximation is plotted above.
The cosine obeys the identity
![cos(ntheta)=2costhetacos[(n-1)theta]-cos[(n-2)theta]](http://mathworld.wolfram.com/images/equations/Cosine/NumberedEquation6.gif) |
(10) |
and the multiple-angle formula
![cos(nx)=sum_(k=0)^n(n; k)cos^kxsin^(n-k)xcos[1/2(n-k)pi],](http://mathworld.wolfram.com/images/equations/Cosine/NumberedEquation7.gif) |
(11) |
where 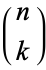 is a binomial coefficient. It is related to
is a binomial coefficient. It is related to 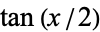 via
via
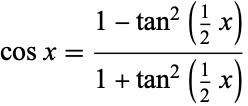 |
(12) |
(Trott 2006, p. 39).
Summation of  from
from 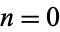 to
to 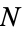 can be done in closed form as
can be done in closed form as
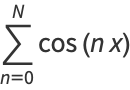 |
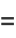 |
![R[sum_(n=0)^(N)e^(inx)]](http://mathworld.wolfram.com/images/equations/Cosine/Inline30.gif) |
(13) |
 |
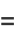 |
![R[(e^(i(N+1)x)-1)/(e^(ix)-1)]](http://mathworld.wolfram.com/images/equations/Cosine/Inline33.gif) |
(14) |
 |
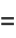 |
![R[(e^(i(N+1)x/2))/(e^(ix/2))(e^(i(N+1)x/2)-e^(-i(N+1)x/2))/(e^(ix/2)-e^(-ix/2))]](http://mathworld.wolfram.com/images/equations/Cosine/Inline36.gif) |
(15) |
 |
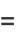 |
![(sin[1/2(N+1)x])/(sin(1/2x))R[e^(iNx/2)]](http://mathworld.wolfram.com/images/equations/Cosine/Inline39.gif) |
(16) |
 |
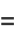 |
![(cos(1/2Nx)sin[1/2(N+1)x])/(sin(1/2x)).](http://mathworld.wolfram.com/images/equations/Cosine/Inline42.gif) |
(17) |
Similarly,
![sum_(n=0)^inftyp^ncos(nx)=R[sum_(n=0)^inftyp^ne^(inx)],](http://mathworld.wolfram.com/images/equations/Cosine/NumberedEquation9.gif) |
(18) |
where  . The exponential sum formula gives
. The exponential sum formula gives
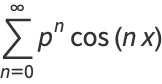 |
 |
![R[(1-pe^(-ix))/(1-2pcosx+p^2)]](http://mathworld.wolfram.com/images/equations/Cosine/Inline46.gif) |
(19) |
 |
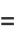 |
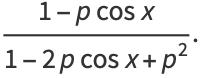 |
(20) |
The sum of 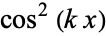 can also be done in closed form,
can also be done in closed form,
![sum_(k=0)^Ncos^2(kx)=1/4{3+2N+cscxsin[x(1+2N)]}.](http://mathworld.wolfram.com/images/equations/Cosine/NumberedEquation10.gif) |
(21) |
The Fourier transform of 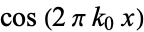 is given by
is given by
](http://mathworld.wolfram.com/images/equations/Cosine/Inline52.gif) |
 |
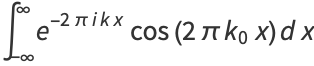 |
(22) |
 |
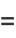 |
![1/2[delta(k-k_0)+delta(k+k_0)],](http://mathworld.wolfram.com/images/equations/Cosine/Inline57.gif) |
(23) |
where 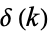 is the delta function.
is the delta function.
Cvijović and Klinowski (1995) note that the following series
 |
(24) |
has closed form for 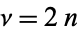 ,
,
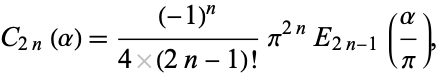 |
(25) |
where 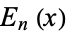 is an Euler polynomial.
is an Euler polynomial.
A definite integral involving  is given by
is given by
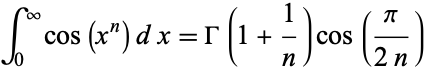 |
(26) |
for 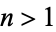 where
where 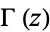 is the gamma function (T. Drane, pers. comm., Apr. 21, 2006).
is the gamma function (T. Drane, pers. comm., Apr. 21, 2006).
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Circular Functions." §4.3 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 71-79, 1972.
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 215, 1987.
Cvijović, D. and Klinowski, J. "Closed-Form Summation of Some Trigonometric Series." Math. Comput. 64, 205-210, 1995.
Hansen, E. R. A Table of Series and Products. Englewood Cliffs, NJ: Prentice-Hall, 1975.
Hardy, G. H. Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, 3rd ed. New York: Chelsea, p. 68, 1959.
Jeffrey, A. "Trigonometric Identities." §2.4 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 111-117, 2000.
Kaplan, S. R. "The Dottie Number." Math. Mag. 80, 73-74, 2007.
Project Mathematics. "Sines and Cosines, Parts I-III." Videotape. http://www.projectmathematics.com/sincos1.htm.
Sloane, N. J. A. Sequence A003957 in "The On-Line Encyclopedia of Integer Sequences."
Spanier, J. and Oldham, K. B. "The Sine  and Cosine
and Cosine 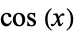 Functions." Ch. 32 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 295-310, 1987.
Functions." Ch. 32 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 295-310, 1987.
Tropfke, J. Teil IB, §1. "Die Begriffe des Sinus und Kosinus eines Winkels." In Geschichte der Elementar-Mathematik in systematischer Darstellung mit besonderer Berücksichtigung der Fachwörter, fünfter Band, zweite aufl. Berlin and Leipzig, Germany: de Gruyter, pp. 11-23, 1923.
Trott, M. The Mathematica GuideBook for Symbolics. New York: Springer-Verlag, 2006. http://www.mathematicaguidebooks.org/.
Zwillinger, D. (Ed.). "Trigonometric or Circular Functions." §6.1 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 452-460, 1995.



|
|
|
|
"عادة ليلية" قد تكون المفتاح للوقاية من الخرف
|
|
|
|
|
|
|
ممتص الصدمات: طريقة عمله وأهميته وأبرز علامات تلفه
|
|
|
|
|
|
|
المجمع العلمي للقرآن الكريم يقيم جلسة حوارية لطلبة جامعة الكوفة
|
|
|