In this section we consider several classic results concerning matchings. We begin with a few more definitions. Given a graph G and Amatching M ,an M-alternating path is a path in G where the edges alternate between M-edges and non-M-edges .An M-augmenting path is an M-alternating path where both end vertices are M-unsaturated. As an example, consider the graph G and the matching M indicated in Figure 1.1.

 An example of an M-alternating path is c, a, d, e, i. An example of an M-augmenting path is j, g, f, a, c, b. The reason for calling such a path “Maugmenting”  will become apparent soon.

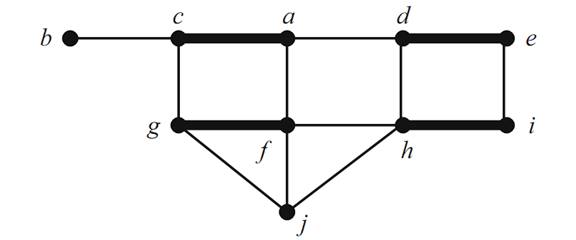
                                    FIGURE 1.1. The graph G and matching M.

The following result is due to Berge [2].

Theorem 1.1 (Berge’s Theorem).

Let M be a matching in a graph G. M is maximum if and only if G contains no M-augmenting paths.

Proof. First, assume that M is a maximum matching, and suppose that P :

v₁,v₂,...,v_k is an M-augmenting path. Due to the alternating nature of M-augmenting paths, it must be that k is even and that the edges v₂v₃, v₄v₅, ... , v_k−2v_k−1 are all edges of M. We also see that the edges v₁v₂, v₃v₄, ... , v_k−1 v_k are not edges of M (Figure 1.2).

 But then if we define the set of edgesM₁ to be

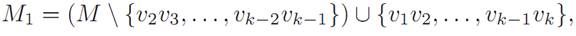
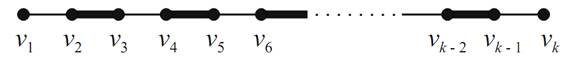
                                  FIGURE 1.2. An M-augmenting path.

then M₁ is a matching that contains one more edge than M, a matching that we assumed to be maximum. This is a contradiction, and we can conclude that G contains no M-augmenting paths.

For the converse, assume that G has noM-augmenting paths, and suppose that M^/is a matching that is larger than M. Define a subgraph H of G as follows: Let V (H)= V (G) and let E(H) be the set of edges of G that appear in exactly one of M and M^/. Now consider some properties of this subgraph H. Since each of the vertices of G lies on at most one edge from M and at most one edge from M^/, it must be that the degree (in H) of each vertex of H is at most 2. This implies that each connected component of H is either a single vertex, a path, or a cycle. If a component is a cycle, then it must be an even cycle, since the edges alternate between M-edges and M^/-edges. So, since |M^/| > |M|, there must be at least one component of H that is a path that begins and ends with edges from M^/.

But this path is an M-augmenting path, contradicting our assumption. Therefore, no such matching M^/ can exist—implying that M is maximum.

         Before we see Hall’s classic matching theorem, we need to define one more term. If G is a bipartite graph with partite sets X and Y , we say that X can be matched into Y if there exists a matching in G that saturates the vertices of X. Consider the two examples in Figure 1.3. In the bipartite graph on the left,

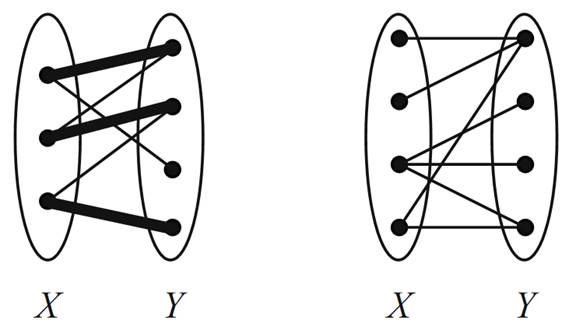
                                                FIGURE 1.3.

we see that X can be matched into Y . In the graph on the right, though, it is impossible to match X into Y. What conditions on a bipartite graph must exist if we want to match one partite set into the other? The answer to this question is found in the following result of Hall [3] (Philip, not Monty).

Recall that the neighborhood of a set of vertices S, denoted by N(S),is the union of the neighborhoods of the vertices of S.

Theorem 1.2 (Hall’s Theorem).

 Let G be a bipartite graph with partite sets X and Y . X can be matched into Y if and only if |N(S)|≥|S| for all subsets S of X.

Proof. First suppose that X can be matched into Y ,and let S be some subset of X. Since S itself is also matched into Y , we see immediately that |S|≤|N(S)|

(see Figure 1.4). Now suppose that |N(S)|≥|S| for all subsets S of X, and

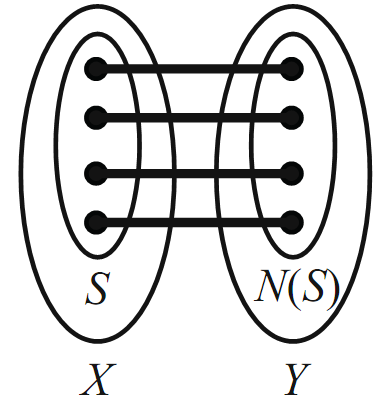
                        FIGURE 1.4.

let M be a maximum matching. Suppose that u ∈ X is not saturated by M (see Figure 1.5).Define the set A to be the set of vertices of G that can be joined to u

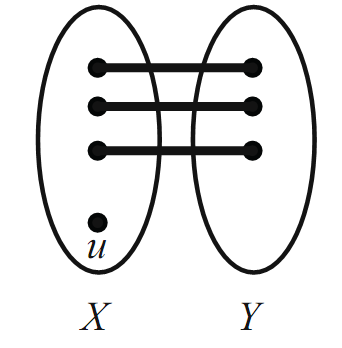
                        FIGURE 1.5.

by an M-alternating path. Let S = A∩X, and let T = A∩Y (see Figure 1.6). Notice now that Berge’s Theorem implies that every vertex of T is saturated by

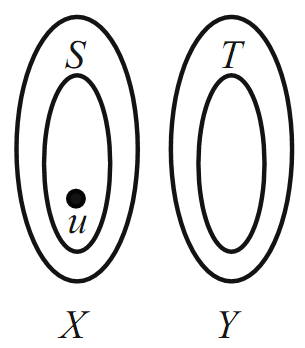
                 FIGURE 1.6.

M and that u is the only unsaturated vertex of S. That is, every vertex of T is saturated, and every vertex of S {u} is saturated. This implies that |T | = |S|−1.

It follows from Berge’s Theorem and the definition of T that N(S)= T . But then we have that |N(S)| = |S|− 1 < |S|, and this is a contradiction. We conclude that such a vertex u cannot exist in X and that M saturates all of X.

Given some family of sets X,a system of distinct representatives, or SDR ,for the sets in X can be thought of as a “representative” collection of distinct elements from the sets of X. For instance, let S₁, S₂, S₃, S₄,and S₅ be defined as follows:

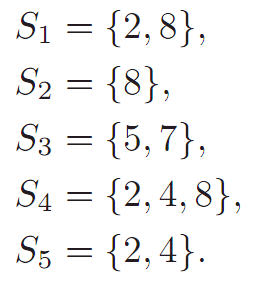
The family X₁ = {S₁,S₂,S₃,S₄} does have an SDR, namely {2, 8, 7, 4}.The family X2 = {S₁,S₂,S₄,S₅} does not have an SDR.

So under what conditions will a finite family of sets have an SDR? We answer this question with the following theorem.

Theorem 1.3.

Let S₁, S₂, ..., S_k be a collection of finite, nonempty sets. This collection has an SDR if and only if for every t ∈{1,...,k}, the union of any t of these sets contains at least t elements.

Proof. Since each of the sets is finite, then of course S = S₁ ∪ S₂ ∪···∪ S_k is finite. Let us say that the elements of S are a₁, ..., a_n.

We now construct a bipartite graph with partite sets X = {S₁,...,S_k} and Y = {a₁,...,a_n} (Figure 1.7). We place an edge between Si and a_j if and only if a_j ∈ S_i.

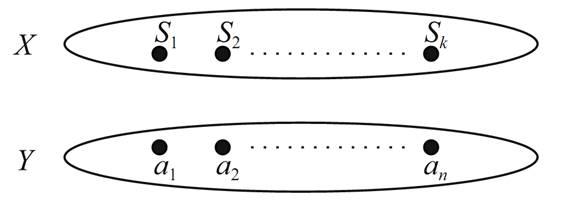
          FIGURE 1.7. Constructing a bipartite graph.

Hall’s Theorem now implies that X can be matched into Y if and only if |A|≤|N(A)| for all subsets A of X. In other words, the collection of sets has an SDR if and only if for every t ∈{1,...,k}, the union of any t of these sets contains at least t elements.

________________________________________________________________________________

1-Combinatorics and Graph Theory, Second Edition, John M. Harris • Jeffry L. Hirst • Michael J. Mossinghoff,2000,page(104-108)

2-C. Berge, Two theorems in graph theory, Proc. Natl. Acad. Sci. USA 43 (1957), 842–844.

3-P. Hall, On representation of subsets, J. London Math. Soc. 10 (1935), 26–30.

4- J. Clark and D. A. Holton, A First Look at Graph Theory, World Scientific, Teaneck, NJ, 1991.

مواضيع ذات صلة

Minimum Spanning Tree

Maximum Leaf Number

Lobster Graph

Labeled Tree

Internal Path Length

Red-Black Tree

Pseudotree

Pseudoforest

Prüfer Code

Planted Tree

Planted Planar Tree

Graph Strength