
The uniqueness theorem
 المؤلف:
Richard Fitzpatrick
المؤلف:
Richard Fitzpatrick
 المصدر:
Classical Electromagnetism
المصدر:
Classical Electromagnetism
 الجزء والصفحة:
p 167
الجزء والصفحة:
p 167
 4-1-2017
4-1-2017
 2415
2415
We have already seen the great value of the uniqueness theorem for Poisson's equation (or Laplace's equation) in our discussion of Helmholtz's theorem. Let us now examine this theorem in detail. Consider a volume V bounded by some surface S. Suppose that we are given the charge density ρ throughout V and the value of the scalar potential ϕS on S. Is this sufficient information to uniquely specify the scalar potential throughout V ? Suppose, for the sake of argument, that the solution is not unique. Let there be two potentials ϕ1 and ϕ2 which satisfy
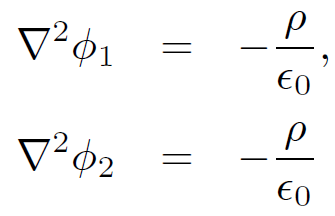 (1.1)
(1.1)
throughout V , and
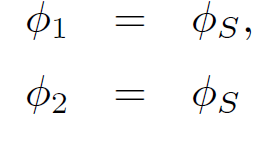 (1.2)
(1.2)
on S. We can form the difference between these two potentials:
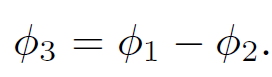 (1.3)
(1.3)
The potential ϕ3 clearly satisfies
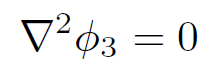 (1.4)
(1.4)
throughout V , and
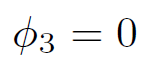 (1.5)
(1.5)
on S. According to vector field theory
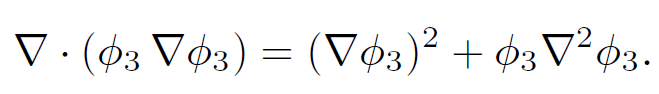 (1.6)
(1.6)
Thus, using Gauss' theorem
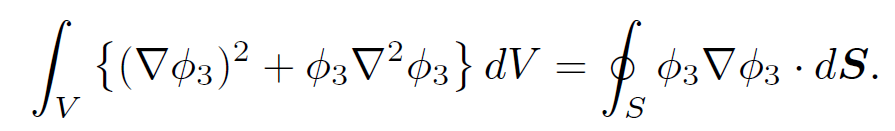 (1.7)
(1.7)
But, ∇2ϕ3 = 0 throughout V and ϕ3 = 0 on S, so the above equation reduces to
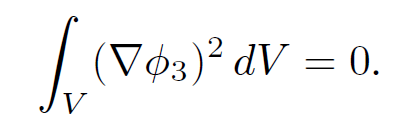 (1.8)
(1.8)
Note that (∇ϕ3)2 is a positive definite quantity. The only way in which the volume integral of a positive definite quantity can be zero is if that quantity itself is zero throughout the volume. This is not necessarily the case for a non-positive definite quantity; we could have positive and negative contributions from various regions inside the volume which cancel one another out. Thus, since (∇ϕ3)2 is positive definite it follows that
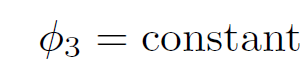 (1.9)
(1.9)
throughout V . However, we know that ϕ3 = 0 on S, so we get
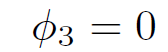 (1.10)
(1.10)
throughout V . In other words,
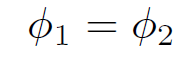 (1.11)
(1.11)
throughout V and on S. Our initial assumption that ϕ1 and ϕ2 are two different solutions of Laplace's equations, satisfying the same boundary conditions, turns out to be incorrect. The fact that the solutions to Poisson's equation are unique is very useful. It means that if we find a solution to this equation no matter how contrived the derivation then this is the only possible solution. One immediate use of the uniqueness theorem is to prove that the electric field inside an empty cavity in a conductor is zero. Recall that our previous proof of this was rather involved, and was also not particularly rigorous. We know that the interior surface of the conductor is at some constant potential V , say. So, we have ϕ = V on the boundary of the cavity and ∇2ϕ = 0 inside the cavity (since it contains no charges). One rather obvious solution to these equations is ϕ = V throughout the cavity. Since the solutions to Poisson's equation are unique this is the only solution. Thus,
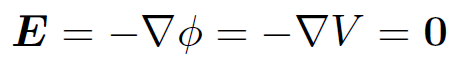 (1.12)
(1.12)
inside the cavity. Suppose that some volume V contains a number of conductors. We know that the surface of each conductor is an equipotential, but, in general, we do not know what potential each surface is at (unless we are specifically told that it is earthed, etc.). However, if the conductors are insulated it is plausible that we might know the charge on each conductor. Suppose that there are N conductors, each carrying a charge Qi (i = 1 to N), and suppose that the region V containing these conductors is filled by a known charge density ρ and bounded by some surface S which is either infinity or an enclosing conductor. Is this enough information to uniquely specify the electric field throughout V? Well, suppose that it is not enough information, so that there are two fields E1 and E2 which satisfy
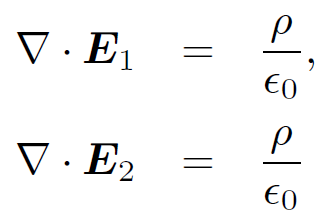 (1.13)
(1.13)
throughout V , with
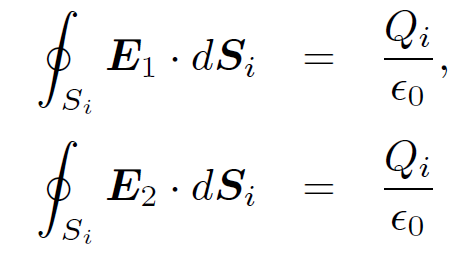 (1.14)
(1.14)
on the surface of the ith conductor, and, finally,
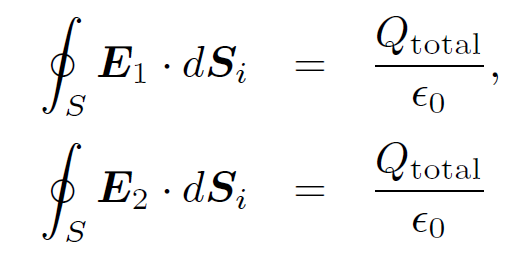 (1.15)
(1.15)
over the bounding surface, where
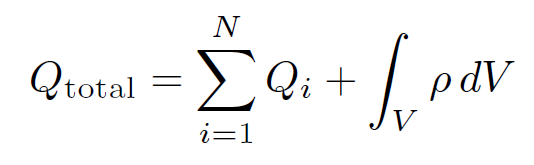 (1.16)
(1.16)
is the total charge contained in volume V. Let us form the difference field
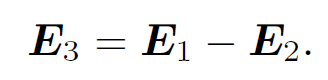 (1.17)
(1.17)
It is clear that
 (1.18)
(1.18)
throughout V , and
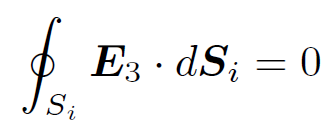 (1.19)
(1.19)
for all i, with
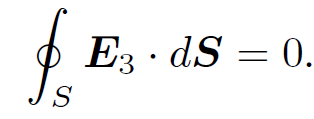 (1.20)
(1.20)
Now, we know that each conductor is at a constant potential, so if
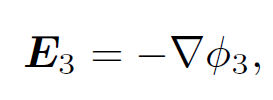 (1.21)
(1.21)
then ϕ3 is a constant on the surface of each conductor. Furthermore, if the outer surface S is infinity then ϕ1 = ϕ2 = ϕ3 = 0 on this surface. If the outer surface is an enclosing conductor then ϕ3 is a constant on this surface. Either way, ϕ3 is constant on S. Consider the vector identity
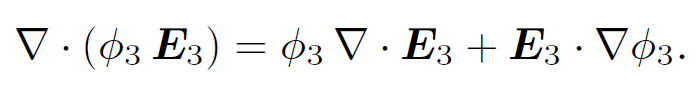 (1.22)
(1.22)
We have ∇. E3 = 0 throughout V and ∇ϕ3 = -E3, so the above identity reduces to
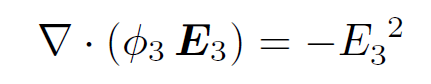 (1.23)
(1.23)
throughout V . Integrating over V and making use of Gauss' theorem yields
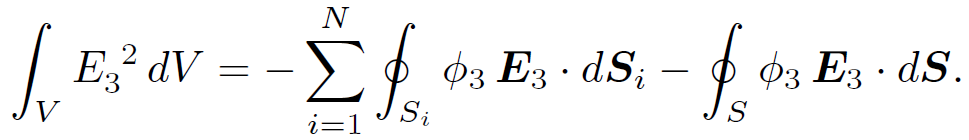 (1.24)
(1.24)
However, ϕ3 is a constant on the surfaces Si and S. So, making use of Eqs. (1.20) and (1.21), we obtain
 (1.25)
(1.25)
Of course, E32 is a positive definite quantity, so the above relation implies that
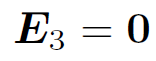 (1.26)
(1.26)
throughout V ; i.e., the fields E1 and E2 are identical throughout V. It is clear that, for a general electrostatic problem involving charges and conductors, if we are given either the potential at the surface of each conductor or the charge carried by each conductor (plus the charge density throughout the volume, etc.) then we can uniquely determine the electric field. There are many other uniqueness theorems which generalize this result still further; i.e., we could be given the potential of some of the conductors and the charge carried by the others and the solution would still be unique.
 الاكثر قراءة في الكهربائية
الاكثر قراءة في الكهربائية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


