


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 4-10-2021
Date: 16-12-2021
Date: 22-9-2021
|
There are two sets of constants that are commonly known as Lebesgue constants. The first is related to approximation of function via Fourier series, which the other arises in the computation of Lagrange interpolating polynomials.
Assume a function  is integrable over the interval
is integrable over the interval ![[-pi,pi]](https://mathworld.wolfram.com/images/equations/LebesgueConstants/Inline2.gif) and
and 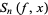 is the
is the 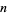 th partial sum of the Fourier series of
th partial sum of the Fourier series of 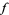 , so that
, so that
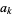 |
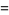 |
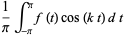 |
(1) |
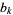 |
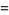 |
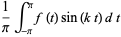 |
(2) |
and
![S_n(f,x)=1/2a_0+{sum_(k=1)^n[a_kcos(kx)+b_ksin(kx)]}.](https://mathworld.wolfram.com/images/equations/LebesgueConstants/NumberedEquation1.gif) |
(3) |
If
 |
(4) |
for all  , then
, then
![S_n(f,x)<=1/piint_0^pi(|sin[1/2(2n+1)theta]|)/(sin(1/2theta))dtheta=L_n,](https://mathworld.wolfram.com/images/equations/LebesgueConstants/NumberedEquation3.gif) |
(5) |
and 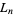 is the smallest possible constant for which this holds for all continuous
is the smallest possible constant for which this holds for all continuous  . The first few values of
. The first few values of 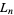 are
are
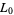 |
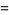 |
 |
(6) |
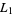 |
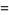 |
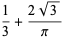 |
(7) |
 |
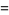 |
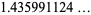 |
(8) |
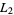 |
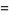 |
 |
(9) |
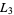 |
 |
![1/7+1/(3pi)[22sin(pi/7)-2cos(pi/(14))+10cos((3pi)/(14))]](https://mathworld.wolfram.com/images/equations/LebesgueConstants/Inline30.gif) |
(10) |
 |
 |
 |
(11) |
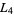 |
 |
![(13)/(2sqrt(3)pi)+1/9+1/pi[7sin((2pi)/9)-5sin(pi/9)-cos(pi/(18))]](https://mathworld.wolfram.com/images/equations/LebesgueConstants/Inline36.gif) |
(12) |
 |
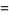 |
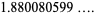 |
(13) |
Some sum formulas for 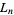 include
include
 |
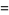 |
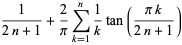 |
(14) |
 |
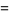 |
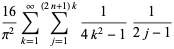 |
(15) |
(Zygmund 1959) and integral formulas include
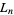 |
 |
![4int_0^infty(tanh[(2n+1)x])/(tanhx)(dx)/(pi^2+4x^2)](https://mathworld.wolfram.com/images/equations/LebesgueConstants/Inline49.gif) |
(16) |
 |
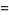 |
![4/(pi^2)int_0^infty(sinh[(2n+1)x])/(sinhx)ln{coth[1/2(2n+1)x]}dx](https://mathworld.wolfram.com/images/equations/LebesgueConstants/Inline52.gif) |
(17) |
(Hardy 1942). For large 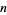 ,
,
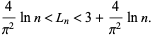 |
(18) |
This result can be generalized for an  -differentiable function satisfying
-differentiable function satisfying
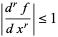 |
(19) |
for all  . In this case,
. In this case,
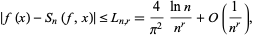 |
(20) |
where
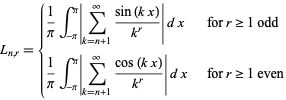 |
(21) |
(Kolmogorov 1935, Zygmund 1959).
Watson (1930) showed that
![lim_(n->infty)[L_n-4/(pi^2)ln(2n+1)]=c,](https://mathworld.wolfram.com/images/equations/LebesgueConstants/NumberedEquation8.gif) |
(22) |
where
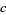 |
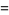 |
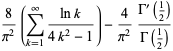 |
(23) |
 |
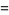 |
![8/(pi^2)[sum_(j=0)^(infty)(lambda(2j+2)-1)/(2j+1)]+4/(pi^2)(2ln2+gamma)](https://mathworld.wolfram.com/images/equations/LebesgueConstants/Inline61.gif) |
(24) |
 |
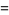 |
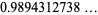 |
(25) |
(OEIS A086052), where 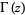 is the gamma function,
is the gamma function,  is the Dirichlet lambda function, and
is the Dirichlet lambda function, and  is the Euler-Mascheroni constant.
is the Euler-Mascheroni constant.
Define the 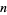 th Lebesgue constant for the Lagrange interpolating polynomial by
th Lebesgue constant for the Lagrange interpolating polynomial by
 |
(26) |
It is then true that
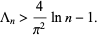 |
(27) |
The efficiency of a Lagrange interpolation is related to the rate at which 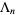 increases. Erdős (1961) proved that there exists a positive constant such that
increases. Erdős (1961) proved that there exists a positive constant such that
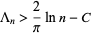 |
(28) |
for all 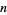 . Erdős (1961) further showed that
. Erdős (1961) further showed that
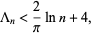 |
(29) |
so (◇) cannot be improved upon.
REFERENCES:
Finch, S. R. "Lebesgue Constants." §4.2 in Mathematical Constants. Cambridge, England: Cambridge University Press, pp. 250-255, 2003.
Erdős, P. "Problems and Results on the Theory of Interpolation, II." Acta Math. Acad. Sci. Hungary 12, 235-244, 1961.
Hardy, G. H. "Note on Lebesgue's Constants in the Theory of Fourier Series." J. London Math. Soc. 17, 4-13, 1942.
Kolmogorov, A. N. "Zur Grössenordnung des Restgliedes Fourierscher reihen differenzierbarer Funktionen." Ann. Math. 36, 521-526, 1935.
Sloane, N. J. A. Sequence A086052 in "The On-Line Encyclopedia of Integer Sequences."
Watson, G. N. "The Constants of Landau and Lebesgue." Quart. J. Math. Oxford 1, 310-318, 1930.
Zygmund, A. G. Trigonometric Series, 2nd ed., Vols. 1-2. Cambridge, England: Cambridge University Press, 1959.



|
|
|
|
التوتر والسرطان.. علماء يحذرون من "صلة خطيرة"
|
|
|
|
|
|
|
مرآة السيارة: مدى دقة عكسها للصورة الصحيحة
|
|
|
|
|
|
|
المركز العراقي لتوثيق جرائم التطرُّف: مؤتمر ذاكرة الألم سيشهد مشاركة 13 دولة عربية وأجنبية
|
|
|