


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 10-7-2021
Date: 14-6-2021
Date: 6-7-2021
|
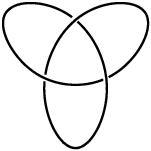 |
 |
The trefoil knot  , also called the threefoil knot or overhand knot, is the unique prime knot with three crossings. It is a (3, 2)-torus knot and has braid word
, also called the threefoil knot or overhand knot, is the unique prime knot with three crossings. It is a (3, 2)-torus knot and has braid word 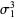 . The trefoil and its mirror image are not equivalent, as first proved by Dehn (1914). In other words, the trefoil knot is not amphichiral. It is, however, invertible, and has Arf invariant 1.
. The trefoil and its mirror image are not equivalent, as first proved by Dehn (1914). In other words, the trefoil knot is not amphichiral. It is, however, invertible, and has Arf invariant 1.
Its laevo form is implemented in the Wolfram Language, as illustrated above, as KnotData["Trefoil"].
M. C. Escher's woodcut "Knots" (Bool et al. 1982, pp. 128 and 325; Forty 2003, Plate 71) depicts three trefoil knots composed of differing types of strands. A preliminary study (Bool et al. 1982, p. 123) depicts another trefoil.

The animation above shows a series of gears arranged along a Möbius strip trefoil knot (M. Trott).
The bracket polynomial can be computed as follows.
 |
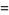 |
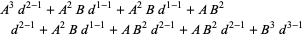 |
(1) |
 |
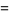 |
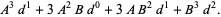 |
(2) |
Plugging in
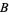 |
 |
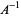 |
(3) |
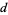 |
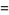 |
 |
(4) |
gives
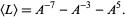 |
(5) |
The corresponding Kauffman polynomial X is then given by
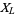 |
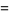 |
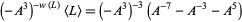 |
(6) |
 |
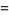 |
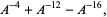 |
(7) |
where the writhe 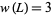 (Kauffman 1991, p. 35; Livingston 1993, p. 219)
(Kauffman 1991, p. 35; Livingston 1993, p. 219)
The Alexander polynomial 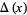 , BLM/Ho polynomial
, BLM/Ho polynomial 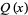 , Conway polynomial
, Conway polynomial 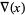 , HOMFLY polynomial
, HOMFLY polynomial 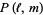 , Jones polynomial
, Jones polynomial  , and Kauffman polynomial F
, and Kauffman polynomial F 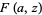 of the trefoil knot are
of the trefoil knot are
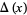 |
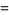 |
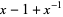 |
(8) |
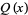 |
 |
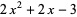 |
(9) |
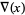 |
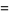 |
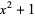 |
(10) |
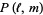 |
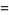 |
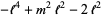 |
(11) |
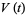 |
 |
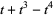 |
(12) |
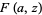 |
 |
 |
(13) |
Here, 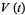 corresponds to the right-hand trefoil.
corresponds to the right-hand trefoil.
There are no other knots on 10 or fewer crossings sharing the same Alexander polynomial, BLM/Ho polynomial, or Jones polynomial.
The knot group of the trefoil knot is
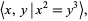 |
(14) |
or equivalently
 |
(15) |
(Rolfsen 1976, pp. 52 and 61).
REFERENCES:
Bar-Natan, D. "The Knot  ." https://www.math.toronto.edu/~drorbn/KAtlas/Knots/3.1.html.
." https://www.math.toronto.edu/~drorbn/KAtlas/Knots/3.1.html.
Bool, F. H.; Kist, J. R.; Locher, J. L.; and Wierda, F. M. C. Escher: His Life and Complete Graphic Work. New York: Abrams, 1982.
Claremont High School. "Trefoil_Knot Movie." Binary encoded QuickTime movie. ftp://chs.cusd.claremont.edu/pub/knot/trefoil.cpt.bin.
Crandall, R. E. Mathematica for the Sciences. Redwood City, CA: Addison-Wesley, 1993.
Dehn, M. "Die beiden Kleeblattschlingen." Math. Ann. 75, 402-413, 1914.
Escher, M. C. "Knots." Woodcut in red, green and brown, printed from 3 blocks. 1965. https://www.mcescher.com/Gallery/recogn-bmp/LW444.jpg.
Forty, S. M.C. Escher. Cobham, England: TAJ Books, 2003.
Kauffman, L. H. Knots and Physics. Singapore: World Scientific, pp. 8 and 29-35, 1991.
KnotPlot. " ." https://newweb.cecm.sfu.ca/cgi-bin/KnotPlot/KnotServer/kserver?ncomp=1&ncross=3&id=1.
." https://newweb.cecm.sfu.ca/cgi-bin/KnotPlot/KnotServer/kserver?ncomp=1&ncross=3&id=1.
Livingston, C. Knot Theory. Washington, DC: Math. Assoc. Amer., 1993.
Nordstrand, T. "Threefoil Knot." https://jalape.no/math/tknottxt.
Pappas, T. "The Trefoil Knot." The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, p. 96, 1989.
Rolfsen, D. Knots and Links. Wilmington, DE: Publish or Perish Press, pp. 51 and 60, 1976.
Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, p. 265, 1999.



|
|
|
|
دخلت غرفة فنسيت ماذا تريد من داخلها.. خبير يفسر الحالة
|
|
|
|
|
|
|
ثورة طبية.. ابتكار أصغر جهاز لتنظيم ضربات القلب في العالم
|
|
|
|
|
|
|
العتبة العباسية المقدسة تستعد لإطلاق الحفل المركزي لتخرج طلبة الجامعات العراقية
|
|
|