


 تاريخ الرياضيات
تاريخ الرياضيات
 الرياضيات في الحضارات المختلفة
الرياضيات في الحضارات المختلفة 
 الرياضيات المتقطعة
الرياضيات المتقطعة
 الجبر
الجبر
 الهندسة
الهندسة 
 المعادلات التفاضلية و التكاملية
المعادلات التفاضلية و التكاملية 
 التحليل
التحليل
 علماء الرياضيات
علماء الرياضيات |
Read More
Date: 18-9-2019
Date: 5-9-2019
Date: 15-9-2019
|

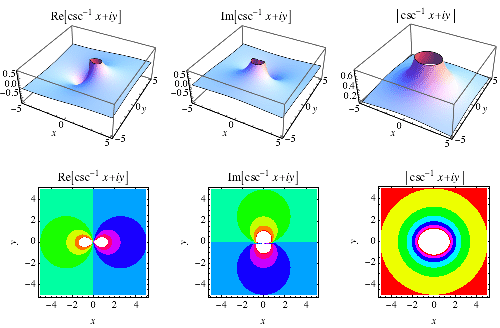 |
The inverse cosecant is the multivalued function 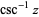 (Zwillinger 1995, p. 465), also denoted
(Zwillinger 1995, p. 465), also denoted 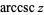 (Abramowitz and Stegun 1972, p. 79; Spanier and Oldham 1987, p. 332; Harris and Stocker 1998, p. 315; Jeffrey 2000, p. 125), that is the inverse function of the cosecant. The variants
(Abramowitz and Stegun 1972, p. 79; Spanier and Oldham 1987, p. 332; Harris and Stocker 1998, p. 315; Jeffrey 2000, p. 125), that is the inverse function of the cosecant. The variants 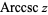 (e.g., Beyer 1987, p. 141; Bronshtein and Semendyayev, 1997, p. 70) and
(e.g., Beyer 1987, p. 141; Bronshtein and Semendyayev, 1997, p. 70) and 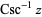 are sometimes used to refer to explicit principal values of the inverse cosecant, although this distinction is not always made (e.g,. Zwillinger 1995, p. 466). Worse yet, the notation
are sometimes used to refer to explicit principal values of the inverse cosecant, although this distinction is not always made (e.g,. Zwillinger 1995, p. 466). Worse yet, the notation  is sometimes used for the principal value, with
is sometimes used for the principal value, with 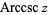 being used for the multivalued function (Abramowitz and Stegun 1972, p. 80). Note that in the notation
being used for the multivalued function (Abramowitz and Stegun 1972, p. 80). Note that in the notation 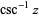 (commonly used in North America and in pocket calculators worldwide),
(commonly used in North America and in pocket calculators worldwide), 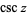 is the cosecant and the superscript
is the cosecant and the superscript 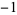 denotes an inverse function, not the multiplicative inverse.
denotes an inverse function, not the multiplicative inverse.
The principal value of the inverse cosecant is implemented as ArcCsc[x] in the Wolfram Language.
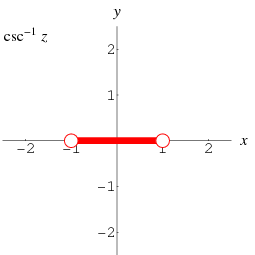
The inverse cosecant is a multivalued function and hence requires a branch cut in the complex plane, which the Wolfram Language's convention places at 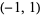 . This follows from the definition of
. This follows from the definition of 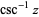 as
as
 |
(1) |
The derivative of 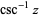 is given by
is given by
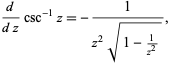 |
(2) |
which simplifies to
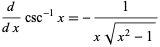 |
(3) |
for 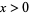 . Its indefinite integral is
. Its indefinite integral is
![intcsc^(-1)zdz=zcsc^(-1)z+ln[z(1+sqrt((z^2-1)/(z^2)))]+C,](http://mathworld.wolfram.com/images/equations/InverseCosecant/NumberedEquation4.gif) |
(4) |
which simplifies to
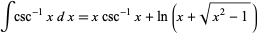 |
(5) |
for 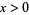 .
.
The inverse cosecant has Taylor series about infinity of
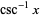 |
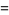 |
 |
(6) |
 |
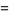 |
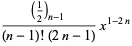 |
(7) |
 |
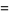 |
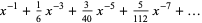 |
(8) |
(OEIS A055786 and A002595), where 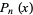 is a Legendre polynomial and
is a Legendre polynomial and 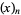 is a Pochhammer symbol.
is a Pochhammer symbol.
The inverse cosecant satisfies
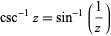 |
(9) |
for 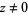 ,
,
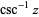 |
 |
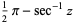 |
(10) |
 |
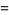 |
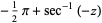 |
(11) |
for all complex 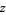 , and
, and
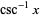 |
 |
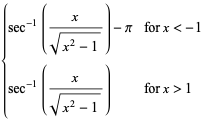 |
(12) |
 |
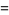 |
 |
(13) |
 |
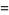 |
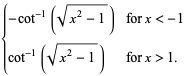 |
(14) |
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). "Inverse Circular Functions." §4.4 in Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 79-83, 1972.
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 142-143, 1987.
Bronshtein, I. N. and Semendyayev, K. A. Handbook of Mathematics, 3rd ed. New York: Springer-Verlag, 1997.
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 6th ed. San Diego, CA: Academic Press, 2000.
Harris, J. W. and Stocker, H. Handbook of Mathematics and Computational Science. New York: Springer-Verlag, p. 315, 1998.
Jeffrey, A. "Inverse Trigonometric and Hyperbolic Functions." §2.7 in Handbook of Mathematical Formulas and Integrals, 2nd ed. Orlando, FL: Academic Press, pp. 124-128, 2000.
Sloane, N. J. A. Sequences A002595/M4233 and A055786 in "The On-Line Encyclopedia of Integer Sequences."
Spanier, J. and Oldham, K. B. "Inverse Trigonometric Functions." Ch. 35 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 331-341, 1987.
Zwillinger, D. (Ed.). "Inverse Circular Functions." §6.3 in CRC Standard Mathematical Tables and Formulae. Boca Raton, FL: CRC Press, pp. 465-467, 1995.



|
|
|
|
التوتر والسرطان.. علماء يحذرون من "صلة خطيرة"
|
|
|
|
|
|
|
مرآة السيارة: مدى دقة عكسها للصورة الصحيحة
|
|
|
|
|
|
|
نحو شراكة وطنية متكاملة.. الأمين العام للعتبة الحسينية يبحث مع وكيل وزارة الخارجية آفاق التعاون المؤسسي
|
|
|