
The Circle
 المؤلف:
المرجع الالكتروني للمعلوماتيه
المؤلف:
المرجع الالكتروني للمعلوماتيه
 المصدر:
www.almerja.com
المصدر:
www.almerja.com
 الجزء والصفحة:
...
الجزء والصفحة:
...
 14-3-2017
14-3-2017
 2793
2793
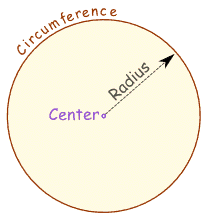
Circle
|
|
A circle is easy to make:
Draw a curve that is "radius" away
from a central point.
And so:
All points are the same distance from the center.
|
|
You Can Draw It Yourself
Put a pin in a board, put a loop of string around it, and insert a pencil into the loop. Keep the string stretched and draw the circle!
|

|
Radius, Diameter and Circumference

The Radius is the distance from the center to the edge.
The Diameter starts at one side of the circle, goes through the center and ends on the other side.
The Circumference is the distance around the edge of the circle.
And here is the really cool thing:
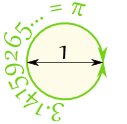
When you divide the circumference by the diameter you get 3.141592654...
which is the number π (Pi)
|
So when the diameter is 1, the circumference is 3.141592654...
|
|
|
We can say:
Circumference = π × Diameter
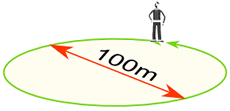
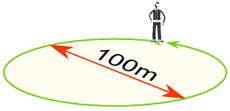
Example: You walk around a circle which has a diameter of 100m, how far have you walked?
|
|
Distance walked = Circumference = π × 100m
= 314m (to the nearest m)
|
Also note that the Diameter is twice the Radius:
Diameter = 2 × Radius
And so this is also true:
Circumference = 2 × π × Radius
Remembering
The length of the words may help you remember:
- Radius is the shortest word
- Diameter is longer (and is 2 × Radius)
- Circumference is the longest (and is π × Diameter)
Definition
|
The circle is a plane shape (two dimensional):
And the definition of a circle is:
|
|

|
The set of all points on a plane that are a fixed distance from a center.
Area
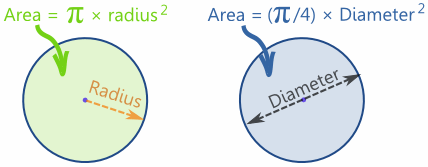
The area of a circle is π times the radius squared, which is written:
A = π r2
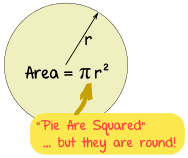
To help you remember think "Pie Are Squared"
(even though pies are usually round)
Or, in relation to Diameter:
A = (π/4) × D2
Example: What is the area of a circle with radius of 1.2 m ?
A = π × r2
A = π × 1.22
A = π × (1.2 × 1.2)
A = 3.14159... × 1.44 = 4.52 (to 2 decimals)
Area Compared to a Square
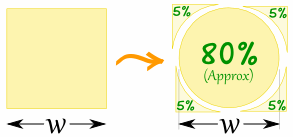
A circle has about 80% of the area of a similar-width square.
The actual value is (π/4) = 0.785398... = 78.5398...%
Names
Because people have studied circles for thousands of years special names have come about.
Nobody wants to say "that line that starts at one side of the circle, goes through the center and ends on the other side" when a word like "Diameter" would do.
So here are the most common special names:
|
|
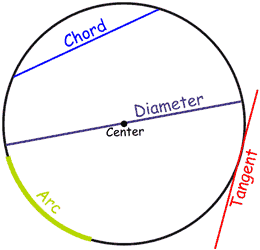
Lines
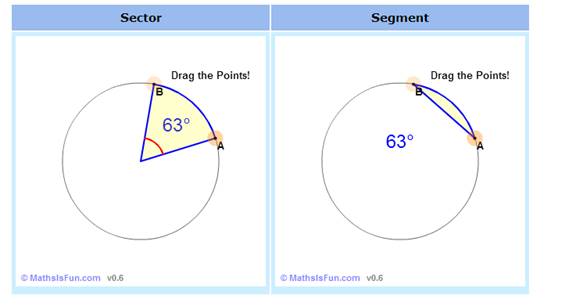
A line that goes from one point to another on the circle's circumference is called a Chord.
If that line passes through the center it is called aDiameter.
A line that "just touches" the circle as it passes by is called a Tangent.
And a part of the circumference is called an Arc.
|
|
Slices
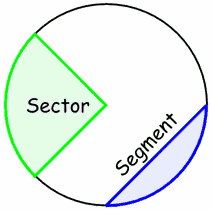
There are two main "slices" of a circle
The "pizza" slice is called a Sector.
And the slice made by a chord is called a Segment.
|

|
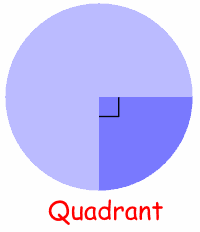
Common Sectors
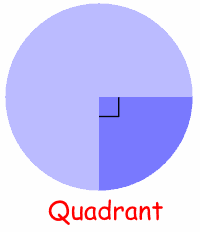
The Quadrant and Semicircle are two special types of Sector:
|
|
Quarter of a circle is called a Quadrant.
Half a circle is called a Semicircle.
|
|

|
Inside and Outside
|
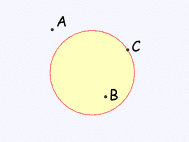
|
A circle has an inside and an outside (of course!). But it also has an "on", because you could be right on the circle.
Example: "A" is outside the circle, "B" is inside the circle and "C" is on the circle.
|
Pi (π)
|
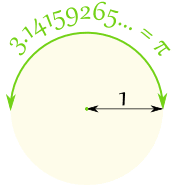
|
|
Draw a circle with a radius of 1.
The distance half way around the edge of the circle
will be 3.14159265... a number known as Pi
|
|
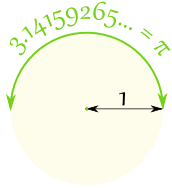
Or you could draw a circle with a diameter of 1.
Then the circumference (the distance all the way
around the edge of the circle) will be Pi
|
|
|
|

|
|
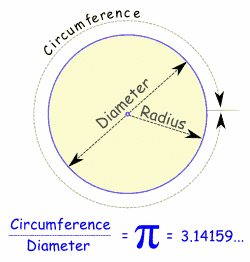
Pi (the symbol is the Greek letter π) is:
The ratio of the Circumference
to the Diameter
of a Circle.
|
|
In other words, if you measure the circumference, and then divide by the diameter of the circle you get the number π
It is approximately equal to:
3.14159265358979323846…
The digits go on and on with no pattern. In fact, π has been calculated to over two quadrillion decimal places and still there is no pattern.
|
|
Example: You walk around a circle which has a diameter of 100m, how far have you walked?
|
|
Distance walked = Circumference = π × 100m = 314.159...m
= 314m (to the nearest m)
|
Approximation
A quick and easy approximation for π is 22/7
22/7 = 3.1428571...
But as you can see, 22/7 is not exactly right. In fact π is not equal to the ratio of any two numbers, which makes it an irrational number.
A better approximation (but stll not exact) is:
355/113 = 3.1415929...
(think "113355", then divide the "355" by the "113")
Remembering
I usually just remember "3.14159", but you can also count the letters of:
"May I have a large container of butter today"
3 1 4 1 5 9 2 6 5
To 100 Decimal Places
Here is π with the first 100 decimal places:
|
3.14159265358979323846264338327950288419716939937510 58209749445923078164062862089986280348253421170679...
|
Circle Sector and Segment
|
Slices
There are two main "slices" of a circle:
- The "pizza" slice is called a Sector.
- And the slice made by a chord is called aSegment.
|
|
Common Sectors
The Quadrant and Semicircle are two special types of Sector:
|
|
Quarter of a circle is called a Quadrant.
Half a circle is called a Semicircle.
|
|

|
|
Area of a Sector
You can work out the Area of a Sector by comparing its angle to the angle of a full circle.
Note: I am using radians for the angles.
|

|
|
This is the reasoning:
- A circle has an angle of 2π and an Area of: πr2
- So a Sector with an angle of θ (instead of 2π) must have an area of: (θ/2π) × πr2
- Which can be simplified to: (θ/2) × r2
Area of Sector = ½ × θ × r2 (when θ is in radians)
Area of Sector = ½ × (θ × π/180) × r2 (when θ is in degrees)
|
|
|
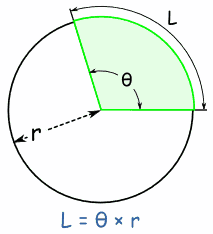
Arc Length
By the same reasoning, the arc length (of a Sector or Segment) is:
L = θ × r (when θ is in radians)
L = (θ × π/180) × r (when θ is in degrees)
|
|
Area of Segment
The Area of a Segment is the area of a sector minus the triangular piece (shown in light blue here).
There is a lengthy reason, but the result is a slight modification of the Sector formula:
|

|
|
Area of Segment = ½ × (θ - sin θ) × r2 (when θ is in radians)
Area of Segment = ½ × ( (θ × π/180) - sin θ) × r2 (when θ is in degrees)
Area of a Circle by Cutting into Sectors
Here is a way to find the formula for the area of a circle:
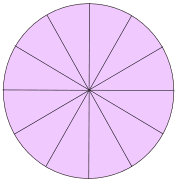
Cut a circle into equal sectors (12 in this example)
Divide just one of the sectors into two equal parts. You now have thirteen sectors – number them 1 to 13:

Rearrange the 13 sectors like this:

Which resembles a rectangle:
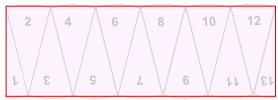
What are the (approximate) height and width of the rectangle?
The height is the circle's radius: just look at sectors 1 and 13 above. When they were in the circle they were "radius" high.
The width (actually one "bumpy" edge) is half of the curved parts along the circle's edge ... in other words it is about half the circumference of the circle.
We know that:
Circumference = 2 × π × radius
And so the width is about:
Half the Circumference = π × radius
And so we have (approximately):

Now we just multply the width by the height to find the area of the rectangle:
Area = (π × radius) × (radius)
= π × radius2
Note: The rectangle and the "bumpy edged shape" made by the sectors are not an exact match.
But we could get a better result if we divided the circle into 25 sectors (23 with an angle of 15° and 2 with an angle of 7.5°).
And the more we divided the circle up, the closer we would get to being exactly right.
Conclusion
Area of Circle = π r2
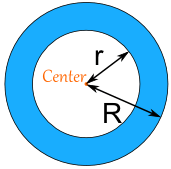
Annulus
|
|
|
An annulus is a flat shape like a ring.
Its edges are two circles that have the same center.
|
Area
Because it is a circle with a circular hole, you can calculate the area by subtracting the area of the "hole" from the big circle's area:
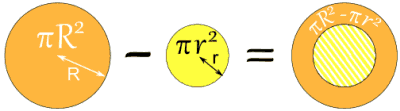
Area = πR2 - πr2
= π( R2 - r2 )

Example: a steel pipe has an outside diameter (OD) of 100mm and an inside diameter (ID) of 80mm, what is the area of the cross section?
Convert diameter to radius for both outside and inside circles:
- R = 100 mm / 2 = 50 mm
- r = 80 mm / 2 = 40 mm
Now calculate area:
Area = π( R2 - r2 )
Area = 3.14159... × ( 502 - 402 )
Area = 3.14159... × ( 2500 - 1600 )
Area = 3.14159... × 900
Area = 2827 mm2 (to nearest mm2)
Circle Theorems
Some interesting things about angles and circles.
Inscribed Angle
First off, a definition:
Inscribed Angle: an angle made from points sitting on the circle's circumference.
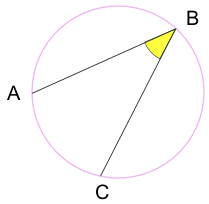
A and C are "end points"
B is the "apex point"
Inscribed Angle Theorems
An inscibed angle a° is half of the central angle 2a°
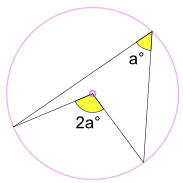
(Called the Angle at the Center Theorem)
And (keeping the endpoints fixed) ...
... the angle a° is always the same, no matter where it is on the circumference:
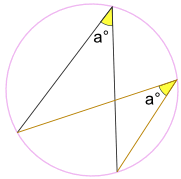
Angle a° is the same.
(Called the Angles Subtended by Same Arc Theorem)
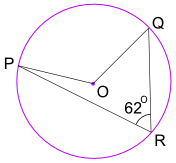
Example: What is the size of Angle POQ? (O is circle's center)
|
|
Angle POQ = 2 × Angle PRQ = 2 × 62° = 124°
|
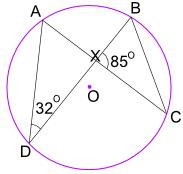
Example: What is the size of Angle CBX?
|
|
Angle ADB = 32° equals Angle ACB.
And Angle ACB equals Angle XCB.
So in triangle BXC we know Angle BXC = 85°, and Angle XCB = 32°
Now use angles of a triangle add to 180° :
Angle CBX + Angle BXC + Angle XCB = 180°
Angle CBX + 85° + 32° = 180°
Angle CBX = 63°
|
Angle in a Semicircle
An angle inscribed in a semicircle is always a right angle:
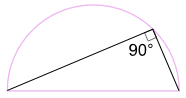
(The end points are either end of a circle's diameter,
the apex point can be anywhere on the circumference.)
|
Why? Because:
The inscibed angle 90° is half of the central angle 180°
(Using "Angle at the Center Theorem" above)
|

|
|
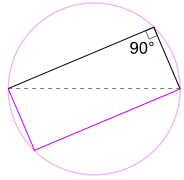
|
|
Another Good Reason Why It Works
We could also rotate the shape around 180° to make a rectangle!
It is a rectangle, because all sides are parallel, and both diagonals are equal.
And so its internal angles are all right angles (90°).
|
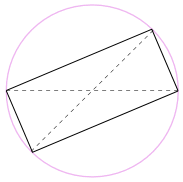
|
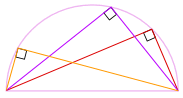
So there we go! No matter where that angle is
on the circumference, it is always 90°
Example: What is the size of Angle BAC?
|
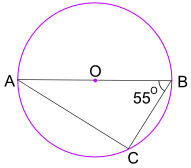
|
The Angle in the Semicircle Theorem tells us that Angle ACB = 90°
Now use angles of a triangle add to 180° to find Angle BAC:
Angle BAC + 55° + 90° = 180°
Angle BAC = 35°
|
Cyclic Quadrilateral
|
A "Cyclic" Quadrilateral has every vertex on a circle's circumference:
|
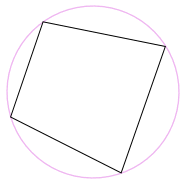
|
|
A Cyclic Quadrilateral's opposite angles add to 180°:
- a + c = 180°
- b + d = 180°
|

|
Example: What is the size of Angle WXY?
|
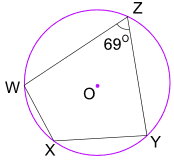
|
Opposite angles of a cyclic quadrilateral add to 180°
Angle WZY + Angle WXY = 180°
69° + Angle WXY = 180°
Angle WXY = 111°
|
|

|
Tangent Angle
A tangent is a line that just touches a circle at one point.
It always forms a right angle with the circle's radius.
|
|
 الاكثر قراءة في الهندسة المستوية
الاكثر قراءة في الهندسة المستوية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة


