
تاريخ الفيزياء

علماء الفيزياء


الفيزياء الكلاسيكية

الميكانيك

الديناميكا الحرارية


الكهربائية والمغناطيسية

الكهربائية

المغناطيسية

الكهرومغناطيسية


علم البصريات

تاريخ علم البصريات

الضوء

مواضيع عامة في علم البصريات

الصوت


الفيزياء الحديثة


النظرية النسبية

النظرية النسبية الخاصة

النظرية النسبية العامة

مواضيع عامة في النظرية النسبية

ميكانيكا الكم

الفيزياء الذرية

الفيزياء الجزيئية


الفيزياء النووية

مواضيع عامة في الفيزياء النووية

النشاط الاشعاعي


فيزياء الحالة الصلبة

الموصلات

أشباه الموصلات

العوازل

مواضيع عامة في الفيزياء الصلبة

فيزياء الجوامد


الليزر

أنواع الليزر

بعض تطبيقات الليزر

مواضيع عامة في الليزر


علم الفلك

تاريخ وعلماء علم الفلك

الثقوب السوداء


المجموعة الشمسية

الشمس

كوكب عطارد

كوكب الزهرة

كوكب الأرض

كوكب المريخ

كوكب المشتري

كوكب زحل

كوكب أورانوس

كوكب نبتون

كوكب بلوتو

القمر

كواكب ومواضيع اخرى

مواضيع عامة في علم الفلك

النجوم

البلازما

الألكترونيات

خواص المادة


الطاقة البديلة

الطاقة الشمسية

مواضيع عامة في الطاقة البديلة

المد والجزر

فيزياء الجسيمات


الفيزياء والعلوم الأخرى

الفيزياء الكيميائية

الفيزياء الرياضية

الفيزياء الحيوية

الفيزياء العامة


مواضيع عامة في الفيزياء

تجارب فيزيائية

مصطلحات وتعاريف فيزيائية

وحدات القياس الفيزيائية

طرائف الفيزياء

مواضيع اخرى
Niels Bohr
المؤلف:
William H. Cropper
المصدر:
Great Physicists
الجزء والصفحة:
7-12-2015
4983
Niels Bohr

Hail to Niels Bohr
Quantum theory was not an overnight success. Its reception during the first decade of its history was hesitant, and its practitioners were scarce. By 1910, the Planck postulates were more or less recognized, but they had been applied mostly to problems concerning radiation and the solid state, and hardly at all in the realm of atoms and molecules. There had been no movement toward the formulation of a general quantum physics.
In the summer of 1913, there appeared in the Philosophical Magazine the first of a series of papers that began to turn the tide. The author was Niels Bohr, a twenty eight year old Danish physicist with a rare personality. Bohr's theory described the behavior of atoms, particularly hydrogen atoms, with a carefully concocted mixture of the Planck postulates and the classical mechanics of Kepler and Newton. Bohr applied the theory, with spectacular success, to the beautiful spectral patterns emitted by hydrogen gas when it is excited electrically. (The physical apparatus is similar to that used in neon lighting.) This was, to the physicists of the time, an incredible achievement. Spectroscopists, the experimentalists who study the regularities of light wavelengths (spectra) emitted by atoms and molecules, had done their work so long without benefit of a theory that they had despaired of ever finding one. Bohr's papers brought new hope for spectroscopy, and for quantum theory as well.
To some extent, Bohr's role in this was good fortune. Quantum theory loomed large enough in 1913 that its value to atomic physics could not have been missed much longer. Even so, Bohr's task was no simple exercise. It took skill and intuitive sense in large measure to devise a workable mixture of classical and quantum physics. Einstein remarked that he had had similar ideas, “but had no pluck to develop them.” To Einstein, Bohr's sensitive application of the “insecure and contradictory foundation” supplied by quantum theory to atomic problems was a marvel, “the highest form of musicality in the sphere of thought.”
Bohr did more than create theoretical masterpieces. He also built, almost single-handedly, a great school of theoretical and experimental physics in Copenhagen. The Bohr Institute (officially, the University Institute of Theoretical Physics) was inaugurated on March 3, 1921, and it quickly attracted an extraordinary collection of young German, English, Russian, Dutch, Hungarian, Indian, Swedish, and American physicists. Bohr offered them a place to live and work when academic positions were scarce and theoretical physicists, like artists, were poor.
Activities at the institute were not always what one would expect from a learned gathering: Ping-Pong (played in the library), girl watching, and cowboy movies were favorite pastimes. But a lot of strenuous and brilliant work was done in this seemingly easy atmosphere. Wolfgang Pauli, Werner Heisenberg, Paul Dirac, Lev Landau, Felix Bloch, Edward Teller, George Gamow, and Walter Heitler were all visitors at the Bohr Institute: their names and accomplishments tell a large part of what happened in quantum physics during the crucial years of the 1920s and 1930s. Robert Oppenheimer writes of this period and Bohr's indispensable role in it: “It was a heroic time. It was not the doing of one man; it involved the collaboration of scores of scientists from many different lands, though from first to last the deep creative and critical spirit of Niels Bohr guided, restrained, and finally transmuted the enterprise.”
Bohr had few of the characteristics expected of a man of such influence. His lectures were likely to be “neither acoustically nor otherwise completely understandable.” Despite a prodigiously thorough effort, his papers and books were frequently repetitious and dense. Anecdotes are told of his unembarrassed questions about matters of common knowledge. His stock of jokes at any time was limited to about six. Yet his personality was forceful and penetrating. Bohr spoke with a gentle directness and sincerity that impressed students, colleagues, and presidents alike. As Leon Rosenfeld, one of Bohr's collaborators, remarked of the stream of visitors to Copenhagen: “They come to the scientist, but they find the man, in the full sense of the word.”
Bohr's generosity was repaid in a remarkable way. Apparently, Bohr could not think creatively without human company. Throughout his career he conceived, shaped, and finished his scientific ideas in conversations with small, critical audiences, usually selected from those at hand at the institute. So attuned were his thoughts to a living presence that no part of the creative process could proceed without a human sounding board. Papers and lectures were written in restless, erratic dictating sessions that were sometimes monologues. One of Bohr's assistants, Oskar Klein, gives us a glimpse of Bohr refining a lecture: “With some writing paper and a pencil in front of me I was placed at a table around which Bohr wandered, alternately dictating in English and explaining in Danish, while I tried to get the English on paper. Sometimes there were long interruptions either for pondering what was to follow, or because Bohr had thought about something outside the theme he had to tell me about. . . . Often, also, work was interrupted by short running trips or cycling to the shore together with the family for bathing.”
Bohr's energy and tenacity in the perfecting of a paper seemed almost superhuman. Every word, sentence, concept, and equation had to be reviewed and revised. After five or six drafts (the last one probably on a printer's page proofs), with no end in sight, Bohr would retire to some quiet corner of the institute, accompanied by the indispensable amanuensis, and the struggle would continue. Finally, unbelievably, Bohr would be satisfied. Wolfgang Pauli, who was often invited to Copenhagen for his services as a valuable, but not always sympathetic, critic, responded to one invitation with: “If the last proof is sent away, then I will come.”
With his relentless insistence on clarity, and his vast gift for coaxing criticism from others in marathon conversations, Bohr managed to penetrate some of the most difficult problems in quantum physics, including those of a conceptual and philosophical nature. His arguments had daring and a thoroughness that was unassailable. His interpretation of quantum theory, particularly its paradoxes, contrasted with and often contradicted Einstein's viewpoints. Beginning in 1927 at a Solvay conference, and continuing for twenty years, Bohr and Einstein carried on a friendly debate concerning the meaning of quantum physics. Einstein could never accept Bohr's conclusion that the microworld of atoms and molecules is ultimately indeterminate, and did his best to break Bohr's defenses. But Bohr always had an answer to Einstein's criticisms, and his arguments prevailed.
Like some of the other physicists whose stories are told in these chapters, Bohr was blessed with an ideal marriage. Margrethe Norlund Bohr was a lovely, intelligent woman, and a fine manager and hostess. The Bohrs had six sons two of them did not survive childhood, and the eldest, Christian, was drowned in a sailing accident and after 1932 they lived in the Carlsberg “House of Honor” for Denmark's first citizen. Rosenfeld tells us about Margrethe's vital place in this complicated existence: “Margrethe's role was not an easy one. Bohr was of a sensitive nature, and constantly needed the stimulus of sympathy and understanding. When children came . . . Bohr took very seriously his duty as paterfamilias. His wife adapted herself without apparent effort to the part of hostess, and evenings at the Bohr home were distinguished by warm cordiality and exhilarating conversation.”
Bohr won a Nobel Prize. He advised Presidents Roosevelt and Truman and Prime Minister Churchill, and became known in every corner of the world of physics. His life, personality, and aspirations became legendary. Only Einstein and Marie Curie, among scientists of the twentieth century, reached positions of such eminence. But before all else Bohr's place was with the carefree, yet devoted and gifted, members of the institute, taking and using their criticism, and enjoying their spoofing:
Hail to Niels Bohr from the worshipful nations!
You are the master by whom we are led,
Awed by your cryptic and proud affirmations,
Each of us, driven half out of his head,
—Yet remains true to you,
—Wouldn't say boo to you,
Swallows your theories from alpha to zed,
—Even if (Drink to him,
—Tankards must clink to him!)
None of us fathoms a word you have said!
The Bohr-Rutherford Atom
No doubt it is significant that Niels Bohr began his career as a practicing physicist in a laboratory “full of characters from all parts of the world working with joy under the energetic and inspiring influence of the ‘great man.’ ” The “great man” in the laboratory of Bohr’s apprenticeship known as “Papa” or “the Prof” to the inhabitants was Ernest Rutherford, who gave us the concept of the atomic nucleus. Rutherford, a New Zealander transplanted to England, presided over nuclear physics during its most creative and, one might say, in view of later developments, its most innocent and happiest years.
The atomic nucleus is a particle about 10-13 centimeter in diameter that carries a positive charge and most of the atom's mass. It is surrounded by a balancing negative charge to a total atomic radius of about 10-8 centimeter. In other words, the atom is a hundred thousand times bigger than its nucleus. In dimension, the nucleus in the atom is like “a fly in a cathedral,” according to Ernest Lawrence, who helped build nuclear physics on Rutherford's foundations. (But this is a fantastically heavy fly; it weighs several thousand times more than the cathedral.)
Rutherford drew his atomic model from the evidence of a monumental series of experiments reported in 1913 by Hans Geiger later of “Geiger counter” fame, and one of the most gifted in Rutherford’s group of experimentalists and Ernest Marsden, a young student. Geiger and Marsden observed the scattering of alpha particles (helium ions produced by radioactive materials) by thin metallic foils. Most of the alpha particles passed through the thin foils with little or no deflection, as expected, but the paths of a few were drastically altered, as if they had collided with something very small and very massive in the metallic foil the atomic nuclei of Rutherford's model.
Bohr joined Rutherford and his “tribe” at the University of Manchester in 1912, just as the nuclear atom was beginning to emerge. (Bohr had also spent a brief time working for J. J. Thomson at the Cavendish Laboratory, Cambridge. Bohr's rudimentary English, and his not always tactful insistence on critical discussions, seem to have alienated Thomson, who was inclined to be distant on scientific matters anyway.) The Manchester laboratory and its chief were much to Bohr's liking: “Rutherford is a man you can rely on; he comes regularly and enquires how things are going and talks about the smallest details Rutherford is such an outstanding man and really interested in the work of all the people around him,” Bohr wrote to his brother Harald. Although Bohr showed signs of being a theorist, a breed of physicist not always welcome in Rutherford territory, his talent, obvious sincerity, and lack of pretension and previous fame as a soccer player seem to have impressed Rutherford immediately: “Bohr's different. He's a football player!”
Bohr was fascinated by the nuclear model of the atom, not only by its impressive successes in accounting for the Geiger-Marsden foil experiments, but also for its most conspicuous failure. It was obvious that no simple version of the nuclear atom could have the infinite stability atoms normally have. For example, it seemed reasonable to picture the negative electricity surrounding the nucleus as electrons moving in planet like orbits around the nucleus. But electrons circulating in orbits should have behaved like the electrical charge circulating or oscillating in a radio antenna, and therefore an atom containing orbital electrons should have imitated the antenna and continuously radiated energy. Sooner or later, the electrons would have collapsed into the nucleus, thus destroying the atom.
Such was the unnatural fate predicted for Rutherford's nuclear atom by the classical theory of electrodynamics. But this problem of atoms collapsing on themselves was no challenge to the nucleus itself: the Geiger-Marsden foil experiments left no doubt that Rutherford’s picture of the nucleus was correct. The mystery to be solved, for which the Geiger-Marsden data offered no clues, concerned the status of the surrounding electrons.
To Bohr and several others who had thought about the problem before him it was clear that, however the electrons disposed themselves in atoms, they had to obey physical laws that were in some sense radically different from the laws of radio antennas and other objects from the macro world. Bohr noted in his first paper on atomic structure, On the Constitution of Atoms and Molecules, the “general acknowledgment of the inadequacies of the classical electrodynamics in describing the behavior of systems of atomic size.”
But why allow the classical theory, which had been applied and tested only in the macroscopic realm, to create a mystery concerning non-radiating atomic electrons when there was no reason to believe that the classical theory applied? Why adhere to the classical theory and assume that electrons in atoms should radiate energy? The greatest accomplishment of Bohr's theory was that it introduced the assumption that electrons have “waiting places” or “stationary states” in which they do not radiate, and have constant, stable energies. This postulate, which Bohr restated and reexamined throughout five lengthy papers published between 1913 and 1915, finally emerged as this statement: “An atomic system possesses a number of states in which no emission of energy takes place, even if the particles are in motion relative to each other, and such an emission is to be expected in ordinary electrodynamics. The states are denoted as ‘stationary’ states of the system under consideration.”
How could electrons be described as they moved around in an atom under the restriction of Bohr’s stationary states? Bohr, like Planck, felt that classical physics should be retained wherever possible. Although classical electrodynamics created the difficulty that orbiting electrons should radiate energy, there appeared to be no reason why the laws of classical mechanics, which governed the orbital motion of planets, should be rejected. So Bohr pictured electrons in stationary states moving in circular or elliptical orbits prescribed by the mechanics of Newton and Kepler. On the other hand, when an electron changed from one stationary state to another it did so in a discontinuous “jump,” not governed by classical mechanics. Bohr stated a second postulate: “The dynamical equilibrium of the systems in the stationary states is governed by the ordinary laws of mechanics, while those laws do not hold for the transition from one state to another.”
Bohr found it expedient to characterize an electron occupying one of the stationary states by specifying the electron's “binding energy” E in the orbit the energy required to remove the electron from the atom that holds it and its frequency of rotation ⍵, the number of orbital circuits completed per second. He derived the classical equation
E3 = R⍵2, (1)
relating E and ⍵, with R a composite of several constants whose values had been accurately measured.
In the version of his theory we are viewing, Bohr deftly committed his theory to the quantum viewpoint by introducing a second energy-frequency connection by way of “extra-mechanical fiat,” in the apt phrase of the science historians John Heilbron and Thomas Kuhn. Bohr's second equation was
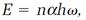 (2)
(2)
with E, as before, an electron's binding energy, n a positive integer called a “quantum number,” and a proportionality factor to be evaluated at a later stage. According to this equation, with n = 1,2,3, . . . , an atomic electron can have the “quantized” energy values
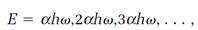
and no others. Bohr was asserting here a formal analogy with Planck's rule that atoms in the walls of a blackbody oven can have only the quantized energies
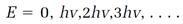
The atom as a dynamic quantum-emitting entity took shape with a second and radically different Bohr frequency rule. This one pictured an atom jumping from one stationary state of higher energy E1 to another of lower energy E2, with an energy change E1 - E2, and emitting radiation whose frequency v is connected with the energy change by Planck's constant h:
E1 - E2 = hv. (3)
The two frequencies, ⍵ and v, the first representing an electron's rotation frequency and the second a radiation frequency, were separated in Bohr's theory. This was a drastic departure from the classical theory, which would have pictured an orbiting electron irradiating at a frequency equal to its rotation frequency.
Although the rotation frequency ⍵ and the radiation frequency v were generally separated in Bohr's theory, the theory did allow for the exaggerated case in which an atom was so stretched in size that it became a classical object, behaved like an ordinary radio antenna, and radiated frequencies equivalent to the electron rotation frequencies. In this special case, ⍵ = v, and the quantum-theoretical laws merged into the classical laws.
The theoretical device of connecting the quantum and classical realms making them “correspond,” as Bohr put it was one of Bohr's most valuable contributions, and one in which he took particular pride. This “correspondence principle” was used by Bohr throughout much of his work on quantum theory, and it finally became a cornerstone in the quantum mechanics created by Werner Heisenberg.
When equations (1) and (2) are combined by eliminating ⍵, a simple equation results relating the electron binding energy E and the quantum number n,
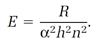
If this equation is written twice for two states whose energies are E1 and E2, and quantum numbers are n1 and n2,
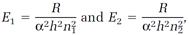
and these two results are substituted in equation (3), we have
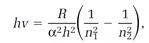
or
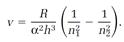 (4)
(4)
By invoking his correspondence argument, Bohr proved that the constant α had the value 1⁄2, and this put his frequency equation (4) in its final form:
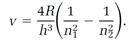 (5)
(5)
Balmer’s Formula
Bohr did not pick these equations out of theoretical thin air. He was guided by the observed patterns in the radiation spectra emitted by elemental substances, particularly atomic hydrogen. If the components of the hydrogen emission spectrum are sorted out by an instrument called a spectroscope, the observed frequencies fall in regular series. One of the hydrogen spectral series had been discovered thirty years before Bohr's work by Johann Balmer, a Swiss schoolteacher accomplished in the art of distilling precise numerical formulas from complex physical data. Balmer discovered that the visible lines in the hydrogen emission spectrum had frequencies that fit a formula such as
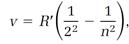
in which R' represents a parameter whose value is determined by the spectral data, and n here is any integer larger than 2: n = 3,4,. . . . Balmer appreciated that his formula might imply a more general formula such as
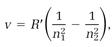 (6)
(6)
with n1 given the value 2 in his spectral series, but possibly other values in other series.
Balmer's formula, and a variety of other empirical rules of spectroscopy contributed particularly by the Swedish spectroscopist Johannes Rydberg (whose version of Balmer's formula is quoted above), had been known for years without arousing any suspicion that they contained simple clues to atomic structure. Bohr once remarked that the Balmer-Rydberg formula and others like it were regarded in the same light “as the lovely patterns in the wings of butterflies; their beauty
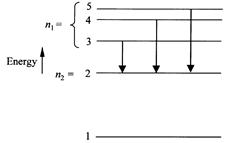
Figure 1.1. An energy-level diagram showing emission transitions for three of the lines in the hydrogen Balmer series.
can be admired, but they are not supposed to reveal any fundamental biological laws.”
The “lovely patterns” of the hydrogen emission spectrum were the substance of Bohr's theory. His arguments were pointed, and sometimes forced so that his derived equations would match the observed spectral patterns. Bohr's immediate theoretical aim was accomplished when he derived equation (5), which imitated the Balmer-Rydberg equation (6). The final and crucial test of the theory was passed when the theoretically derived constant 4R /h3in equation (5) was compared with its empirical counterpart R' in equation (6). Calculation of the former from the known fundamental constants (the electronic charge e and mass m and Planck's constant h were involved) came to within a few percent of the measured values of the latter. This was an impressive achievement. Not often in the history of science has a theoretician had such success in bringing theory together with experiment without benefit of those handy numerical devices disrespectful students call “fudge factors.”
Bohr's equation (5) is displayed in figure 1.1 as an energy-level diagram. Each horizontal line represents the energy of a stationary state and is labeled with a value of a quantum number, n1 or n2. The downward-jumping atomic transitions that produce three of the emitted frequencies in the Balmer series are indicated with arrows.
 الاكثر قراءة في علماء الفيزياء
الاكثر قراءة في علماء الفيزياء
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















