Trigonometric functions of any angle

Unit circle. Counting of angles in a unit circle. 
Negative and positive angles. Quarters of a unit circle. 
Sine and cosine lines. Sine. Cosine. Signs of sine and 
cosine in different quarters of a unit circle. Tangent 
and cotangent lines. Tangent. Cotangent. Signs of 
tangent and cotangent in different quarters of a unit 
circle. Secant and cosecant.

To build all trigonometry, laws of which would be valid for any angles ( not only for acute angles, but also for obtuse, positive and negative angles), it is necessary to consider so called a unit circle, that is a circle with a radius, equal to 1 ( Fig.3 ).

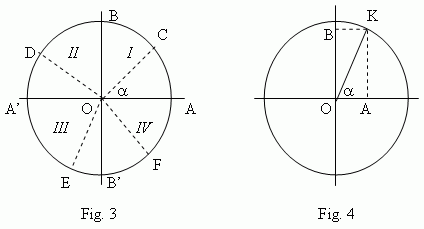
Let draw two diameters: a horizontal AA’ and a vertical BB’. We count angles off a point A (starting point). Negative angles are counted in a clockwise, positive in an opposite direction. A movable radius OC forms angle α with an immovable radius OA. It can be placed in the 1-st quarter ( COA ), in the 2-nd quarter ( DOA ), in the 3-rd quarter ( EOA ) or in the 4-th quarter ( FOA ). Considering OA  and  OB  as positive directions and  OA’  and  OB’ as negative ones, we determine trigonometric functions of angles as follows.

A sine line of an angle  ( Fig.4 ) is a vertical diameter of a unit circle, a cosine line of an angle α - a horizontal diameter of a unit circle. A sine of an angle α ( Fig.4 ) is the segment OB of a sine line, that is a projection of a movable radius OK to a sine line; acosine of an angle  - the segment OA of a cosine line, that is a projection of a movable radius OK to a cosine line.

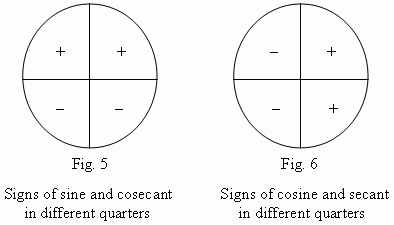
Signs of sine and cosine in different quarters of a unit circle are shown on Fig.5 and Fig.6.

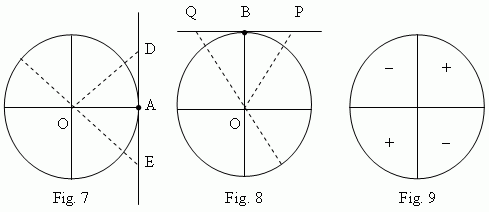
A tangent line ( Fig.7 ) is a tangent, drawn to a unit circle through the point A of a horizontal diameter.
A cotangent line ( Fig.8 ) is a tangent, drawn to a unit circle through the point B of a vertical diameter.
A tangent is a segment of a tangent line between the tangency point A and an intersection point ( D, E, etc., Fig.7 ) of a tangent line and a radius line. 
A cotangent is a segment of a cotangent line between the tangency point B and an intersection point ( P, Q, etc., Fig.8 ) of a cotangent line and a radius line.

Signs of tangent and cotangent in different quarters of a unit circle see on Fig.9.

Secant and cosecant are determined as reciprocal values of cosine and sine correspondingly.

اخر الاخبار

اخبار العتبة العباسية المقدسة

قسم الشؤون الطبية يقدم الخدمات الصحية والعلاجية لزائري مدينة سامراء

العتبة العباسية المقدسة تقدم خدماتها لزائري مرقد الإمامين العسكريين (عليهما السلام) في سامراء

المجمَع العلمي ووفد وزارة التربية يبحثان سبل التعاون المشترك

قسم الشؤون الفكرية يصدر العدد 192 من مجلة الرياحين للأطفال

المزيد

الآخبار الصحية

كنز غذائي على طبقك.. ماذا تفعل السبانخ بجسمك؟

ما لا يتوقعه أحد.. "سر غذائي" في الجبن الدسم

لتحسين الهضم والنوم.. هذا أفضل وقت لتناول العشاء

10 طرق بسيطة وغير مألوفة لحرق المزيد من السعرات يوميا

المزيد

الآخبار التكنلوجية

سابقة بعالم الطيران.. طائرة تقرر الهبوط بنفسها بعد ظرف طارئ

"الإنفلونزا الخارقة" تكتسح دول العالم.. وتثير قلقا كبيرا

مرحلة ما قبل الأعراض.. دواء جديد يستهدف "شرارة" الزهايمر

إزالة ثاني أكسيد الكربون من الغلاف الجوي.. ما دور المحيطات والبحار؟

المزيد

مواضيع ذات صلة

Trigonometric inequalities

Trigonometric functions of an acute angle

Trigonometric equations. Main methods for solving

Transforming of trigonometric expressions to product

Transforming of degree measure to radian one and back

Systems of simultaneous trigonometric equations

Some important correlations

Solving of right-angled triangles

Relations between trigonometric functions of the same angle

Reduction formulas

Radian and degree measures of angles

Problems: Trigonometric transformations