To introduce the concept of conditional probability, consider the following example. An urn contains 10 large marbles of which 6 are white and 4 are black, and 10 small marbles of which 3 are white and 7 are black. Let the event that a marble drawn at random is white be denoted by W, and that it is large be denoted by L.

It is clear that P(W) = 9/20  Suppose that a marble is drawn and it is large. The probability that it is also white is 5. The reason these values are different is that the first is based on a sample space with 20 points of which 9 correspond to white marbles, and the second is based on a sample space of 10 points of which 6 correspond to white marbles. The second probability will be referred to as the probability that the ball is white knowing it is large, and will be denoted by P(WIL). This intuitive ex- ample suggests the following definition.

DEFINITION. Let X be an event in an arbitrary sample space with nonzero probability, and let Y be any event in the same sample space. The conditional probability that Y occurs knowing that X has occurred is defined by

If both numerator and denominator of P(YIX) are divided by the number of points in the sample space, this formula becomes

Still another useful form for the formula is obtained by multiplying both sides of (2) by P(X). This gives

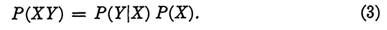
A final formula which is often useful comes as an application of Theorem 2 of (The number of elements in a set). Let Y be any event, and let X₁, X ₂, ... , X_m be events

representing disjoint sets (mutually exclusive) and such that

                                           X₁+X₂+...+X_m= 1,

the entire sample space. Then it follows from Theorem 2 of (The number of elements in a set).

            that P(Y) = P(YX₁) + P(YX₂) + + P(YX_m), and applying (3)  above, we obtain

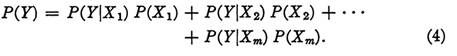
Example 1. Find the probability that a card dealt from a bridge deck is an ace, if it is known to be either an ace or a face card (that is, A, K, Q, or J).

Solution. Let Y denote the event that the card is an ace, and X the event that it is either an ace or a face card. The definition gives

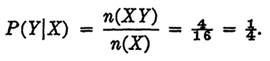
EXAMPLE 2. A certain group of students is two thirds males and one third females. Of these, one tenth of the males are color-blind. What is the probability that a student selected at random will be a color-blind male?

Solution. Let Y be the event that the student is color-blind, and X the event that he is male. Equation (3) gives

                         P(XY) = P(YI X) P(X) =(1/10) (2/3)=1/15

EXAMPLE 3. In a factory, three operators A, B, and C alternate in shifts in operating a certain machine. Records show that the number of parts produced by A, B, and C, respectively, are in the ratio 4:5:6. Of the parts produced,  1% of A's, 2% of B's, and 3% of C's are defective. What is the probability that a part drawn at random from the output of their machine will be defective?

Solution. Let D represent the event that the part is defective, and let A, B,  and C represent the events that the part is produced by operator A, B, or C,  respectively. Using Eq. (4), we find that

P(D) = P(DIA) P(A) + P(DIB) P(B) + P(DIC) P(C)

= 0.01 (4/15)+0.02(1/3)+0.03(2/5)

= 0.0213,   approximately.

مواضيع ذات صلة

Zero Polynomial

Kronecker,s Polynomial Theorem

THE ALGEBRA OF SETS-The number of elements in a set

THE ALGEBRA OF SETS-Solution of equations

THE ALGEBRA OF SETS-Conditional equations

THE ALGEBRA OF SETS-Properties of set inclusion

THE ALGEBRA OF SETS- Expanding, factoring, and simplifying

THE ALGEBRA OF SETS-Fundamental laws

THE ALGEBRA OF SETS-Venn diagrams

THE ALGEBRA OF SETS-The combination of sets

THE ALGEBRA OF SETS-Element and set

THE ALGEBRA OF SETS-Introduction