

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Traveling Salesman Problem
المؤلف:
Applegate, D.; Bixby, R.; Chvatal, V.; and Cook, W.
المصدر:
"Finding Cuts in the TSP (a Preliminary Report)." Technical Report 95-05, DIMACS. Piscataway NJ: Rutgers University, 1995.
الجزء والصفحة:
...
21-12-2021
3062
Traveling Salesman Problem
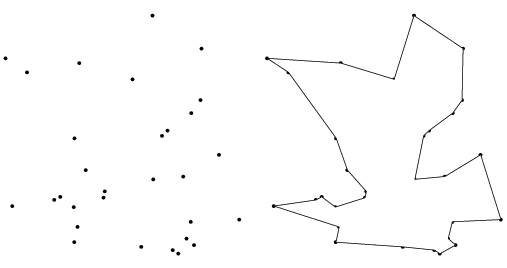
The traveling salesman problem is a problem in graph theory requiring the most efficient (i.e., least total distance) Hamiltonian cycle a salesman can take through each of 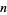 cities. No general method of solution is known, and the problem is NP-hard.
cities. No general method of solution is known, and the problem is NP-hard.
The Wolfram Language command FindShortestTour[g] attempts to find a shortest tour, which is a Hamiltonian cycle (with initial vertex repeated at the end) for a Hamiltonian graph  if it returns a list with first element equal to the vertex count of
if it returns a list with first element equal to the vertex count of  .
.
The traveling salesman problem is mentioned by the character Larry Fleinhardt in the Season 2 episode "Rampage" (2006) of the television crime drama NUMB3RS.
REFERENCES:
Applegate, D.; Bixby, R.; Chvatal, V.; and Cook, W. "Finding Cuts in the TSP (a Preliminary Report)." Technical Report 95-05, DIMACS. Piscataway NJ: Rutgers University, 1995.
Applegate, D.; Bixby, R.; Chvatal, V.; and Cook, W. "Solving Traveling Salesman Problems." http://www.tsp.gatech.edu/.
Hoffman, P. The Man Who Loved Only Numbers: The Story of Paul Erdős and the Search for Mathematical Truth. New York: Hyperion, pp. 168-169, 1998.
Kruskal, J. B. "On the Shortest Spanning Subtree of a Graph and the Traveling Salesman Problem." Proc. Amer. Math. Soc. 7, 48-50, 1956.
Lawler, E.; Lenstra, J.; Rinnooy Kan, A.; and Shmoys, D. The Traveling Salesman Problem: A Guided Tour of Combinatorial Optimization. New York: Wiley, 1985.
Lin, S. "Computer Solutions of the Traveling Salesman Problem." Bell System Tech. J. 44, 2245-2269, 1965.
Platzman, L. K. and Bartholdi, J. J. "Spacefilling Curves and the Planar Travelling Salesman Problem." J. Assoc. Comput. Mach. 46, 719-737, 1989.
Reinelt, G. "TSPLIB--A Traveling Salesman Problem Library." ORSA J. Comput. 3, 376-384, 1991.
Rosenkrantz, D. J.; Stearns, R. E.; and Lewis, P. M. "An Analysis of Several Heuristics for the Traveling Salesman Problem." SIAM J. Comput. 6, 563-581, 1977.
Skiena, S. "Traveling Salesman Tours." §5.3.5 in Implementing Discrete Mathematics: Combinatorics and Graph Theory with Mathematica. Reading, MA: Addison-Wesley, pp. 199-202, 1990.
Skiena, S. S. "Traveling Salesman Problem." §8.5.4 in The Algorithm Design Manual. New York: Springer-Verlag, pp. 319-322, 1997.
Steinhaus, H. Mathematical Snapshots, 3rd ed. New York: Dover, pp. 120-121, 1999.
 الاكثر قراءة في الرياضيات التطبيقية
الاكثر قراءة في الرياضيات التطبيقية
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة
قسم الشؤون الفكرية يصدر كتاباً يوثق تاريخ السدانة في العتبة العباسية المقدسة "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)


















