Can the previously discussed quantum effects of black holes be observed? As has already been mentioned, black holes formed by stellar collapse are much too heavy to exhibit quantum behaviour. To form smaller black holes one needs higher densities which can only occur under the extreme situations of the early Universe. Such primordial black holes can originate in the radiation-dominated phase during which no stars or other objects can be formed.

Consider for simplicity a spherically symmetric region with radius R and density ρ = ρ_c + δ_ρ embedded in a flat Universe with the critical density ρ_c, cf Carr (1985). For spherical symmetry the inner region is not affected by matter in the surrounding part of the Universe, so it will behave like a closed Friedmann Universe (since its density is overcritical), i.e. the expansion of this region will come to a halt at some stage, followed by a collapse. In order to reach a complete collapse, the (absolute value of the) potential energy, V , at the time of maximal expansion has to exceed the inner energy, U, given by the pressure p, that is,

 (1.1)

If the equation of state reads as p = wR (w = 1/3 for radiation dominance), this gives

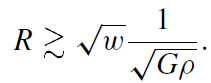 (1.2)

The lower bound for R is thus just given by the Jeans length. An upper bound also exists. The reason is that R must be smaller than the curvature radius (given by 1/√Gρ) of the over dense region at the moment of collapse. Otherwise the region would contain a compact three-sphere which is topologically disconnected from the rest of the Universe. This case would not then lead to a black hole within our Universe. Using ρ ∼ ρ_c ∼ H²/G, where H denotes the Hubble parameter of the background flat Universe, one has the condition

 (1.3)

evaluated at the time of collapse, for the formation of a black hole. This relation can also be rewritten as a condition referring to any initial time of interest (Carr 1985). In particular, one is often interested in the time where the fluctuation enters the horizon in the radiation-dominated Universe. This is illustrated in figure 1.1, where the presence of a possible inflationary phase at earlier times is also shown.

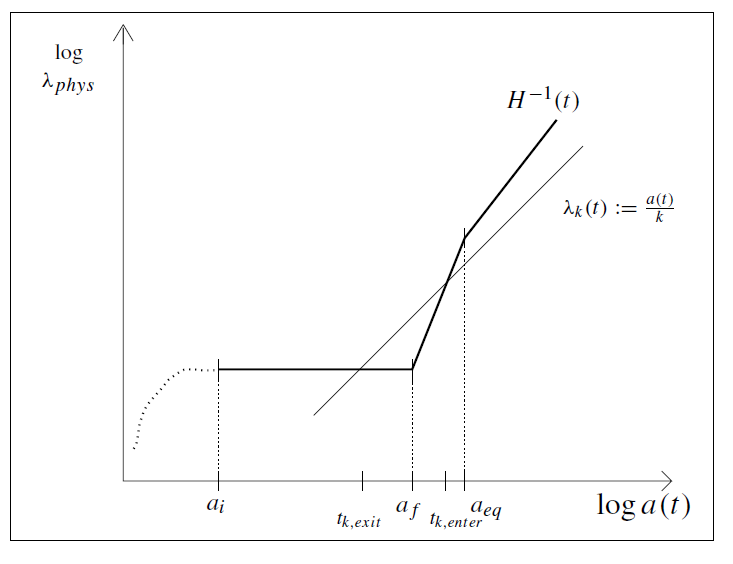
Figure 1.1. Time development of a physical scale λ(t) and the Hubble horizon H⁻¹(t). During an inflationary phase H⁻¹(t ) remains approximately constant. After the end of inflation (a f ) the horizon H⁻¹(t ) increases faster than any scale. Therefore λk enters the horizon again at t_k,enter in the radiation- (or matter-) dominated phase.

At horizon entry one gets, denoting δ ≡ δρ/ρc,

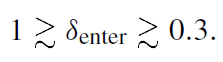 (1.4)

This is, however, only a rough estimate. Numerical calculations give instead the bigger value of δ_min ≈ 0.7 (Niemeyer and Jedamzik 1999). Taking from (1.3) R ≈ √wH⁻¹, one gets for the initial mass of a primordial black hole (PBH)

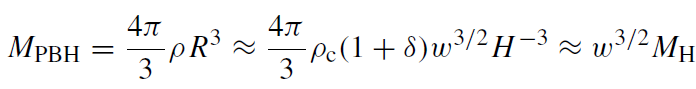 (1.5)

where M_H ≡ (4π/3)ρcH⁻³ denotes the mass inside the horizon. Since M_PBH is of the order of this horizon mass, a collapsing region will form a black hole practically immediately after horizon entry. Using the relation M_H = t/G, valid for a radiation-dominated Universe, one gets from (1.5) the quantitative estimate

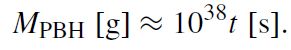 (1.6)

This means that one can create Planck-mass black holes at the Planck time, and PBHs with M_PBH ≈ 5 × 10¹⁴ g at t ≈ 5 × 10⁻²⁴ s. The latter value is important since, black holes with masses smaller than M_PBH ≈ 5 × 10¹⁴ g have by now evaporated due to Hawking radiation. PBHs with bigger mass are still present today. At t ≈ 10⁻⁵ s, one can create a solar mass black hole and at t ≈ 10 s (the time of nucleosynthesis) one could form a PBH with the mass of the Galactic black hole. The initial mass can increase by means of accretion, but it turns out that this is negligible under most circumstances (Carr 1985).

In the presence of an inflationary phase in the early Universe, all PBHs produced before the end of inflation are diluted away. This gives the bound

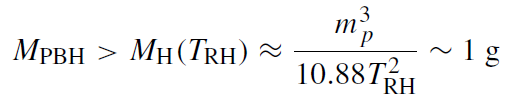 (1.7)

if for the reheating temperature T_RH a value of 10¹⁶ GeV is chosen.

According to the numerical calculations by Niemeyer and Jedamzik (1999), there exists a whole spectrum of initial masses,

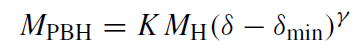 (1.8)

a relation that is reminiscent of the theory of critical phenomena. This may change some of the quantitative conclusions.

To calculate the production rate of PBHs, one needs an initial spectrum of fluctuations. This is usually taken to be of a Gaussian form, as predicted by most inflationary models (cf Liddle and Lyth 2000). Therefore, there always exists a non-vanishing probability that the density contrast is high enough to form a black hole, even if the maximum of the Gaussian corresponds to a small value. One can then calculate the mass ratio (compared to the total mass) of regions which will develop into PBHs with mass M_PBH > M, see, e.g., Bringmann et al (2001) for details. This mass ratio, given by

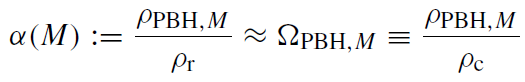 (1.9)

where ρ_r is the radiation density, is then compared with observation. This, in turn, gives a constraint on the theoretically calculated initial spectrum. Table 1.1 presents various observational constraints on α (see Green and Liddle 1997). The corresponding maximal value for each α is shown for the various constraints in figure 1.1.

Constraints arise either from Hawking radiation or from the gravitational contribution of PBHs to the present Universe (last entry). PBHs with initial mass of about 5 × 10¹⁴ g evaporate ‘today’. (They release about 10³⁰ erg in the last second.) From observations of the γ -ray background one can find the constraint given in table 1.1 which corresponds to an upper limit of about 10⁴ PBHs per cubic parsec or Ω_PBH,0 < 10⁻⁸. One can also try to observe directly the final evaporation event of a single PBH. This gives an upper limit of about 4.4 × 10⁵ events per cubic parsec per year.

Given these observational constraints, one can then calculate the ensuing constraints on the primordial spectrum. The gravitational constraint Ω_PBH,0 < 1 gives surprisingly strong restrictions (cf Bringmann et al 2001). For a scale free spectrum of the form ∝ kⁿ, as is usually discussed for inflationary models,

Table 1.1. Constraints on the mas fraction α(M) := ρ_PBH,M/ρ_r ≈ Ω_PBH,M of primordial black holes at their time of formation (Green and Liddle 1997).

Figure 1.2. Strongest constraints on the initial PBH mass fraction. The numbers correspond to the various entries in table 1.1.

one finds restrictions on n that are comparable to the limits obtained by large scale observations (the anisotropy spectrum of the cosmic microwave background radiation). Since these restrictions come from observational constraints referring to much smaller scales, they constitute an important complementary test.

The question as to whether PBHs really exist in nature has thus not yet been settled. Their presence would be of an importance that could hardly be overestimated. They would give the unique opportunity to study the quantum effects of black holes and could yield the crucial key for the construction of a final theory of quantum gravity.