Another way of describing the molecular collisions is to talk not about the time between collisions, but about how far the particle moves between collisions. If we say that the average time between collisions is τ, and that the molecules have a mean velocity v, we can expect that the average distance between collisions, which we shall call l, is just the product of τ and v. This distance between collisions is usually called the mean free path:

Mean free path l=τv.                                        (43.9)

In this chapter we shall be a little careless about what kind of average we mean in any particular case. The various possible averages—the mean, the root-mean-square, etc.—are all nearly equal and differ by factors which are near to one. Since a detailed analysis is required to obtain the correct numerical factors anyway, we need not worry about which average is required at any particular point. We may also warn the reader that the algebraic symbols we are using for some of the physical quantities (e.g., l for the mean free path) do not follow a generally accepted convention, mainly because there is no general agreement.

Just as the chance that a molecule will have a collision in a short time dt is equal to dt/τ, the chance that it will have a collision in going a distance dx is dx/l. Following the same line of argument used above, the reader can show that the probability that a molecule will go at least the distance x before having its next collision is e^−x/l.

The average distance a molecule goes before colliding with another molecule—the mean free path l—will depend on how many molecules there are around and on the “size” of the molecules, i.e., how big a target they represent. The effective “size” of a target in a collision we usually describe by a “collision cross section,” the same idea that is used in nuclear physics, or in light-scattering problems.

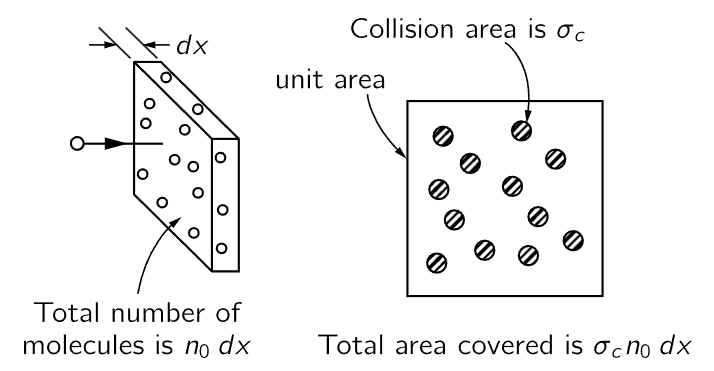
Fig. 43–1. Collision cross section.

Consider a moving particle which travels a distance dx through a gas which has n₀ scatterers (molecules) per unit volume (Fig. 43–1). If we look at each unit of area perpendicular to the direction of motion of our selected particle, we will find there n₀dx molecules. If each one presents an effective collision area or, as it is usually called, “collision cross section,” σ_c, then the total area covered by the scatterers is σ_cn₀dx.

By “collision cross section” we mean the area within which the center of our particle must be located if it is to collide with a particular molecule. If molecules were little spheres (a classical picture) we would expect that σ_c=π(r₁+r₂)², where r₁ and r₂ are the radii of the two colliding objects. The chance that our particle will have a collision is the ratio of the area covered by scattering molecules to the total area, which we have taken to be one. So the probability of a collision in going a distance dx is just σ_cn₀dx:

Chance of a collision in dx = σ_cn₀dx.                                  (43.10)

We have seen above that the chance of a collision in dx can also be written in terms of the mean free path l as dx/l. Comparing this with (43.10), we can relate the mean free path to the collision cross section:

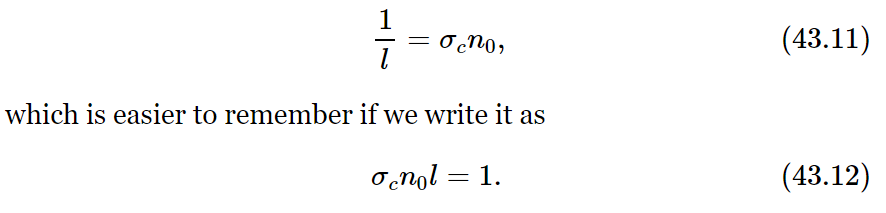
This formula can be thought of as saying that there should be one collision, on the average, when the particle goes through a distance l in which the scattering molecules could just cover the total area. In a cylindrical volume of length l and a base of unit area, there are n₀l scatterers; if each one has an area σ_c the total area covered is n₀lσ_c, which is just one unit of area. The whole area is not covered, of course, because some molecules are partly hidden behind others. That is why some molecules go farther than l before having a collision. It is only on the average that the molecules have a collision by the time they go the distance l. From measurements of the mean free path l we can determine the scattering cross section σ_c, and compare the result with calculations based on a detailed theory of atomic structure. But that is a different subject! So we return to the problem of nonequilibrium states.