تاريخ الرياضيات
الاعداد و نظريتها
تاريخ التحليل
تار يخ الجبر
الهندسة و التبلوجي
الرياضيات في الحضارات المختلفة
العربية
اليونانية
البابلية
الصينية
المايا
المصرية
الهندية
الرياضيات المتقطعة
المنطق
اسس الرياضيات
فلسفة الرياضيات
مواضيع عامة في المنطق
الجبر
الجبر الخطي
الجبر المجرد
الجبر البولياني
مواضيع عامة في الجبر
الضبابية
نظرية المجموعات
نظرية الزمر
نظرية الحلقات والحقول
نظرية الاعداد
نظرية الفئات
حساب المتجهات
المتتاليات-المتسلسلات
المصفوفات و نظريتها
المثلثات
الهندسة
الهندسة المستوية
الهندسة غير المستوية
مواضيع عامة في الهندسة
التفاضل و التكامل
المعادلات التفاضلية و التكاملية
معادلات تفاضلية
معادلات تكاملية
مواضيع عامة في المعادلات
التحليل
التحليل العددي
التحليل العقدي
التحليل الدالي
مواضيع عامة في التحليل
التحليل الحقيقي
التبلوجيا
نظرية الالعاب
الاحتمالات و الاحصاء
نظرية التحكم
بحوث العمليات
نظرية الكم
الشفرات
الرياضيات التطبيقية
نظريات ومبرهنات
علماء الرياضيات
500AD
500-1499
1000to1499
1500to1599
1600to1649
1650to1699
1700to1749
1750to1779
1780to1799
1800to1819
1820to1829
1830to1839
1840to1849
1850to1859
1860to1864
1865to1869
1870to1874
1875to1879
1880to1884
1885to1889
1890to1894
1895to1899
1900to1904
1905to1909
1910to1914
1915to1919
1920to1924
1925to1929
1930to1939
1940to the present
علماء الرياضيات
الرياضيات في العلوم الاخرى
بحوث و اطاريح جامعية
هل تعلم
طرائق التدريس
الرياضيات العامة
نظرية البيان
Jacobsthal Number
المؤلف:
Bergum, G. E.; Bennett, L.; Horadam, A. F.; and Moore, S. D
المصدر:
"Jacobsthal Polynomials and a Conjecture Concerning Fibonacci-Like Matrices." Fib. Quart. 23
الجزء والصفحة:
...
6-12-2020
790
+
-
20
Jacobsthal Number
The Jacobsthal numbers are the numbers obtained by the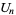 
s in the Lucas sequence with 
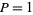and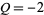 
, corresponding to 
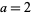and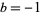 
. They and the Jacobsthal-Lucas numbers (the 
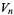s) satisfy the recurrence relation
 |
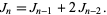(1) |
The Jacobsthal numbers satisfy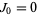 
and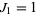 
and are 0, 1, 1, 3, 5, 11, 21, 43, 85, 171, 341, ... (OEIS A001045). The Jacobsthal-Lucas numbers satisfy 
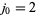and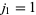 
and are 2, 1, 5, 7, 17, 31, 65, 127, 257, 511, 1025, ... (OEIS A014551). The properties of these numbers are summarized in Horadam (1996).
Microcontrollers (and other computers) use conditional instructions to change the flow of execution of a program. In addition to branch instructions, some microcontrollers use skip instructions which conditionally bypass the next instruction. This winds up being useful for one case out of the four possibilities on 2 bits, 3 cases on 3 bits, 5 cases on 4 bits, 11 on 5 bits, 21 on 6 bits, 43 on 7 bits, 85 on 8 bits, ..., which are exactly the Jacobsthal numbers (Hirst 2006).
The Jacobsthal and Jacobsthal-Lucas numbers are given by the closed form expressions
 |
 |
 |
(2) |
 |
 |
 |
(3) |
where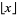 
is the floor function and 
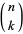is a binomial coefficient. The Binet forms are
 |
 |
 |
(4) |
 |
 |
![1/3[2^n-(-1)^n]](https://mathworld.wolfram.com/images/equations/JacobsthalNumber/Inline24.gif) |
(5) |
 |
 |
 |
(6) |
 |
 |
 |
(7) |
Amazingly, when interpreted in binary, the Jacobsthal numbers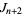 
give the 
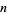th iteration of applying the rule 28 cellular automaton to initial conditions consisting of a single black cell (E. W. Weisstein, Apr. 12, 2006).
The generating functions are
 |
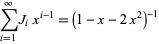(8) |
 |
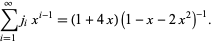(9) |
The Simson formulas are
 |
 |
 |
(10) |
 |
 |
 |
(11) |
Summation formulas include
 |
 |
 |
(12) |
 |
 |
 |
(13) |
Interrelationships are
 |
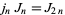(14) |
 |
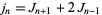(15) |
 |
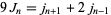(16) |
 |
(17) |
 |
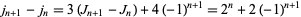(18) |
 |
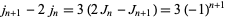(19) |
 |
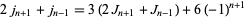(20) |
 |
 |
 |
(21) |
 |
 |
 |
(22) |
 |
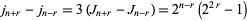(23) |
 |
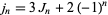(24) |
 |
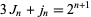(25) |
 |
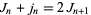(26) |
 |
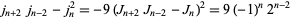(27) |
 |
(28) |
 |
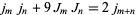(29) |
 |
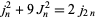(30) |
 |
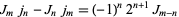(31) |
 |
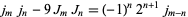(32) |
 |
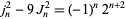(33) |
(Horadam 1996).
REFERENCES:
Bergum, G. E.; Bennett, L.; Horadam, A. F.; and Moore, S. D. "Jacobsthal Polynomials and a Conjecture Concerning Fibonacci-Like Matrices." Fib. Quart. 23, 240-248, 1985.
Hirst, C. "Hopscotch--Multiple Bit Testing." May 15, 2006. https://www.avrfreaks.net/index.php?module=FreaksAcademy&func=viewItem&item_id=229&item_type=project.
Horadam, A. F. "Jacobsthal and Pell Curves." Fib. Quart. 26, 79-83, 1988.
Horadam, A. F. "Jacobsthal Representation Numbers." Fib. Quart. 34, 40-54, 1996.
Sloane, N. J. A. Sequences A001045/M2482 and A014551 in "The On-Line Encyclopedia of Integer Sequences."
Hoggatt and Bicknell, in ÒConvolution Triangles,Ó FQ 10 (1972), 599-608),
الاكثر قراءة في نظرية الاعداد
اخر الاخبار
اخبار العتبة العباسية المقدسة
الآخبار الصحية
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
