The set {+infty,-infty}" src="https://mathworld.wolfram.com/images/equations/AffinelyExtendedRealNumbers/Inline1.gif" style="height:15px; width:90px" /> obtained by adjoining two improper elements to the set 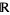 of real numbers is normally called the set of (affinely) extended real numbers. Although the notation for this set is not completely standardized,  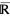is commonly used. The set may also be written in interval notation as . With an appropriate topology,  is the two-point compactification (or affine closure) of 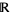. The improper elements, the affine infinities  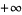and 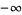, correspond to ideal points of the number line. Note that these improper elements are not real numbers, and that this system of extended real numbers is not a field.

Instead of writing 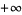, many authors write simply 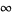. However, the compound symbol  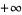will be used here to represent the positive improper element of 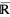, allowing the individual symbol  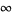to be used unambiguously to represent the unsigned improper element of 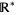, the one-point compactification (or projective closure) of .

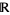A very important property of 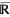, which  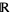lacks, is that every subset  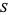of 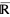 has an infimum (greatest lower bound) and a supremum (least upper bound). In particular,  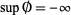and, if  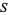is unbounded above, then 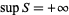. Similarly,  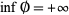and, if  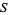is unbounded below, then .

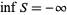Order relations can be extended from 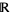 to 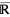, and arithmetic operations can be partially extended. For ,

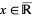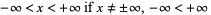	(1)
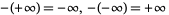	(2)
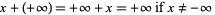	(3)
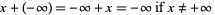	(4)
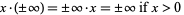	(5)
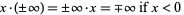	(6)
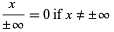	(7)
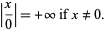	(8)

However, the expressions 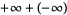, , 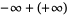and 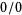 are undefined.

The above statements which define results of arithmetic operations on 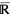 may be considered as abbreviations of statements about determinate limit forms. For example,  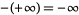may be considered as an abbreviation for "If  increases without bound, then  decreases without bound." Most descriptions of 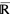 also make a statement concerning the products of the improper elements and 0, but there is no consensus as to what that statement should be. Some authors (e.g., Kolmogorov 1995, p. 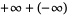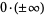193) state that, like  and ,  and 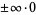 should be undefined, presumably because of the indeterminate status of the corresponding limit forms. Other authors (such as McShane 1983, p. 2) accept , at least as a convention which is useful in certain contexts.

Many results for other operations and functions can be obtained by considering determinate limit forms. For example, a partial extension of the function 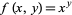 can be obtained for  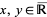as

		{0 if y<0; +infty if y>0" src="https://mathworld.wolfram.com/images/equations/AffinelyExtendedRealNumbers/Inline54.gif" style="height:41px; width:84px" />	(9)
		{0 if 0<x<1; +infty if x>1" src="https://mathworld.wolfram.com/images/equations/AffinelyExtendedRealNumbers/Inline57.gif" style="height:41px; width:106px" />	(10)
		{+infty if 0<x<1; 0 if x>1." src="https://mathworld.wolfram.com/images/equations/AffinelyExtendedRealNumbers/Inline60.gif" style="height:41px; width:106px" />	(11)

The functions 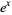 and  can be fully extended to 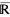, with

			(12)
			(13)
			(14)
			(15)

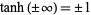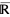Some other important functions (e.g.,  and ) can be extended to , while others (e.g., 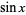, 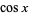) cannot. Evaluations of expressions involving  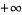and 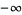, derived by considering determinate limit forms, are routinely used by computer algebra languages such as the Wolfram Language when performing simplifications.

Floating-point arithmetic, with its two signed infinities, is intended to approximate arithmetic on  (Goldberg 1991, pp. 21-22).

REFERENCES:

Goldberg, D. "What Every Computer Scientist Should Know About Floating-Point Arithmetic." ACM Comput. Surv. 23, 5-48, March 1991. https://docs.sun.com/source/806-3568/ncg_goldberg.html.

Kolmogorov, N. A. "Infinity." Encyclopaedia of Mathematics: An Updated and Annotated Translation of the Soviet "Mathematical Encyclopaedia," 2nd ed., Vol. 3. (Managing Ed. M. Hazewinkel). Dordrecht, Netherlands: Reidel, 1995.

McShane, E. J. Unified Integration. Orlando, FL: Academic Press, p. 2, 1983.

اخر الاخبار

اخبار العتبة العباسية المقدسة

العتبة العباسية المقدسة تواصل تنظيم مجالس العزاء الصباحية بذكرى زيارة عاشوراء

مواكب العزاء تستذكر في التاسع من شهر المحرم شهادة عبدالله الرضيع بن الحسين (عليهما السلام)

العتبة العباسية المقدسة تستذكر تضحيات القاسم بن الحسن (عليهما السلام)

شعبة الحرم الشريف تواصل تنفيذ خطتها الخاصة بشهر المحرّم داخل مرقد أبي الفضل العباس (عليه السلام)

المزيد

الآخبار الصحية

لهذا السبب.. إغلاق 25 مركزًا لعلاج الإدمان في مصر ؟

خلافا للاعتقاد الشائع.. علماء يكشفون أسرار بذور البطيخ ؟!

هذا ما يحدث لجسمك عند تناولك الطماطم بانتظام !

تعرف على أطعمة تسرّع "تعافي الجسم" من حروق الشمس

المزيد

الآخبار التكنلوجية

الثلوج تغطي الصحراء الأكثر جفافا في العالم!

بتلسكوب "ألما".. التقاط صورا غير مسبوقة لبدايات الكون !

هل هاتفك مراقب؟.. 5 علامات خطيرة تكشف التجسس عليك

دبي تطلق أول رحلة تجريبية للتاكسي الجوي

المزيد