The Kepert model

    For many years after the classic work of Werner which laid the foundations for the correct formulation of d-block metal complexes , it was assumed that a metal in a given oxidation state would have a fixed coordination number and geometry. In the light of the success (albeit not universal success) of VSEPR theory in predicting the shapes of molecular species of the p-block elements, we might reasonably expect the structures of the complex ions [V(H₂O(₆]^3+‏ (d²),  Mn)H₂O(₆]³⁺‏ (d⁴), [Co(H₂O)₆]³⁺‏ (d⁶), [Ni(H₂O)₆]²⁺‏ (d⁸) and [Zn(H₂O)₆]^2‏+ (d¹⁰) to vary as the electronic configuration of the metal ion changes. However, each of these species has an octahedral arrangement of ligands (1.1). Thus, it is clear that VSEPR theory is not applicable to d-block metal complexes.

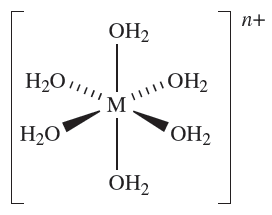
(1.1)

   We turn instead to the Kepert model, in which the metal lies at the centre of a sphere and the ligands are free to move over the surface of the sphere. The ligands are considered to repel one another in a similar manner to the point charges in the VSEPR model; however, unlike the VSEPR model, that of Kepert ignores non-bonding electrons. Thus, the coordination geometry of a d-block species is considered by Kepert to be independent of the ground state electronic configuration of the metal centre, and so ions of type [ML_n]^m+‏ and [ML_n]^m- have the same coordination geometry.

   The Kepert model rationalizes the shapes of d-block metal complexes [ML_n], [ML_n]^m‏⁺ or [ML_n]^m- by considering the repulsions between the groups L. Lone pairs of electrons are ignored. For coordination numbers between 2 and 6, the following arrangements of donor atoms are predicted:

2 linear

3 trigonal planar

4 tetrahedral

5 trigonal bipyramidal or square-based pyramidal

6 octahedral

   Table 1.4 lists coordination environments associated with coordination numbers between 2 and 9; not all are predictable using the Kepert model. For example, after considering the repulsions between the cyano ligands in [Cu(CN(₃]^2- , the coordination sphere would be predicted to be trigonal planar (1.2). Indeed, this is what is found experimentally. The other option in Table 1.4 is trigonal pyramidal, but this does not minimize interligand repulsions. One of the most important classes of structure for which the Kepert model does not predict the correct answer is that of the square planar complex, and here electronic effects are usually the controlling factor.. Another factor that may lead to a breakdown of the Kepert model is the inherent constraint of a ligand.

 For example:

• the four nitrogen donor atoms of a porphyrin ligand () are confined to a square planar array;
• tripodal ligands such as 1.3 have limited flexibility which means that the donor atoms are not necessarily free to adopt the positions predicted by Kepert;
• macrocyclic ligands are less flexible than open chain ligands.

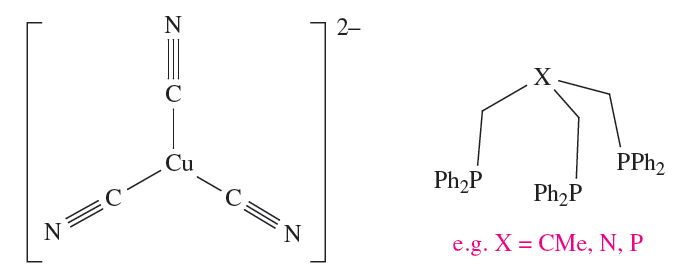
(1.2)                                                         (1.3)

    A tripodal ligand (e.g. 1.3) is one containing three arms, each with a donor atom, which radiate from a central atom or group; this central point may itself be a donor atom.  In the remaining part of this section, we give a systematic outline of the occurrence of different coordination numbers and geometries in solid state d-block metal complexes.   A general word of caution: molecular formulae can be misleading in terms of coordination number. For example in CdI₂, each Cd centre is octahedrally sited, and molecular halides or pseudo-halides (e.g. [CN]^-) may contain M^_X^_M bridges and exist as oligomers, e.g. α-PdCl₂ is polymeric (1.4).

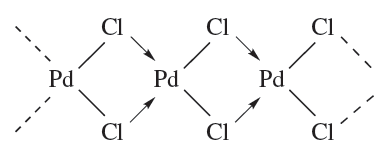
(1.4)

   A further ambiguity arises when the bonding mode of a ligand can be described in more than one way.  This often happens in organometallic chemistry, for example with cyclopentadienyl ligands, there is still the question of whether to consider, for example, an [η⁵-C₅H₅]^- ligand as occupying one or five sites in the coordination sphere of a metal atom: thus, the coordination number of the Ti(IV) centre in [(η⁵-C₅H₅)₂TiCl₂] may be represented as either 1.5a or 1.5b.

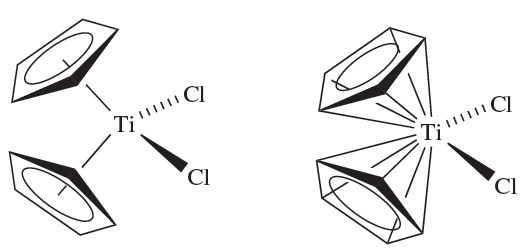
(1.5a)                                                    (1.5b)

مواضيع ذات صلة

ايجاد الصيغة التركيبية للمعقدات

معقدات الخارصين

معقدات النحاس

كيمياء ومعقدات بعض عناصر السلسلة الانتقالية الاولى

تعريف المركبات المعقدة

Crystal Field Stabilization Energies

Colors of Transition-Metal Complexes

The Nature of the Ligands

Principal Quantum Number of the Metal

Charge on the Metal Ion

Factors That Affect the Magnitude of Δo

Electronic Structures of Metal Complexes