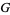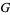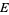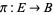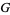A principal bundle is a special case of a fiber bundle where the fiber is a group . More specifically,  is usually a Lie group. A principal bundle is a total space  along with a surjective map  to a base manifold . Any fiber  is a space isomorphic to . More specifically,  acts freely without fixed point on the fibers, and this makes a fiber into a homogeneous space. For example, in the case of a circle bundle (i.e., when {e^(it)}" src="https://mathworld.wolfram.com/images/equations/PrincipalBundle/Inline9.gif" style="height:21px; width:83px" />), the fibers are circles, which can be rotated, although no point in particular corresponds to the identity. Near every point, the fibers can be given the group structure of 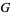 in the fibers over a neighborhood  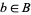by choosing an element in each fiber to be the identity element. However, the fibers cannot be given a group structure globally, except in the case of a trivial bundle.

An important principal bundle is the frame bundle on a Riemannian manifold. This bundle reflects the different ways to give an orthonormal basis for tangent vectors.

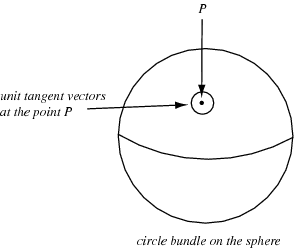
Consider all of the unit tangent vectors on the sphere. This is a principal bundle 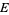 on the sphere with fiber the circle . Every tangent vector projects to its base point in 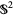, giving the map 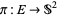. Over every point in , there is a circle of unit tangent vectors. No particular vector is singled out as the identity, but the group  of rotations acts freely without fixed point on the fibers.

In a similar way, any fiber bundle corresponds to a principal bundle where the group (of the principal bundle) is the group of isomorphisms of the fiber (of the fiber bundle). Given a principal bundle 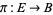 and an action of  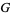on a space 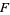, which could be a group representation, this can be reversed to give an associated fiber bundle.

A trivialization of a principal bundle, an open set 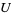 in 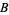 such that the bundle over 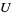, , 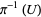is expressed as 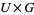, has the property that the group  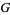acts on the left. That is,  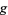acts on  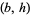by 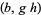. Tracing through these definitions, it is not hard to see that the transition functions take values in 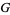, acting on the fibers by right multiplication. This way the action of  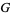on a fiber is independent of coordinate chart.