

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Normal Distribution Function
المؤلف:
Abramowitz, M. and Stegun, I. A.
المصدر:
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover,
الجزء والصفحة:
...
12-4-2021
3648
Normal Distribution Function
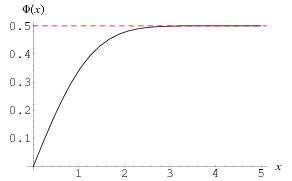

A normalized form of the cumulative normal distribution function giving the probability that a variate assumes a value in the range ![[0,x]](https://mathworld.wolfram.com/images/equations/NormalDistributionFunction/Inline1.gif) ,
,
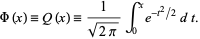 |
(1) |
It is related to the probability integral
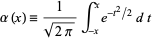 |
(2) |
by
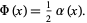 |
(3) |
Let 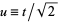 so
so 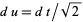 . Then
. Then
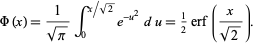 |
(4) |
Here, erf is a function sometimes called the error function. The probability that a normal variate assumes a value in the range ![[x_1,x_2]](https://mathworld.wolfram.com/images/equations/NormalDistributionFunction/Inline4.gif) is therefore given by
is therefore given by
![Phi(x_1,x_2)=1/2[erf((x_2)/(sqrt(2)))-erf((x_1)/(sqrt(2)))].](https://mathworld.wolfram.com/images/equations/NormalDistributionFunction/NumberedEquation5.gif) |
(5) |
Neither  nor erf can be expressed in terms of finite additions, subtractions, multiplications, and root extractions, and so must be either computed numerically or otherwise approximated.
nor erf can be expressed in terms of finite additions, subtractions, multiplications, and root extractions, and so must be either computed numerically or otherwise approximated.
Note that a function different from 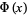 is sometimes defined as "the" normal distribution function
is sometimes defined as "the" normal distribution function
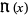 |
 |
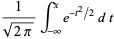 |
(6) |
 |
 |
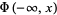 |
(7) |
 |
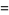 |
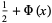 |
(8) |
 |
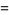 |
![1/2[1+erf(x/(sqrt(2)))]](https://mathworld.wolfram.com/images/equations/NormalDistributionFunction/Inline18.gif) |
(9) |
(Feller 1968; Beyer 1987, p. 551), although this function is less widely encountered than the usual  . The notation
. The notation 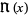 is due to Feller (1971).
is due to Feller (1971).
The value of 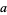 for which
for which 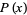 falls within the interval
falls within the interval ![[-a,a]](https://mathworld.wolfram.com/images/equations/NormalDistributionFunction/Inline23.gif) with a given probability
with a given probability 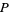 is a related quantity called the confidence interval.
is a related quantity called the confidence interval.
For small values 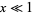 , a good approximation to
, a good approximation to 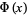 is obtained from the Maclaurin series for erf,
is obtained from the Maclaurin series for erf,
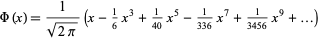 |
(10) |
(OEIS A014481). For large values  , a good approximation is obtained from the asymptotic series for erf,
, a good approximation is obtained from the asymptotic series for erf,
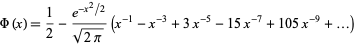 |
(11) |
(OEIS A001147).
The value of 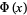 for intermediate
for intermediate  can be computed using the continued fraction identity
can be computed using the continued fraction identity
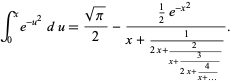 |
(12) |
A simple approximation of 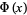 which is good to two decimal places is given by
which is good to two decimal places is given by
|
(13) |
Abramowitz and Stegun (1972) and Johnson et al. (1994) give other functional approximations. An approximation due to Bagby (1995) is
|
(14) |
The plots below show the differences between 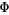 and the two approximations.
and the two approximations.
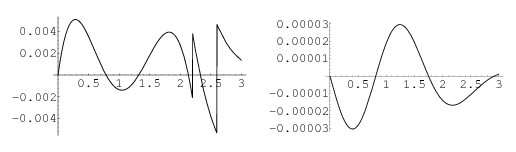
The value of 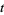 giving
giving 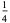 is known as the probable error of a normally distributed variate.
is known as the probable error of a normally distributed variate.
REFERENCES:
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, pp. 931-933, 1972.
Bagby, R. J. "Calculating Normal Probabilities." Amer. Math. Monthly 102, 46-49, 1995.
Beyer, W. H. (Ed.). CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, 1987.
Bryc, W. "A Uniform Approximation to the Right Normal Tail Integral." Math. Comput. 127, 365-374, 2002.
Feller, W. An Introduction to Probability Theory and Its Applications, Vol. 1, 3rd ed. New York: Wiley, 1968.
Feller, W. An Introduction to Probability Theory and Its Applications, Vol. 2, 3rd ed. New York: Wiley, p. 45, 1971.
Hastings, C. Approximations for Digital Computers. Princeton, NJ: Princeton University Press, 1955.
Johnson, N.; Kotz, S.; and Balakrishnan, N. Continuous Univariate Distributions, Vol. 1, 2nd ed. Boston, MA: Houghton Mifflin, 1994.
Patel, J. K. and Read, C. B. Handbook of the Normal Distribution. New York: Dekker, 1982.
Sloane, N. J. A. Sequences A001147/M3002 and A014481 in "The On-Line Encyclopedia of Integer Sequences."
Whittaker, E. T. and Robinson, G. "Normal Frequency Distribution." Ch. 8 in The Calculus of Observations: A Treatise on Numerical Mathematics, 4th ed. New York: Dover, pp. 164-208, 1967.
 الاكثر قراءة في الاحتمالات و الاحصاء
الاكثر قراءة في الاحتمالات و الاحصاء
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















