The irrational constant

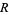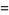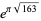			(1)
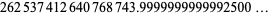			(2)

(OEIS A060295), which is very close to an integer. Numbers such as the Ramanujan constant can be found using the theory of modular functions. In fact, the nine Heegner numbers (which include 163) share a deep number theoretic property related to some amazing properties of the j-function that leads to this sort of near-identity.

Although Ramanujan (1913-1914) gave few rather spectacular examples of almost integers (such 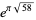), he did not actually mention the particular near-identity given above. In fact, Hermite (1859) observed this property of 163 long before Ramanujan's work. The name "Ramanujan's constant" was coined by Simon Plouffe and derives from an April Fool's joke played by Martin Gardner (Apr. 1975) on the readers of Scientific American. In his column, Gardner claimed that  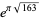was exactly an integer, and that Ramanujan had conjectured this in his 1914 paper. Gardner admitted his hoax a few months later (Gardner, July 1975).

The Ramanujan constant can be approximated to 14 digits by

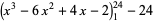			(3)
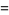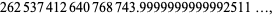			(4)

(OEIS A102912; Piezas), where 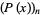 is a polynomial root.

REFERENCES:

Ball, W. W. R. and Coxeter, H. S. M. Mathematical Recreations and Essays, 13th ed. New York: Dover, p. 387, 1987.

Castellanos, D. "The Ubiquitous Pi. Part I." Math. Mag. 61, 67-98, 1988.

Gardner, M. "Mathematical Games: Six Sensational Discoveries that Somehow or Another have Escaped Public Attention." Sci. Amer. 232, 127-131, Apr. 1975.

Gardner, M. "Mathematical Games: On Tessellating the Plane with Convex Polygons." Sci. Amer. 232, 112-117, Jul. 1975.

Good, I. J. "What is the Most Amazing Approximate Integer in the Universe?" Pi Mu Epsilon J. 5, 314-315, 1972.

Hermite, C. "Sur la théorie des équations modulaires." Comptes Rendus Acad. Sci. Paris 49, 16-24, 110-118, and 141-144, 1859. Reprinted in Oeuvres complètes, Tome II. Paris: Hermann, p. 61, 1912.

Michon, G. P. "Final Answers: Numerical Constants." http://home.att.net/~numericana/answer/constants.htm#ramanju.

Piezas, T. III "Ramanujan's Constant And Its Cousins." http://www.geocities.com/titus_piezas/Ramanujan_a.htm.

Ramanujan, S. "Modular Equations and Approximations to 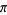." Quart. J. Pure Appl. Math. 45, 350-372, 1913-1914.

Sloane, N. J. A. Sequences A060295 and A102912 in "The On-Line Encyclopedia of Integer Sequences."

Wolfram, S. The Mathematica Book, 5th ed. Champaign, IL: Wolfram Media, p. 33, 2003.

Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, p. 913, 2002.