

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Base
المؤلف:
Allouche, J.-P. and Shallit, J.
المصدر:
"Representations in Negative Bases." §3.7 in Automatic Sequences: Theory, Applications, Generalizations. Cambridge, England: Cambridge University Press
الجزء والصفحة:
...
22-11-2019
2236
Base
The word "base" in mathematics is used to refer to a particular mathematical object that is used as a building block. The most common uses are the related concepts of the number system whose digits are used to represent numbers and the number system in which logarithms are defined. It can also be used to refer to the bottom edge or surface of a geometric figure.
A real number  can be represented using any integer number
can be represented using any integer number  as a base (sometimes also called a radix or scale). The choice of a base yields to a representation of numbers known as a number system. In base
as a base (sometimes also called a radix or scale). The choice of a base yields to a representation of numbers known as a number system. In base 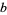 , the digits 0, 1, ...,
, the digits 0, 1, ...,  are used (where, by convention, for bases larger than 10, the symbols A, B, C, ... are generally used as symbols representing the decimal numbers 10, 11, 12, ...).
are used (where, by convention, for bases larger than 10, the symbols A, B, C, ... are generally used as symbols representing the decimal numbers 10, 11, 12, ...).
The digits of a number  in base
in base 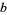 (for integer
(for integer 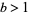 ) can be obtained in the Wolfram Language using IntegerDigits[x, b].
) can be obtained in the Wolfram Language using IntegerDigits[x, b].
Let the base  representation of a number
representation of a number  be written
be written
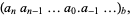 |
(1) |
(e.g., 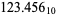 ). Then, for example, the number 10 is written in various bases as
). Then, for example, the number 10 is written in various bases as
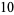 |
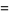 |
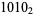 |
(2) |
 |
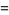 |
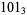 |
(3) |
 |
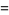 |
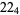 |
(4) |
 |
 |
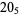 |
(5) |
 |
 |
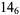 |
(6) |
 |
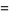 |
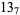 |
(7) |
 |
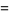 |
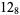 |
(8) |
 |
 |
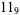 |
(9) |
 |
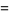 |
 |
(10) |
 |
 |
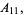 |
(11) |
since, for example,
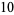 |
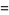 |
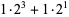 |
(12) |
 |
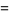 |
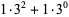 |
(13) |
 |
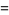 |
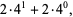 |
(14) |
and so on.
Common bases are given special names based on the value of  , as summarized in the following table. The most common bases are binary and hexadecimal (used by computers) and decimal (used by people).
, as summarized in the following table. The most common bases are binary and hexadecimal (used by computers) and decimal (used by people).
| base | number system |
| 2 | binary |
| 3 | ternary |
| 4 | quaternary |
| 5 | quinary |
| 6 | senary |
| 7 | septenary |
| 8 | octal |
| 9 | nonary |
| 10 | decimal |
| 11 | undenary |
| 12 | duodecimal |
| 16 | hexadecimal |
| 20 | vigesimal |
| 60 | sexagesimal |
The index of the leading digit needed to represent the number is
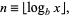 |
(15) |
where  is the floor function. Now, recursively compute the successive digits
is the floor function. Now, recursively compute the successive digits
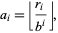 |
(16) |
where 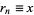 and
and
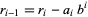 |
(17) |
for 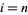 ,
,  , ..., 1, 0, .... This gives the base
, ..., 1, 0, .... This gives the base 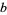 representation of
representation of  . Note that if
. Note that if  is an integer, then
is an integer, then 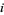 need only run through 0, and that if
need only run through 0, and that if  has a fractional part, then the expansion may or may not terminate. For example, the hexadecimal representation of 0.1 (which terminates in decimal notation) is the infinite expression
has a fractional part, then the expansion may or may not terminate. For example, the hexadecimal representation of 0.1 (which terminates in decimal notation) is the infinite expression 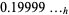 .
.
Some number systems use a mixture of bases for counting. Examples include the Mayan calendar and the old British monetary system (in which ha'pennies, pennies, threepence, sixpence, shillings, half crowns, pounds, and guineas corresponded to units of 1/2, 1, 3, 6, 12, 30, 240, and 252, respectively).
Bergman (1957/58) considered an irrational base, and Knuth (1998) considered transcendental bases. This leads to some rather unfamiliar results, such as equating 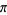 to 1 in "base
to 1 in "base 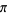 ,"
,"  . Even more unexpectedly, the representation of a given integer in an irrational base may be nonunique, for example
. Even more unexpectedly, the representation of a given integer in an irrational base may be nonunique, for example
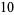 |
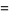 |
 |
(18) |
 |
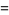 |
 |
(19) |
where 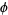 is the golden ratio.
is the golden ratio.
It is also possible to consider negative bases such as negabinary and negadecimal (e.g., Allouche and Shallit 2003). The digits in a negative base may be obtained with the Wolfram Language code
NegativeIntegerDigits[0, n_Integer?Negative] := {0}
NegativeIntegerDigits[i_, n_Integer?Negative] :=
Rest @ Reverse @ Mod[
NestWhileList[(# - Mod[#, -n])/n& ,
i, # != 0& ],
-n]
The base of a logarithm is a number 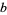 used to define the number system in which the logarithm is computed. In general, the logarithm of a number
used to define the number system in which the logarithm is computed. In general, the logarithm of a number  in base
in base 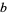 is written
is written  . The symbol
. The symbol 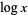 is an abbreviation regrettably used both for the common logarithm
is an abbreviation regrettably used both for the common logarithm 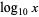 (by engineers and physicists and indicated on pocket calculators) and for the natural logarithm
(by engineers and physicists and indicated on pocket calculators) and for the natural logarithm 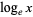 (by mathematicians).
(by mathematicians). 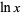 denotes the natural logarithm
denotes the natural logarithm 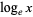 (as used by engineers and physicists and indicated on pocket calculators), and
(as used by engineers and physicists and indicated on pocket calculators), and 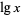 denotes
denotes  . In this work, the notations
. In this work, the notations 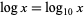 and
and 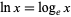 are used.
are used.
To convert between logarithms in different bases, the formula
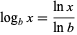 |
(20) |
can be used.
REFERENCES:
Allouche, J.-P. and Shallit, J. "Representations in Negative Bases." §3.7 in Automatic Sequences: Theory, Applications, Generalizations. Cambridge, England: Cambridge University Press, pp. 103-105, 2003.
Abramowitz, M. and Stegun, I. A. (Eds.). Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. New York: Dover, p. 28, 1972.
Bergman, G. "A Number System with an Irrational Base." Math. Mag. 31, 98-110, 1957/58.
Bogomolny, A. "Base Converter." http://www.cut-the-knot.org/binary.shtml.
Knuth, D. E. "Positional Number Systems." §4.1 in The Art of Computer Programming, Vol. 2: Seminumerical Algorithms, 3rd ed. Reading, MA: Addison-Wesley, pp. 195-213, 1998.
Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press, pp. 6-11, 1991.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















