

تاريخ الرياضيات

الاعداد و نظريتها

تاريخ التحليل

تار يخ الجبر

الهندسة و التبلوجي


الرياضيات في الحضارات المختلفة

العربية

اليونانية

البابلية

الصينية

المايا

المصرية

الهندية


الرياضيات المتقطعة

المنطق

اسس الرياضيات

فلسفة الرياضيات

مواضيع عامة في المنطق


الجبر

الجبر الخطي

الجبر المجرد

الجبر البولياني

مواضيع عامة في الجبر

الضبابية

نظرية المجموعات

نظرية الزمر

نظرية الحلقات والحقول

نظرية الاعداد

نظرية الفئات

حساب المتجهات

المتتاليات-المتسلسلات

المصفوفات و نظريتها

المثلثات


الهندسة

الهندسة المستوية

الهندسة غير المستوية

مواضيع عامة في الهندسة

التفاضل و التكامل


المعادلات التفاضلية و التكاملية

معادلات تفاضلية

معادلات تكاملية

مواضيع عامة في المعادلات


التحليل

التحليل العددي

التحليل العقدي

التحليل الدالي

مواضيع عامة في التحليل

التحليل الحقيقي

التبلوجيا

نظرية الالعاب

الاحتمالات و الاحصاء

نظرية التحكم

بحوث العمليات

نظرية الكم

الشفرات

الرياضيات التطبيقية

نظريات ومبرهنات


علماء الرياضيات

500AD

500-1499

1000to1499

1500to1599

1600to1649

1650to1699

1700to1749

1750to1779

1780to1799

1800to1819

1820to1829

1830to1839

1840to1849

1850to1859

1860to1864

1865to1869

1870to1874

1875to1879

1880to1884

1885to1889

1890to1894

1895to1899

1900to1904

1905to1909

1910to1914

1915to1919

1920to1924

1925to1929

1930to1939

1940to the present

علماء الرياضيات

الرياضيات في العلوم الاخرى

بحوث و اطاريح جامعية

هل تعلم

طرائق التدريس

الرياضيات العامة

نظرية البيان
Algebraic Number
المؤلف:
Conway, J. H. and Guy, R. K.
المصدر:
"Algebraic Numbers." In The Book of Numbers. New York: Springer-Verlag
الجزء والصفحة:
...
16-10-2019
1915
Algebraic Number
If 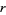 is a root of a nonzero polynomial equation
is a root of a nonzero polynomial equation
 |
(1) |
where the 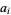 s are integers (or equivalently, rational numbers) and
s are integers (or equivalently, rational numbers) and  satisfies no similar equation of degree
satisfies no similar equation of degree 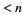 , then
, then 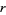 is said to be an algebraic number of degree
is said to be an algebraic number of degree  .
.
A number that is not algebraic is said to be transcendental. If 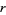 is an algebraic number and
is an algebraic number and 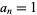 , then it is called an algebraic integer.
, then it is called an algebraic integer.
In general, algebraic numbers are complex, but they may also be real. An example of a complex algebraic number is 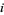 , and an example of a real algebraic number is
, and an example of a real algebraic number is 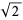 , both of which are of degree 2.
, both of which are of degree 2.
The set of algebraic numbers is denoted 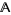 (Wolfram Language), or sometimes
(Wolfram Language), or sometimes 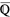 (Nesterenko 1999), and is implemented in the Wolfram Language as Algebraics.
(Nesterenko 1999), and is implemented in the Wolfram Language as Algebraics.
A number  can then be tested to see if it is algebraic in the Wolfram Language using the command Element[x, Algebraics]. Algebraic numbers are represented in the Wolfram Language as indexed polynomial roots by the symbol Root[f, n], where
can then be tested to see if it is algebraic in the Wolfram Language using the command Element[x, Algebraics]. Algebraic numbers are represented in the Wolfram Language as indexed polynomial roots by the symbol Root[f, n], where 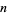 is a number from 1 to the degree of the polynomial (represented as a so-called "pure function")
is a number from 1 to the degree of the polynomial (represented as a so-called "pure function") 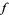 .
.
Examples of some significant algebraic numbers and their degrees are summarized in the following table.
| constant | degree |
Conway's constant 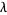 |
71 |
Delian constant  |
3 |
disk covering problem  |
8 |
| Freiman's constant | 2 |
golden ratio  |
2 |
golden ratio conjugate  |
2 |
Graham's biggest little hexagon area 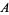 |
10 |
hard hexagon entropy constant 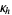 |
24 |
| heptanacci constant | 7 |
| hexanacci constant | 6 |
| i | 2 |
| Lieb's square ice constant | 2 |
logistic map 3-cycle onset 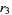 |
2 |
logistic map 4-cycle onset  |
2 |
logistic map 5-cycle onset  |
22 |
logistic map 6-cycle onset 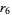 |
40 |
logistic map 7-cycle onset 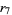 |
114 |
logistic map 8-cycle onset 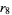 |
12 |
logistic map 16-cycle onset 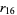 |
240 |
| pentanacci constant | 5 |
| plastic constant | 3 |
Pythagoras's constant 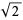 |
2 |
| silver constant | 3 |
| silver ratio | 2 |
| tetranacci constant | 4 |
| Theodorus's constant | 2 |
| tribonacci constant | 3 |
| twenty-vertex entropy constant | 2 |
| Wallis's constant | 3 |
If, instead of being integers, the  s in the above equation are algebraic numbers
s in the above equation are algebraic numbers 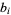 , then any root of
, then any root of
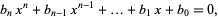 |
(2) |
is an algebraic number.
If 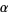 is an algebraic number of degree
is an algebraic number of degree 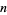 satisfying the polynomial equation
satisfying the polynomial equation
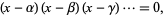 |
(3) |
then there are  other algebraic numbers
other algebraic numbers 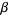 ,
, 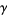 , ... called the conjugates of
, ... called the conjugates of 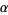 . Furthermore, if
. Furthermore, if 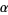 satisfies any other algebraic equation, then its conjugates also satisfy the same equation (Conway and Guy 1996).
satisfies any other algebraic equation, then its conjugates also satisfy the same equation (Conway and Guy 1996).
REFERENCES:
Conway, J. H. and Guy, R. K. "Algebraic Numbers." In The Book of Numbers. New York: Springer-Verlag, pp. 189-190, 1996.
Courant, R. and Robbins, H. "Algebraic and Transcendental Numbers." §2.6 in What Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed. Oxford, England: Oxford University Press, pp. 103-107, 1996.
Ferreirós, J. "The Emergence of Algebraic Number Theory." §3.3 in Labyrinth of Thought: A History of Set Theory and Its Role in Modern Mathematics. Basel, Switzerland: Birkhäuser, pp. 94-99, 1999.
Hancock, H. Foundations of the Theory of Algebraic Numbers, Vol. 1: Introduction to the General Theory. New York: Macmillan, 1931.
Hancock, H. Foundations of the Theory of Algebraic Numbers, Vol. 2: The General Theory. New York: Macmillan, 1932.
Koch, H. Number Theory: Algebraic Numbers and Functions. Providence, RI: Amer. Math. Soc., 2000.
Nagell, T. Introduction to Number Theory. New York: Wiley, p. 35, 1951.
Narkiewicz, W. Elementary and Analytic Number Theory of Algebraic Numbers. Warsaw: Polish Scientific Publishers, 1974.
Nesterenko, Yu. V. A Course on Algebraic Independence: Lectures at IHP 1999. Unpublished manuscript. 1999.
Wagon, S. "Algebraic Numbers." §10.5 in Mathematica in Action. New York: W. H. Freeman, pp. 347-353, 1991.
Wolfram, S. A New Kind of Science. Champaign, IL: Wolfram Media, p. 1168, 2002.
 الاكثر قراءة في نظرية الاعداد
الاكثر قراءة في نظرية الاعداد
 اخر الاخبار
اخر الاخبار
اخبار العتبة العباسية المقدسة

الآخبار الصحية















 "المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة
"المهمة".. إصدار قصصي يوثّق القصص الفائزة في مسابقة فتوى الدفاع المقدسة للقصة القصيرة (نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام)
(نوافذ).. إصدار أدبي يوثق القصص الفائزة في مسابقة الإمام العسكري (عليه السلام) قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)
قسم الشؤون الفكرية يصدر مجموعة قصصية بعنوان (قلوب بلا مأوى)


















